2
$\begingroup$

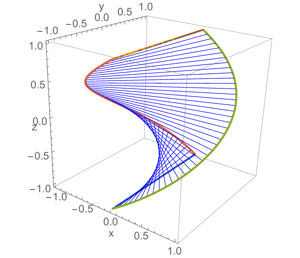
A surface is bounded by four lines parametrised as $(x,y,z)=$

$$ (0,u,- 1), (-1<u<1); \, (0,u,1), (-1<u<1); $$

$$(\cos v, \sin v, 2 v/ \pi), (- \pi/2 < v< \pi/2); \, (-\cos v, -\sin v, 2v /\pi), (-\pi/2,< v < \pi/2); \,$$ It is required to find parametrization for constant $K$ surfaces whose

  1. K= -1
  2. K= 0
  3. K= +1

The Dini surface does not meet requirement of a helix border for case 1. Neither Mean curvature H =0 helicoid of varying K satisfies this case 1.

Untwisted constant $ H$ CMC surfaces catenoid, cylinder, sphere of soap films form across two concentric circular tube edges of radius 1 rotated on x-axis.They have respectively their ODE connecting principal curvatures as:

$$ \kappa_1 + \kappa_ 2 = T,$$

where constant surface tension T can take $ -1, 0, +1 $ values.Their twisted surface parametrization is now sought, thanks for your help here.

EDIT 1:

As far as spanning between two helical lines is concerned, I have chosen circular toroidal upper,lower and flat points in the WolframAlpha link in place of Elliptic Integrals/functions as an approximation to the needed situation, K > 0, K = 0, K <0 regions of the torus can be seen.

EDIT2

A helical cuspidal boundary defined by David Brander as a pseudospherical front must have a spherical front' or gorge/groove etc. imho,that at is how one views positive and negative constant curvature surfaces..David Hilbert proved a cuspidal edge boundary and the conoidal point vertex for $K\equiv _1$, and for spherical case $K\equiv =+1$ someone else also proved the same...Am I right?

EDIT3:

Asked in SE Math for a perhaps easier formulation where the limit / boundary of quadrangle patch is removed defining an infinitely long curved strip:

EDIT 4:

If formulation would be more suitable please consider the case of semi-infinite surface formed by removing upper edges and retaining only the helices:

$$(\cos v, \sin v, 2 v/ \pi), (- \pi/2 < v< \pi/2); \, (-\cos v, -\sin v, 2v /\pi), (-\pi/2,< v < \pi/2).$$

Infinite Warped Strip

Const K surfaces between helices

Improve this question
$\endgroup$
6
  • 2
    $\begingroup$ What does 'it is required to find' mean in this case? Is this a homework problem? (If so, it is a very hard one.) In fact, I find it hard to believe that you will find a surface with this boundary that has $K\equiv1$. Certainly, if you do, it will not be smooth across the boundary because no surface with $K\equiv1$ can contain a straight line as a geodesic (for simple reasons). Similarly, I think you can rule out $K=0$ because you know that the surface will be ruled and it should be easy to show that no ruling can have lines that meet the helices in two points and sweep out a flat surface. $\endgroup$
    – Robert Bryant
    Commented May 11, 2016 at 13:19
  • $\begingroup$ Afraid am not able to agree in both situations.For K>0, imagine a tightly wound hollow tube spiral wound on a cylinder.It produces approximately two spirals of both signs of $K$.If you accept negative then you must also accept positive K. The cuspidaledge can be imagined isometrically deformed so the cuspidaledge edhe can be placed on a straight line in case K=0. $\endgroup$
    – Narasimham
    Commented May 11, 2016 at 16:15
  • 1
    $\begingroup$ I don't understand your comment: A surface in $\mathbb{R}^3$ with positive Gauss curvature (in particular, when $K\equiv1$) cannot contain any straight lines, for, if it did, then the tangent plane at a point on such a line would contain the line, but we know that when $K>0$, the surface is locally strictly convex, so it cannot contain any lines. $\endgroup$
    – Robert Bryant
    Commented May 11, 2016 at 17:33
  • 1
    $\begingroup$ I forgot to mention that we are not talking about smooth continuously differentiable case but the cuspidal edge of an elliptic type sphere,which has to be twisted physically like a rubber sheet and placed on a straight line and by means of isometry formulation with its deformation after the twist around this straight cuspidal line. $\endgroup$
    – Narasimham
    Commented May 11, 2016 at 20:57
  • 1
    $\begingroup$ I'm not convinced that relaxation of boundary regularity will help. After all the surfaces with $K\equiv1$ are known to be real-analytic, and they may satisfy something similar to a Schwartz Reflection Principle (the same way minimal surfaces ($H\equiv0$) do), so that having a continuous boundary that contains a line segment would imply that the surface extends smoothly across the line line segment as a surface that satisfies $K\equiv1$, which, of course, cannot happen in the case $K\equiv1$. For example, it could happen that having a real-analytic boundary implies extendability across it. $\endgroup$
    – Robert Bryant
    Commented May 11, 2016 at 21:58

1 Answer 1

Reset to default
2
$\begingroup$

(The original point of this posting was to correct a typo in the equations; now fixed by the OP, and so original deleted.)

Just for visualization purposes, the below shows the four curves, and a surface formed by connecting corresponding points of the two helices.

            HelicesLinesSurface

Improve this answer
$\endgroup$
1
  • 1
    $\begingroup$ For visualization purpose only.The edges belong to a left handed helicoid actually , the surface need not be ruled. $\endgroup$
    – Narasimham
    Commented May 11, 2016 at 21:06

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .