3
$\begingroup$

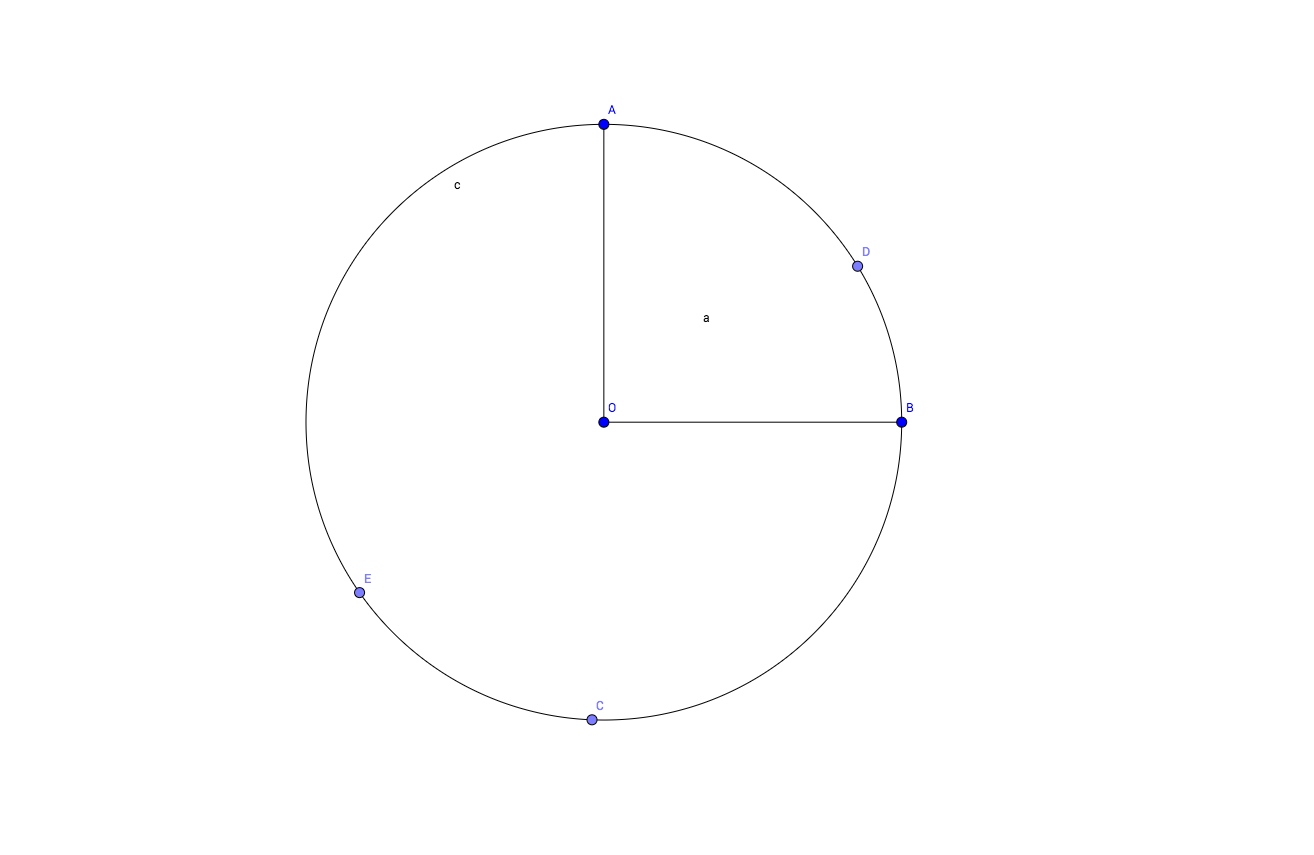
Let ABC be a spherical triangle, where the spherical distance (or angle) AB is $\pi/2$ and $C\neq -A$. For $t\in[0,1]$, let $B(t)$ (resp. $C(t)$) be the only point on the segment $[AB]$ (resp. $[AC]$) such that $AB(t) = t AB$ (resp. $AC(t) = t AC$).

Question: Is it true that $B(t)C(t) \leq 2 BC$ for any $t\in [0,1]$?

The worst case seems to happen when $C$ is close to $-A$, so that $BC\simeq \pi/2$ but $C(t)$ goes in the direction opposite to $B$, so that at some point $B(t)C(t) = \pi$. (See the picture below, where D is that B(t) and E that C(t).)

Worst case

For those who care, the question is related to my paper arXiv:1507.05485 where Lemma 15 is basically the question I ask here but the proof is wrong, as pointed out by a very diligent referee. I can fix the proof, but only with $B(t)C(t) \leq 3 BC$...

Improve this question
$\endgroup$
2
  • 1
    $\begingroup$ Using spherical cosine law it would be left to prove that $$\cos(t\pi/2)\cos(tAC)+\sin(t\pi/2)\sin(tAC)\cos(\alpha)\geq 2\sin^2(AC)\cos^2(\alpha)-1$$ for all $t\in [0,1]$ where $\alpha$ is the angle at $A$. $\endgroup$
    – user35593
    Commented Mar 9, 2016 at 14:54
  • $\begingroup$ @user35593 In the case where $BC \leq \pi/2$, indeed... $\endgroup$
    – Lierre
    Commented Mar 9, 2016 at 15:30

1 Answer 1

Reset to default
2
$\begingroup$

Unfortunately, the answer of user35593 is broken but I obtained something in the same line : $$\cos(B(t)C(t)) = \cos(tAB-tAC) - \sin(tAB)\sin(tAC)(1-\cos a),$$ where $a$ is the angle at $A$. Thus $$\cos(B(t)C(t)) \geq \cos(AB - AC) + \cos a - 1 = \sin(AC) + \cos a -1.$$ We assume that $BC < \frac\pi2$, so that $\cos a \sin(AC) = \cos(BC) > 0$. Since $0\leq AC \leq \pi$ we have $\sin(AC)\geq 0$ and hence $\sin(AC)>0$ and $\cos(a)>0$. This is enough to have $$\sin(AC) + \cos a -1 \geq 2 \cos(a)^2 \sin(AC)^2 - 1 = \cos(2 BC).$$ This gives the claim.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .