Question abouth Skorokhod representation of random variables

Question

It is known that for any two probability measures $\mu$ and $\nu$ on $\mathbb R$ that are close in the Prokhorov metric $\rho$, i.e.

$$\rho(\mu,\nu)<\varepsilon,$$

then there exist two random variables $X$ and $Y$ defined on some probability space s.t. $Law(X)=\mu$, $Law(Y)=\nu$ and

$$P\left[|X-Y|>\varepsilon\right]~<~\varepsilon.$$

My question is the following: If the $\mu$ and $\nu$ above satisfy

$$\left|\int_{\mathbb R}(x-K)^+d\mu(x)-\int_{\mathbb R}(x-K)^+d\nu(x)\right|~<~\varepsilon \mbox{ for all } K\in\mathbb R.$$

Could we find some couple of random variables $X$ and $Y$ s.t. $Law(X)=\mu$, $Law(Y)=\nu$ and

$$E\left[|X-Y|\right]~<~f(\varepsilon),$$

where $f: \mathbb R_+\to\mathbb R_+$ is some continuous function with $f(0)=0$.

Answer 1

The answer to your question is negative: such a function $f$ does not exist.

Indeed, given the distribution functions (d.f.'s) $F$ and $G$ of random variables (r.v.'s) $X$ and $Y$, the smallest value of $E|X-Y|$ is the Wasserstein distance between their distributions, which equals $$d(F,G):=\int_0^1|F^{-1}(u)-G^{-1}(u)|\,du; $$ see e.g. (2) in [1], where $$F^{-1}(u):=\inf\{x\in\mathbb R\colon F(x)\ge u\}. $$

Let $F(x)=(1-1/x)I\{x>1\}$ for real $x$, where $I\{\cdot\}$ is the indicator function. Then $F$ is the distribution function (d.f.) of a random variable (r.v.) $X$.

Take any real $\epsilon>0$ and let $h_n:=\epsilon/\sqrt n$ for natural $n$. Define $x_n\ge1$ and $y_n>x_n$ for natural $n$ by induction. Let $x_1:=1$. For all natural $n$, let $y_n:=(1+h_n)x_n$. For $z\ge x_n$, let $$d_n(z):=\int_{x_n}^z\Big(\frac1x-\frac1{y_n}\Big)\,dx=\ln\frac z{x_n}-\frac{z-x_n}{y_n}. $$ Then $d_n$ continuously decreases on $[y_n,\infty)$ from $d_n(y_n)>0$ to $-\infty$. So, for some unique $x_{n+1}>y_n$ one has $$0=d_n(x_{n+1})=\int_{x_n}^{x_{n+1}}\Big(\frac1x-\frac1{y_n}\Big)\,dx. $$ Thus, $x_n$ and $y_n$ are defined for all natural $n$, with $x_n<y_n<x_{n+1}$.

Define now the d.f. $G$ of a r.v. $Y$ by the conditions: $G=F[=0]$ on $(-\infty,1)$ and $G=F(y_n)=1-1/y_n$ on $[x_n,x_{n+1})$ for each natural $n$. Then $$\int_{\mathbb R}|F(x)-G(x)|\,dx\ge\sum_{n=1}^\infty\int_{x_n}^{y_n}|F(x)-G(x)|\,dx =\sum_{n=1}^\infty d_n(y_n)=\infty, $$ since $d_n(y_n)=\ln(1+h_n)-\frac{h_n}{1+h_n}\sim h_n^2/2=\epsilon^2/(2n)$.

On the other hand, for any real $t>1$, let $n=n_t$ be the unique natural number such that $x_n<t\le x_{n+1}$; then $$|E(t-X)_+-E(t-Y)_+| =\Big|\int_{-\infty}^t(F(x)-G(x))\,dx\Big|$$ $$=d_n(t)\le d_n(y_n)<\epsilon^2/(2n)\le\epsilon^2/2, $$ and $|E(t-X)_+-E(t-Y)_+|=0$ if $t\le1$. (I use $-X,-Y,t=-K$ instead of your $X,Y,K$.)

Thus, $\sup_{t\in\mathbb R}|E(t-X)_+-E(t-Y)_+|\le\epsilon^2/2$, which can be made arbitrarily small, while the smallest value of $E|X-Y|$ for r.v.'s $X$ and $Y$ with these d.f.'s $F$ and $G$ is $d(F,G)=\int_0^1|F^{-1}(u)-G^{-1}(u)|\,du=\int_{\mathbb R}|F(x)-G(x)|\,dx=\infty$; the penultimate equality here reflects the two ways to compute the area between the graphs of $F$ and $G$ (which is the same as the area between the graphs of $F^{-1}$ and $G^{-1}$).

Here the graphs of these $F$ and $G$ are shown for $\epsilon=0.5$; each even-numbered gray area equals the preceding odd-numbered gray area:

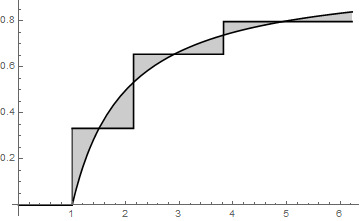
Remark. Instead of $h_n:=\epsilon/\sqrt n$, one could simply set $h_n:=h:=\epsilon$, so that $h_n$ not depend on $n$. Then the proof would be simplified a bit. However, with $h_n:=\epsilon/\sqrt n$, one has the extra property that $E(t-X)_+-E(t-Y)_+\to0$ as $t\to\infty$.