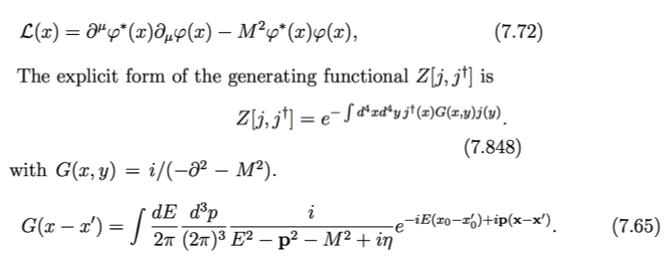
The Lagrangian for the complex scalar field is$$\mathcal{L} = \partial_\mu \phi^* \partial^\mu \phi - m^2 \phi^* \phi.$$Can anyone work out or provide me a reference to the computation of the generating functional$$Z[J, J^*] = \int \mathcal{D}\phi \,\exp\left\{i \int d^4x\,[\mathcal{L} + J(x)\phi^*(x) + J^*(x)\phi(x)]\right\}?$$I need this result for my research, but unfortunately, I am not a physicist by training. Thank you very much!
$\begingroup$
$\endgroup$
3
-
5$\begingroup$ Looks like a QFT homework problem. $\endgroup$– Jeff HarveyCommented Oct 12, 2015 at 22:58
-
$\begingroup$ @JeffHarvey --- it might be --- but since it got a whole bunch of upvotes, I thought I'd answer it to get it out of the "unanswered" queue $\endgroup$– Carlo BeenakkerCommented Oct 13, 2015 at 6:14
-
$\begingroup$ Yes, but I now know for a fact that it was a homework problem and I don't think we should be encouraging people to post homework problems here by answering them. $\endgroup$– Jeff HarveyCommented Nov 20, 2015 at 23:32
Add a comment
|
1 Answer
$\begingroup$
$\endgroup$
you can find this calculation, for example, in chapter 7 of Kleinert's path integral book, I reproduce the relevant equations: