Alexander duality for non-manifolds

Question

Let $X$ be a CW complex and $A$ a subcomplex. I will assume that both are compact, and that $X$ is $n$-dimensional. Furthermore, assume that the local homology of $X$ is that of a manifold in a range. That is, there is a number $k \leq n$ with the property that for each $x \in X$,

$$H_*(X, X\setminus \{ x \}) = 0 \mbox{ for } * < k$$

If $X$ were actually a manifold we would have some form of Alexander duality relating the (co)homology of $A$ and its complement in $X$. For instance, if $X = S^n$, then

$$H_*(A) \cong H^{n-*-1}(X\setminus A)$$

and more generally, there is a statement relating the homology of $A$ to the cohomology of $X$ relative to $X \setminus A$.

Does anything of the sort hold in the setting that I have described above, where $X$ is only a local homology manifold "in a range?" If an isomorphism like the above were to hold, I would for instance expect that the range in which it is true would depend upon the number $k$.

Answer 1

No, I do not think that hypothesis buys you anything. The complementary hypothesis, $H_i(X,X-{x})=\tilde H_i(S^n)$ for $i\geqslant i_0$ does yield duality in a range. The tool for systematically exploiting such hypotheses is Verdier duality.

Alexander duality is mainly Poincaré duality, plus a comparison of the cohomology of a subspace and the limit of the cohomology of its neighborhoods. If the space is a neighborhood retract, there is a cofinal sequence of equivalent neighborhoods. In general, this comparison requires Čech cohomology. Alexander duality in the sphere involves another step, using the long exact sequence of a pair and the fact that a sphere has little cohomology, but that is not relevant to your question. So I will focus on Poincaré duality. Its generalization exploiting local structure is Verdier duality. I will explain how your hypothesis and variations interact with Verdier duality.

Verdier duality says that the homology of the one-point compactification of a space is isomorphic to the sheaf cohomology with coefficients in the dualizing sheaf: $\tilde H_i(Y^+)\cong H^{-i}(Y;D)$. Note the negative sign, from considering a chain complex as a cochain complex. The dualizing sheaf is not a sheaf, but an object of the derived category of chain complexes of sheaves, equivalently, a homotopy sheaf with values in chain complexes. A representative is given by the presheaf of chain complexes $\Gamma(U,D)=C_*(X,X-U)\simeq C_*(U^+,*)$.

Thus the stalks are the local homologies $D_{X,x}=H_*(X,X-\{x\})$. This is particularly easy to see in a locally conical space, where the limit has a constant final subsequence, but maybe a general CW complex is harder. Thus if $X$ is $n$-dimensional, the dualizing sheaf is supported homological degrees $0$ through $n$, which in cohomological terms are degrees $-n$ through $0$. If $X$ is a simplicial complex, then the support of the $H^{-i}(D)$ is contained in the $i$-skeleton because the neighborhood is a product with an $i$-simplex and thus the link is an $i-1$-suspension.

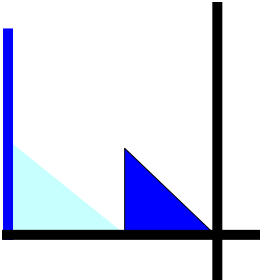
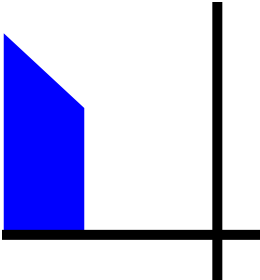
We exploit the stalks by a hypercohomology spectral sequence $E_2^{p,q}=H^q(Y;H^p(D))\Rightarrow H^{p+q}(Y;D)$. Since the dualizing sheaf is concentrated in non-positive degrees, this is a second quadrant spectral sequence. I believe that its support also satisfies $p+q\leqslant$, which I noted above for simplicial complexes and is clearly true for $E_\infty$. Your hypothesis, that $D$ is supported in degrees $-n$ through $-i_0$ does not seem to provide any control. But the complementary hypothesis that its truncation to that range is the constant sheaf $\mathbb Z$ means that the $E_2$ page is, in a range, only a single vertical line, so it collapses with the desired value. (You may also wish to consider the possibility that the dualizing sheaf is approximated by a non-trivial local system, as with unorientable manifold.)

Here are some illustrations of the support of the spectral sequence under various hypotheses. The first is the general picture of the spectral sequence:

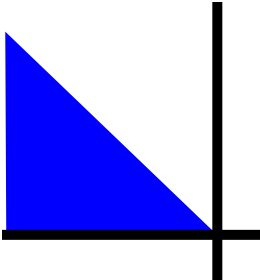
The second is the restriction of support due to your hypothesis:

The third is the restriction of support due to the complementary hypothesis, shown in blue, with the collapse region show in light cyan: