0
$\begingroup$

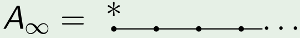
Let $(N \subset M)$ be a unital inclusion of hyperfinite ${\rm II}_1$ factors, with the following principal graph (called TLJ) enter image description here
Question: Is such a subfactor unique (up to ${\rm W}^*$-isomorphism) at fixed index (if it exists)?

Remark: It is true in the amenable case, but we ask in general.

Alternative: we can also ask the same question replacing "hyperfinite" by "isomorphic to $L(\mathbb{F}_{\infty})$".
In this case the existence is known for every index $\ge 4$.

Improve this question
$\endgroup$
2
  • $\begingroup$ Do you mean subfactors of the hyperfinite? Otherwise what do you mean by unique, as you could just change the factor? If you do mean of the hyperfinite, then existence is open, so what you mean by uniqueness is again unclear (unique, if it exists?). $\endgroup$
    – Noah Snyder
    Commented May 7, 2015 at 14:32
  • $\begingroup$ @NoahSnyder: you're right, thank you! I've clarified the post. $\endgroup$
    – Sebastien Palcoux
    Commented May 7, 2015 at 14:49

1 Answer 1

Reset to default
2
$\begingroup$

For your original question I'm pretty sure the answer is obviously no, because you can start with Popa's construction of a TLJ subfactor of $L(F_\infty)$ and then just change the factor in some boring way. For example, start with $N \subset M$ Popa's inclusion of $L(F_\infty)$ factors and then tensor it with the hyperfinite to get $N \otimes R \subset M \otimes R$. Unless I'm missing something, this won't change the standard invariant, and since the free group factors aren't McDuff this gives a different subfactor.

For the hyperfinite $\mathrm{II}_1$ basically everything about TLJ at index above 4 is open. In particular, it's open to determine for which indices it exists and it's open to determine for which indices it's unique. (Just to give a glimpse of how open things are, I think it's open whether there are any transcendental indices realized, whether there is any index at all that isn't realized, and whether there's any index which can be realized in infinitely many distinct ways.)

For the $L(F_\infty)$ case, my understanding was that it's not unique, though I don't myself know how to prove that. I don't know whether this has been proven or is just expected.

Improve this answer
$\endgroup$
3
  • $\begingroup$ The corollary 4.5. p994 of Popa's paper in ICM1990II states that << the set of indices of irreducible subfactors of the hyperfinite type ${\rm II}_1$ factor has a gap between $4$ and $4.026$>>. What's wrong? $\endgroup$
    – Sebastien Palcoux
    Commented May 28, 2015 at 10:23
  • 1
    $\begingroup$ The proof has never appeared. $\endgroup$
    – Noah Snyder
    Commented May 28, 2015 at 22:28
  • $\begingroup$ On p994 there are two theorems (4.2. and 4.3.) and two corollaries (4.4. and 4.5.). Are they some of them proved today? Do you know if this last paper of Popa and Vaes goes in this direction? $\endgroup$
    – Sebastien Palcoux
    Commented May 29, 2015 at 2:39

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .