Illumination of a convex body

Question

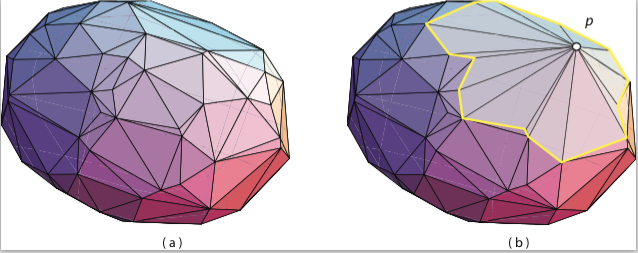
If $\mathbf{K}$ is a compact, convex set with nonempty interior in $d$-dimensional Euclidean space $\mathbb{E}^d$, and $\mathbf{p}$ is an exterior point outside of $\mathbf{K}$, we say that $\mathbf{p}$ 'illuminates' point $\mathbf{q} \in \mathbf{K}$ if the line from $\mathbf{p}$ to $\mathbf{q}$ intersects the interior of $\mathbf{K}$.

Suppose we let $\mathbf{K}_1$ be the set illuminated by $\mathbf{p}$ and $\mathbf{K}_2=\delta \mathbf{K} \setminus \mathbf{K}_1$ (where $\delta \mathbf{K}$ is the boundary of $\mathbf{K}$). My question is: are these two sets ($\mathbf{K}_1$ and $\mathbf{K_2}$) linearly separated by some hyperplane?

Answer 1

If I have understood your question correctly, the answer is No already in $\mathbb{R}^3$, for the "shadow" boundary is, in general, quite irregular:

---

 
  ^{(Figure 2.14 from Discrete and Computational Geometry, p.54. (a) Convex hull $Q$. (b) Hull of $p \cup Q$, with $p$ exterior to $Q$.)}

---

Answer 2

The answer depends on the point $p$ and on the body. Here is a simple counterexample in $R^3$. Take two regular hexagons in parallel planes, so that the line $L$ connecting their centers is perpendicular to these planes. One hexagon is obtained from another by rotation by 30 degrees about the center and parallel translation along the line $L$. The body is the convex hull of the union of the hexagons. The illumination source is somewhere on $L$. I suppose this is simpler that Joseph's example.