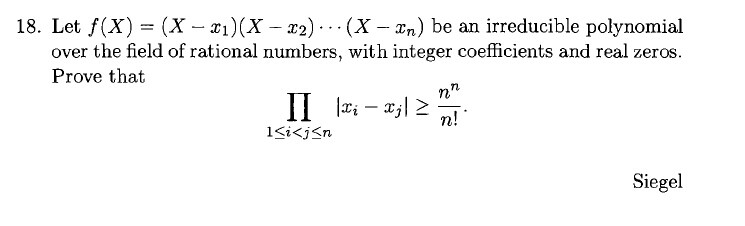8
$\begingroup$

Question:

Let $$f(X)=(X-x_{1})(X-x_{2})\cdots (X-x_{n})$$ be an irreducible polynomial over the field of rational numbers,with integer coefficients and real zeros.

Prove that $$\prod_{1\le i<j\le n}|x_{i}-x_{j}|\ge\dfrac{n^n}{n!}$$

This result is fell interesting, and This problem is from problem book, and this problem is name Siegel created it, and I searched sometimes and can't find it.and can't solve this problem, can someone help me? enter image description here

Improve this question
$\endgroup$
5
  • 9
    $\begingroup$ See Siegel's book "Lectures on the geometry of numbers" (pages 27 and 28). $\endgroup$
    – Lucia
    Commented Dec 12, 2014 at 12:32
  • 5
    $\begingroup$ Here is Google Books link to the reference given by Lucia. I would be quite curious to know the name of the book where you saw this problem. $\endgroup$
    – Martin Sleziak
    Commented Dec 12, 2014 at 13:20
  • 1
    $\begingroup$ @MartinSleziak,is from ：Problems From the Book by Titu Andreescu Page 312 $\endgroup$
    – math110
    Commented Dec 16, 2014 at 16:20
  • $\begingroup$ Is this perhaps related to the Van der Waerden conjecture (now theorem) on the end of this page? en.wikipedia.org/wiki/Doubly_stochastic_matrix $\endgroup$
    – Per Alexandersson
    Commented Dec 16, 2014 at 20:38
  • 1
    $\begingroup$ Not sure why there is a bounty when Lucia already gave an answer; perhaps another place to look would be Goldman's The Queen of Mathematics in which you will find the same result. See Chapter 22 pp. 461-462 (here) in which the result is discussed as a special case of Minkowski's proof of a conjecture due to Kronecker. $\endgroup$
    – Benjamin Dickman
    Commented Dec 23, 2014 at 6:19

0

Reset to default

You must log in to answer this question.

Browse other questions tagged .