Orthogonal mud cracks and Maxwell's reciprocal figures

Question

Is there a succinct mathematical/physical explanation of why mud cracks tend to meet orthogonally?

---

         
          ^{Wikipedia image in this article}

---

There is considerable literature, which I have only skimmed (I will admit), but so far I have not encountered an intuitive explanation. If anyone can either provide a high-level explanation, or point me to an appropriate source, I would appreciate it.

This is unjustified speculation, but I wonder if there is any connection to Maxwell's reciprocal figures:

---

         

---

James C. Maxwell, "On Reciprocal Figures and Diagrams of Forces," 1864. (journal link).

Answer 1

Is the following physical argument "intuitive" enough?

Mud is a mixture of soil and water, and as it dries, the proportion of water decreases. As this occurs, the mud would like to contract relative to its original volume. Thus tensile stresses are generated.

A crack that forms opens up a gap in the surface of the mud, which allows the mud to relax the tension in the direction perpendicular to the crack. The stresses in the direction parallel to the crack are not relieved, and so the large tension in that direction will then open up a crack that is perpendicular to the first one. And so on...

From my brief skim of the literature, I gathered that the following paper of Lachenbruch proposed this idea early on in the literature, but I was unable to get a copy of it.

Lachenbruch, A.H., 1962. Mechanics of thermal contraction cracks and ice-wedge polygons in permafrost. Geological Society of America Special Paper 70, 1-69.

I don't see any particular connection between crack formation and Maxwell's reciprocal figures, which describe forces and stresses in an equilibrium system.

Amusingly, there are also crack patterns that appear to be hexagonal. This I don't understand as well, but I did find this paper by Lucas Goehring which argues that this happens if repeated drying and cracking cycles occur. See Figure 3 from that paper:

Answer 2

I am not sure about the physical interpretation, but there is a mathematical property which might be relevant.

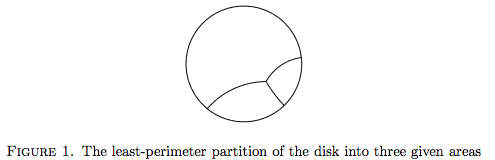
If you have a domain and you want to divide it into two subdomains of given volums (e.g., the two subdomains are to have equal volumes), while minimizing the length of the frontier, then the optimal solution must be a segment or an arc of circle meeting the boundary orthogonally (as shown by an easy variational argument). This argument could explain not only the orientation, but also the shape of the cracks (which look quite like pieces of circles, although it might be a stretch of the data you provided).

Repeatedly dividing a domain in this way produces patterns that look like the ones in your picture (this is what triggered my answer). This model of division has been proposed by D'Arcy Thompson in his wonderful "On Growth and Form" to model the division of some vegetal cells (which are pretty rigid). By the way, this book is probably a huge source of inspiration for such questions, and despite its fame I am not sure that mathematicians have studied most of the question it raises.

This model also has potential to explain the presence of 120° angles, as if you divide a domain in more than two subdomains at once, while minimizing the total length of all needed cracks, then the solution certainly has triple of cracks meeting at 120° angles (by classical variational arguments again).

Physically, the "minimize length of cracks" part is pretty plausible (one can guess that the energy cost of a crack is proportional to its length), but I do not see a good explanation for the "fixed volume" hypothesis.

Answer 3

Following up Benoît Kloeckner's remarks, I include two images below, the first from the paper, "Least-perimeter partitions of the disk into three regions of given areas" (arXiv abs link), the second from D'Arcy Thompson's On Growth and Form:

---

---

---

Thompson explains the circumstances in which $120^\circ$ angles are preferred in some regions of the wing over the more prevalent $90^\circ$ angles. The three arcs in the disk in Fig.1 meet at $120^\circ$, and meet the circumcircle at $90^\circ$.

Answer 4

Suggest you follow this link for a discussion on similar cracking that forms columnar basalt during the cooling of lava. I have collected several samples (from the Mt Baker, WA area) that seem to confirm that the "perfect" shape is that of a hexagon, but that conditions were often not nearly perfect resulting in a variety of other shapes in cross section.

Regardless of the cross section shape, the cross section at different locations in the same column may vary in size. Sometimes this variance is considerable.

http://blogs.agu.org/georneys/2012/11/18/geology-word-of-the-week-c-is-for-columnar-jointing/