5
$\begingroup$

Let $\mathfrak g$ be the semisimple Lie algebra of type $D_{4}$. Let $\sigma$ be the 3-rd order automorphism of $\mathfrak g$ induced by the triality of $D_{4}$:

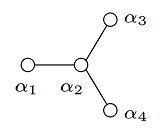enter image description here $$ \sigma:\alpha_{1}\mapsto\alpha_{3}\mapsto\alpha_{4},\alpha_{2}\mapsto\alpha_{2} $$

Let $\mathfrak g^{\sigma}$ be the subalgebra of $\sigma$-stable elements of $\mathfrak g$.

How can Serre generators of $\mathfrak g^{\sigma}$ be expressed through Serre generators of $\mathfrak g$?

I suppose that "Serre generators", "Serre-Chevalley generators", and "Chevalley generators" are equivalent terms. (In my opinion, perhaps the use of a Chevalley basis would be more straightforward?)

Improve this question
$\endgroup$
4
  • 1
    $\begingroup$ I think that you will find all the information you need in this case in Levasseur, T.; Smith, S. P. Primitive ideals and nilpotent orbits in type G2. J. Algebra 114 (1988), no. 1, 81–105. $\endgroup$
    – Bulois Michael
    Commented Nov 28, 2014 at 10:49
  • $\begingroup$ @BuloisMichael Thanks. Somehow, I'm not surprised that one of the authors is from UPMC. :-) $\endgroup$
    – Jake
    Commented Nov 30, 2014 at 11:22
  • $\begingroup$ @BuloisMichael Indeed, quite enlightening. Perhaps you can post it as an answer so I can select it as best answer. $\endgroup$
    – Jake
    Commented Nov 30, 2014 at 12:07
  • 2
    $\begingroup$ @Jake: It's a good idea to look also at the careful treatment of fixed point subalgebras under graph automorphisms in Section 9.5 of Roger Carter's book Lie Algebras of Finite and Affine Type (Cambridge, 2005), including the choice of basis under a graph automorphism. Carter's book is also useful because he later generalizes many of the ideas to affine Lie algebras. $\endgroup$
    – Jim Humphreys
    Commented Dec 1, 2014 at 14:57

1 Answer 1

Reset to default
5
$\begingroup$

In the G$_2$ case, I think that you will find all the information you need in Levasseur, T.; Smith, S. P. Primitive ideals and nilpotent orbits in type G2. J. Algebra 114 (1988), no. 1, 81–105.

Things can also be viewed in a very nice symmetric way using 4-ality. See Section 3.4 of J. Landsberg and L. Manivel, Representation theory and projective geometry. In: Algebraic transformation groups and algebraic varieties, Enc. Math. Sci., 132, Springer-Verlag, 2004, 131-167.

Improve this answer
$\endgroup$
1

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .