In a fibration, can a deformation retraction of the base be lifted to the total space?

Question

Given a fibration $p:E \rightarrow B$ and if $A$ is a deformation retract of $B$. Is it true that $p^{-1}(A)$ is a deformation retract of $E$?. If this is not true, can some conditions be imposed on $p$, $E$ or $B$ to make that statement true?. If so, the theorem is still valid for a strong deformation retract?

Answer 1

Write $E_A = p^{-1}(A)$. Under mild conditions, the inclusion $$ (E_A \times I)\cup (E \times \{ 0\}) \to E\times I $$ will be a cofibration and a (weak) homotopy equivalence. Now set up the lifting/extension problem

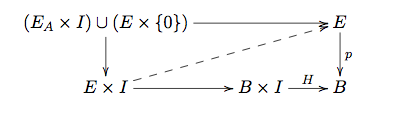
where $H$ is the deformation retraction. The dashed arrow can then be found and it is the deformation retraction you want.

Mild conditions: $A\hookrightarrow B$ is a closed (as Mark Grant mentions) cofibration; then $E_A\hookrightarrow E$ is a cofibration and so is $(E_A \times I)\cup (E \times \{ 0\}) \to E\times I$.

Answer 2

The details of Jeff's answer can be filled in using results of Strøm (presumably unrelated!).

By Theorem 4 in

Strøm, Arne Note on cofibrations. I. Math. Scand. 19 1966 11–14,

Jeff's diagram has a solution whenever $(E,E_A)$ is a closed cofibration. But by results in

Strøm, Arne Note on cofibrations. II. Math. Scand. 22 1968 130–142

this is true whenever $i: A\hookrightarrow B$ is a closed cofibration. So these could be the "mild conditions" our Strom mentioned.

Answer 3

To clarify one point in the previous answers of Jeff and Mark: There are two different definitions of "deformation retraction" that are often used. In the stronger notion the subspace has to be pointwise fixed during the homotopy, while in the weaker version it only needs to be setwise invariant during the homotopy. The original question seems to be using the weaker definition. With this definition it's clear that a lift of a deformation retraction of $B$ to $A$, starting with the identity map of $E$, is a deformation retraction of $E$ onto $p^{-1}(A)$. This lift always exists using the usual definition of a fibration as a map satisfying the homotopy lifting property for all spaces. However, there is in general no guarantee that a lift of a strong deformation retraction will be a strong deformation retraction. The cofibration property for $(B,A)$ suffices to give this "strong" version of the result, as Jeff and Mark explained.

Answer 4

There is another situation when we can lift a strong deformation retraction (SDR). If your fibration $p:E\to B$ is a discrete covering ($\pi^{-1}(b)$ has the discrete topology for every $b\in B$), then no additional assumptions are needed.

Indeed, by definition, the fibration $p$ has the homotopy lifting property from arbitrary spaces. To be concrete, in the diagram, we can lift the the bottom map $H$ to the dashed arrow $\tilde{H}$, so that $H\circ(id_{I}\times p)=p\circ\tilde{H}$:

$$ \begin{eqnarray*} I\times E & \stackrel{\tilde{H}}{\dashrightarrow} & E\\ id_{I}\times p\downarrow\quad & & \downarrow p\\ I\times B & \stackrel{H}{\to} & B \end{eqnarray*} $$

In general, $\tilde{H}$ is only a weak retraction. However, when the fibers of $p$ are discrete, and $H$ is an SDR to $A\subset B$, then $\tilde{H}$ also an SDR from $p^{-1}(B)=E$ to $p^{-1}(A)$.

To prove this, using $H(t,a)=a$ for all $t$, and all $a\in A\subset B$, we get: $$ \tilde{H}(t,c)\in p^{-1}(H\circ(id_{I}\times p)(t,c))=p^{-1}(H(t,p(c)))=p^{-1}(p(c)). $$ Hence, $\tilde{H}(t,c)=c$, for all $c\in p^{-1}(a)$, as wanted, by discreteness and continuity.