Write $E_A = p^{-1}(A)$. Under mild conditions, the inclusion $$ (E_A \times I)\cup (E \times \{ 0\}) \to E\times I $$ will be a cofibration and a (weak) homotopy equivalence. Now set up the lifting/extension problem
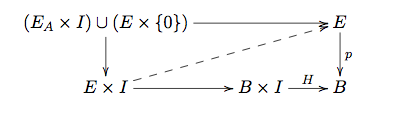
where $H$ is the deformation retraction. The dashed arrow can then be found and it is the deformation retraction you want.
Mild conditions: $A\hookrightarrow B$ is a closed (as Mark Grant mentions) cofibration; then $E_A\hookrightarrow E$ is a cofibration and so is $(E_A \times I)\cup (E \times \{ 0\}) \to E\times I$.
