If you're in the need for computing all positive roots of given root system of type $X_n$ in a way that involves only clicking (quite a few times) on your mouse and without much thinking, then I recommend:
Download Keller's mutation applet (available here: https://webusers.imj-prg.fr/~bernhard.keller/quivermutation/).
Open the applet, and under File > New Quiver, input "1" for the "Enter side length". You'll see a single circle (labelled 1) in the center of the applet.
Create a Dynkin quiver (i.e. the Dynkin diagram with some choice of orientation) of type $X_n$, by clicking on "Add nodes" and "Add arrows", and then click "Done".
Under Clusters > Activate, click on d-vectors, and then again click "Done". You'll see the negative of the $n$ standard basis vectors (labelled $d[1], \ldots, d[n]$) of $\mathbb{R}^n$ appearing (twice, because we click on "Done").
Look for a source of the quiver (i.e. a vertex with all arrows pointing away from that vertex), and click on it. Two things will happen:
i) The quiver will update itself (this is called quiver mutation).
ii) A new vector will appear at the bottom of the applet, with non-negative coefficients. This is a positive root, expressed as a linear combination of the simple roots (where the ith coordinate of the vector is the coefficient of $\alpha_i$ in its expansion).
- Repeat step 5) at a vertex you have not yet clicked on.
The step 5) - step 6) loop will run $n$ times, after which you will have "clicked" on every vertex exactly once. Now "forget" that you have clicked on any vertices, and repeat the step 5) - step 6) loop (performing another $n$ clicks), etc. Eventually, all positive roots will appear at the bottom of the applet.
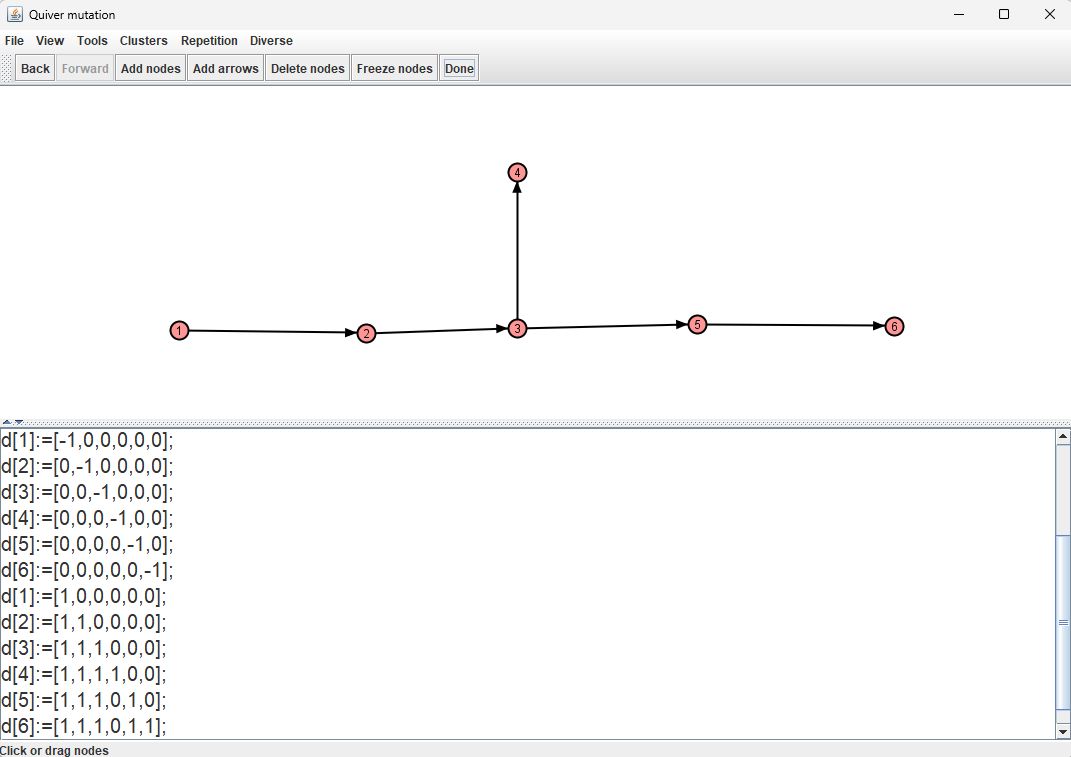
I've attached below what this process looks like in type $E_6$, after having performed the step 5) - step 6) loop $6$ times. The algorithm has picked up the roots $\alpha_1$, $\alpha_1 + \alpha_2$, $\alpha_1 + \alpha_2 + \alpha_3$, $\alpha_1 + \alpha_2 + \alpha_3 + \alpha_4$, $\alpha_1 + \alpha_2 + \alpha_3 + \alpha_5$ and finally $\alpha_1 + \alpha_2 + \alpha_3 + \alpha_5+\alpha_6$. If I click on the vertices along the sequence $(1,2,3,4,5,6)$ I will get another six positive roots in the root system, etc.