Suppose a planar curve $C$ is defined by parametric equations in $t$: $x(t)$ and $y(t)$. Are there conditions on these two functions that guarantee that $C$ does not self-intersect?
For example, the Maclaurin trisectrix can be defined by $$x(t) = \frac{t^2-3}{t^2+1}, \;\;\;\;\;y(t)=\frac{t(t^2-3)}{t^2+1}$$ and it self-intersects:
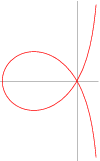
So, rational functions do not suffice to imply non-self-intersection. Pointers would be appreciated—Thanks!
