I'm reading a paper in which the following is done. We have a certain particular map of spaces $f:X\to Y$ and then it is said something along the lines of "let $Z_f$ denote the space whose defining attribute is that it is the space that fits into the following fibration sequence up to homotopy":
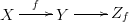
Then it is said "To speak of the sequence above as a homotopy (co)fibration sequence, one needs to specify a preferred null-homotopy for the composite map".
(the (co) is mine, since in fact the writer is working with spectra, and in my interpretation they are being replaced by spaces, where no longer cofibration=fibration)
I don't really understand what's going on. I'm aware of the general fact that the nullhomotopies of $gf$ in the following diagram are in one to one correspondence with the extensions $G$ of $g$ to the homotopy cofiber:
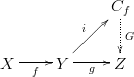
Returning to the case above, why is it needed to specify a preferred null-homotopy for the composite map? Can't we just specify a map $Y\to Z_f$ and prove that the composition is nullhomotopic?
