Given a commutative square in a nice category, say, manifolds $Mfd$. Suppose all edges are submersions (I guess transverse should be OK), then the square is a pullback if and only if it locally is, i.e. for a (or all, no difference) cover $U_i$ of C, we have $P_i=A_i \times_{U_i} B_i$, where $A_i=A\times_C U_i$, similarly define $B_i$, $P_i$.
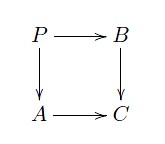
I can show it by hand, the question is how to show this assertion more abstractly? (It seems related to decent theory?) Thanks a lot.
