What's the name of this distribution?

Question

What is the official name of this distribution, $$k\exp(-\lambda|x|^\alpha),$$ where $\alpha$ is usually less than 1, but greater than 0? More importantly, could anyone tell me what the variance of this distribution is? Thank you very much.

---

(Edit: Oct 7 '13 at 21:37) Thank you all.

This distribution can be called generalized normal distribution, whose standard form is

$$f(x)=\beta/2\alpha\Gamma(1/\beta)\exp(-|(x-\mu)/\alpha|^\beta)$$

whose variance is $$\alpha^2 \Gamma(3/\beta)/\Gamma(1/\beta)$$

Answer 1

While Nadarajah (2005) may have used the term 'generalised Normal' to describe a density that nests this form, there are more suitable names that extend far further back in time, and which accordingly seem much more appropriate.

In particular, I believe this should properly be referred to as a Subbotin distribution (Subbotin 1923). Other later references include:

• Diananda (1949)

• Turner (1960)

• Zeckhauser and Thompson (1970)

• McDonald and Newey (1988)

• Mineo and Ruggieri (2005)

The functional form given by Subbotin (1923) defines the pdf as:

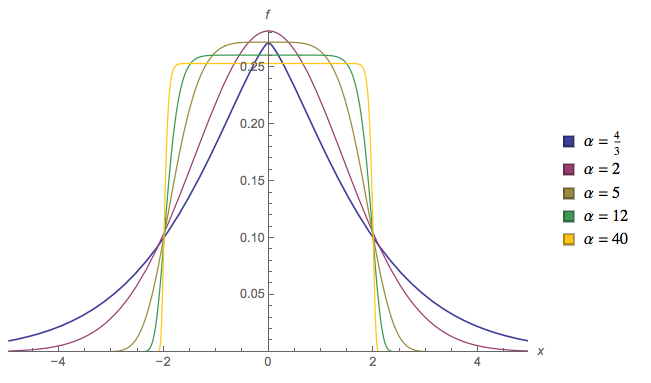
$$f(x) = \frac{\alpha }{2 b \Gamma \left(\frac{1}{\alpha }\right)}\text{exp}\left[-\left|\frac{x}{b}\right|^{\alpha }\right]$$

Subbotin used parameter $b = 1/h$, but the functional form is otherwise identical to that given here. In this form: $$Var(X) = \frac{b^2 \Gamma \left(\frac{3}{\alpha}\right)}{\Gamma \left(\frac{1}{\alpha}\right)}$$

Here is a plot of the pdf with $b=2$, as parameter $\alpha>1$ varies:

(source)

and again for parameter $0<\alpha<1$:

(source)

Other names include: Box-Tiao distribution (McDonald and Newey 1988), and Power-Exponential (McDonald and Newey 1988, Johnson et al. 1995). Finally, it is worth noting that some economic papers inappropriately ascribe the name 'Subbotin distribution' to an Exponential-Power distribution that has a different functional form.

References

• Subbotin, M.T. (1923), On the law of frequency of error, Matematicheskii Sbornik, 31, 296-301.

• Diananda, P. H. (1949), Note on some properties of maximum likelihood estimates, Proceedings of the Cambridge Philosophical Society, 45, 536-544.

• Turner, M. E. (1960), On heuristic estimation methods, Biometrics, 16(2), 299-301.

• Zeckhauser, R. and Thompson, M. (1970), Linear regression with non-normal error terms, The Review of Economics and Statistics, 52, 280-286.

• McDonald, J. B. and Newey, W. K. (1988), Partially adaptive estimation of regression models via the generalized t distribution, Econometric Theory, 4, 428-457.

• Mineo, A. M. and Ruggieri, M. (2005), A software tool for the Exponential Power distribution: the normalp package, Journal of Statistical Software, 12(4), 1-21.

Answer 2

According to Maple, the variance is $$ \dfrac{\sqrt{3}\; 27^{1/\alpha}}{6\pi} \Gamma\left(\dfrac{3+\alpha}{3\alpha}\right) \Gamma\left(\dfrac{3+2\alpha}{3\alpha}\right) \lambda^{-2/\alpha} $$