The sphere is symmetric with respect to any rotation. However, it loses this property as soon as it is triangulated. Are there sequences of triangulations that possess particular large symmetry groups as the number of triangles goes to infinity?
$\begingroup$
$\endgroup$
7
-
1$\begingroup$ Thank you. I am referring to all isometries of the sphere that preserve the triangulation. $\endgroup$– warsagaCommented Oct 6, 2013 at 16:32
-
4$\begingroup$ Well, the "largest" finite subgroups of $SO(3)$ are going to be dihedral groups, so I guess that means you'll be looking at bipyramids en.wikipedia.org/wiki/Bipyramid $\endgroup$– j.c.Commented Oct 6, 2013 at 16:43
-
3$\begingroup$ As indicated by j.c., the finite subgroups of SO(3) have closure proper subgroups of SO(3) (the infinite dihedral group). So one cannot find a sequence of triangulations whose symmetry converges to a group containing every rotation as I think you would like. An analogous result holds for every dimensional sphere, essentially by the Zassenhaus lemma. $\endgroup$– Ian AgolCommented Oct 6, 2013 at 16:49
-
1$\begingroup$ @j.c.There are many more triangulations of $S^2$ with dihedral groups of symmetries than just bipyramids. The triangles could be arbitrarily small, arranged in several horizontal layers between the South and the North poles, the layers forming prisms or antiprisms, with two pyramids at the poles. $\endgroup$– Wlodek KuperbergCommented Oct 9, 2013 at 14:37
-
1$\begingroup$ I do not understand why this question has been put on hold. I admit that it has been phrased somewhat ambiguously, but it has been clarified by the comments, and is related to some very hard questions on triangulating or tiling the sphere with small regions. The comments by Ian Agol (though he presumes the intentions of warsaga) and by j.c. (though his - or her - guess is not quite right) indicate their interest in the question, contrary to the reasons given for putting it on hold... $\endgroup$– Wlodek KuperbergCommented Oct 10, 2013 at 21:55
|
Show 2 more comments
2 Answers
$\begingroup$
$\endgroup$
1
If you consider the symmetry group of the regular icosahedron to be "particularly large" (the group contains several rotations about non-coplanar axes and also orientation-reversing reflections), then the following sequence of refinements of the icosahedral triangulation may be of interest to you. Each of them consists of a family of triangles, their mesh converges to zero, while the triangles remain fairly robust, i.e., not too different from being equilateral and not too different from each other. (There is little hope for finding a triangulation consisting of arbitrarily many triangles that are both small and congruent at the same time.)
Start by projecting the faces of the inscribed regular icosahedron to the sphere; get 20 congruent equilateral triangles. Then partition each triangle by connecting the midpoints of its sides by geodesic arcs on the sphere. Each triangle of the first triangulation is replaced by four smaller triangles; the one in the middle remains equilateral, the other three do not. Keep refining the partition recursively. After the $n$-th partition you will have a triangulation consisting of $\ 20\times4^n\ $ triangles, all of approximately the same small diameter, and all not too far from being equilateral. I think the difference between their shapes is not greater than it is after the first partition. The symmetry group for each of these triangulations remains unchanged.
answered Oct 9, 2013 at 17:32
-
$\begingroup$ Either I need to go to sleep or $n^4$ should be $4^n$. $\endgroup$ Commented Oct 10, 2013 at 2:04
$\begingroup$
My apologies to Wlodek, not noticing that Fuller's dome does not match his description. Here is a second attempt...
$\endgroup$
2
Just to supplement Wlodek's description with an image,
here is Buckminster Fuller's 1954 patent drawing:
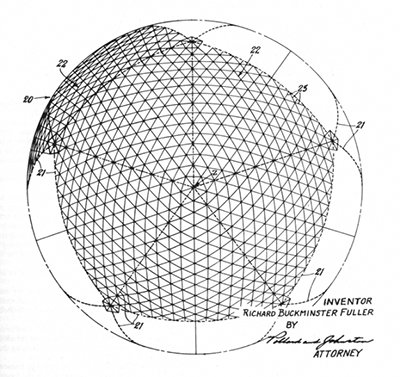
Image from Wikipedia link on B. Fuller page.
My apologies to Wlodek, not noticing that Fuller's dome does not match his description. Here is a second attempt...
answered Oct 9, 2013 at 17:43
-
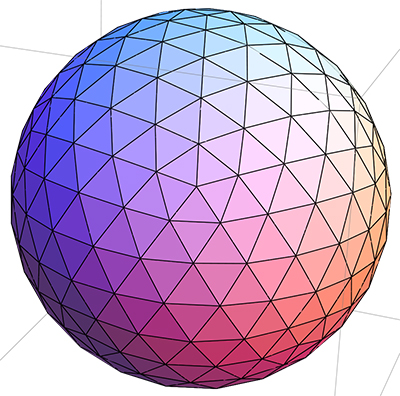
$\begingroup$ Thanks, Joe, but I'm not sure this is the same triangulation. Notice the arc going from the middle of an edge of the big triangle to its vertex. Could you draw a picture showing what I described, with, say, three iterations? $\endgroup$ Commented Oct 9, 2013 at 18:46
-