Are there any results known about the discriminants of indefinite integral binary quadratic forms admitting automorphisms of order 3 or 6? It seems reasonable to expect that any permissible discriminants ought to be quite small and, if so, one would hope that there might be some classical results on the matter.
Thanks!
The structure of the automorphism group becomes clear when one looks at the Conway topograph of a given form. For an indefinite form not representing 0 the topograph has an infinite periodic river separating the positive and negative values, so there is always an infinite cyclic subgroup of the automorphism group, in addition to the order 2 automorphism $(x,y)\mapsto(-x,-y)$ that acts trivially on the topograph. Some forms have additional order 2 symmetries reflecting across lines perpendicular to the river, giving an infinite dihedral subgroup of the automorphism group. If "anti-automorphisms" that change the sign of the values of a form are allowed as automorphisms, there can also be 180 degree rotational symmetries or glide reflection symmetries. Overall, five of the seven frieze groups are realizable as symmetries of the topograph. The two that aren't realizable involve reflections across the river, which cannot be symmetries of the topograph.
In particular all torsion in the automorphism group is 2-torsion, of order either 2 or 4.
Any non-identity automorphism is either an involution (i.e. period $2$) or of infinite order; in particular periods $3$ and $6$ do not occur. Proof: the $\bf Z$-automorphism group is contained in the $\bf R$-automorphism group; but over $\bf R$, an indefinite binary form is equivalent with $Q(x,y) = xy$, whose automorphism group consists of the matrices of the form $\bigl({{a \; 0^{\phantom{-1}}} \atop {0 \; a^{-1}}}\!\bigr)$ or $\bigl({{0^{\phantom{-1}} \; a } \atop {a^{-1} \; 0}}\!\bigr)$, of which the latter is always an involution, and the former is of infinite order unless $a=1$ (identity) or $a=-1$ (involution). $\Box$
no 3 torsion. see Theorem 6.12.4 on pages 132-133, Binary Quadratic Forms by Buchmann and Vollmer. Or pages 31-34 of Binary Quadratic Forms by Buell.
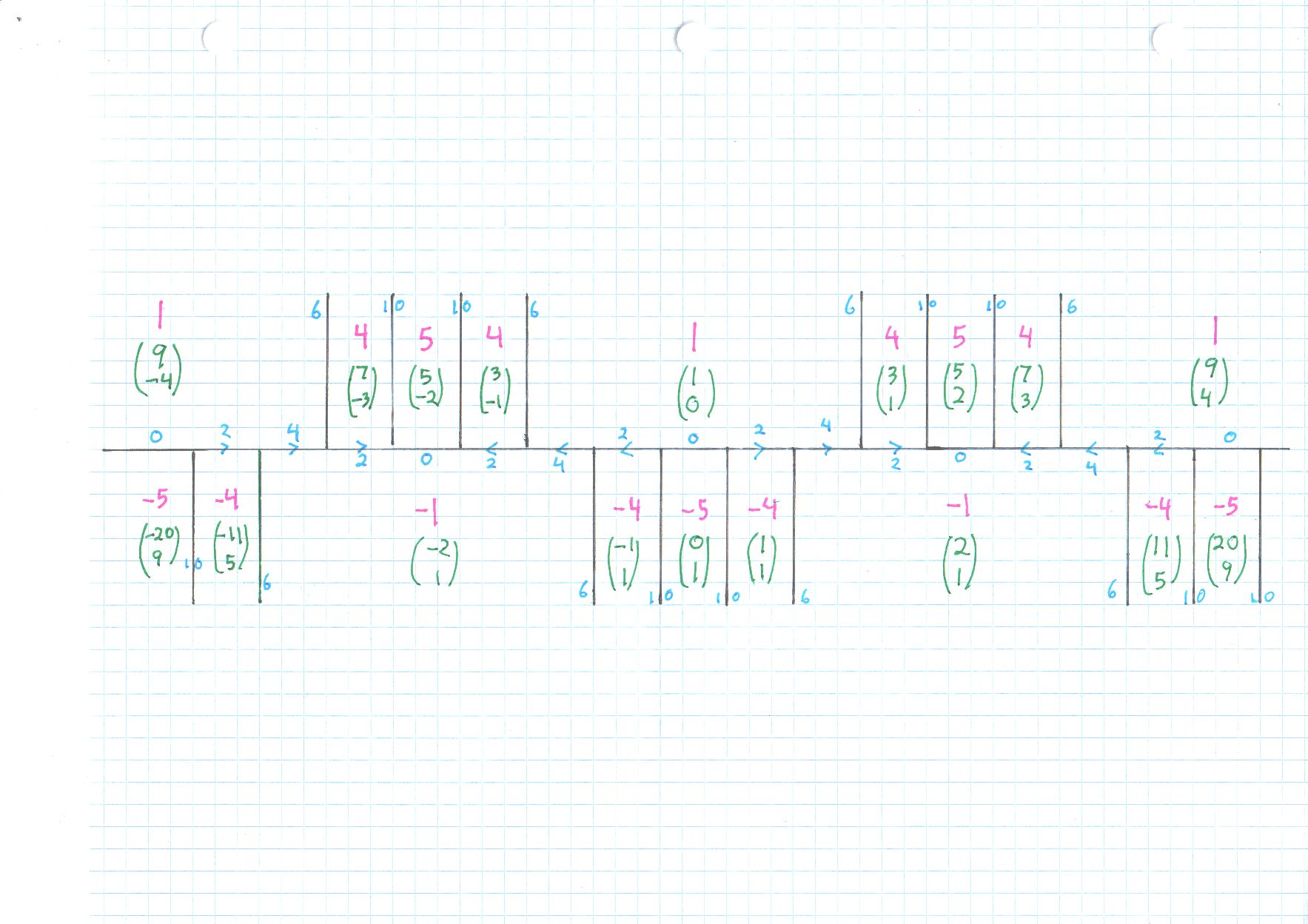
Conway's topograph diagrams seem to be part of this question. Most people have never actually drawn one, so here are some links with diagrams. i wrote a bunch of programs to eliminate errors, but still work. I gradually came to realize the importance, in order to save room, of making one drawing for the river and its immediate neighborhood, then making a separate digram for any of the "trees" leaving the river that are of particular interest. In the diagrams below, I am showing how to guarantee that we have all solutions to $x^2 - 5 y^2 = 44.$ I seem to have drawn four tree diagrams, so there were probably four $SL_2 \mathbb Z$ orbits of solutions.
Oh, one big point, that Conway did not emphasize enough, although he surely knew this. If, you include $(x,y)$ coordinates as I drew in dark green, the generator of the $SL_2 \mathbb Z$ automorphism group becomes explicitly visible as a pair of column vectors put side by side, in this case $$ \left( \begin{array}{cc} 9 & 20 \\ 4 & 9 \end{array} \right) $$ The inverse matrix of this generator is also visible, on the far left instead of the far right. This business of drawing in "coordinates" is emphasized in Stillwell's book, cleared up some confusion for me. Indeed, I revised Conway's very careful use of $\pm v$ at every turn to show exactly how the automorphism group acts on the coordinates.
see MARTY WEISSMAN about upcoming book, big chapter on topograph
http://www.maa.org/press/maa-reviews/the-sensual-quadratic-form (Conway)
http://www.springer.com/us/book/9780387955872 (Stillwell)
https://math.stackexchange.com/questions/228356/how-to-find-solutions-of-x2-3y2-2/228405#228405
https://math.stackexchange.com/questions/1599211/solutions-to-diophantine-equations/1600010#1600010
There is also the article by M.Uludag, A.Zeytin and M.Durmus here. Theoretically, an indefinite binary quadratic form can be seen as an infinite dessin d'enfant of Grothendieck. It's automorphism group becomes visible in this context.
You can have a look the application InfoMod here, in development by more or less the same team. The application is also available for android smart phones I guess.
