My answer to this question gives an example of a locally path connected (but non-semilocally simply connected) space $HA\subset\mathbb{R}^3$ called the Harmonic archipelago: draw the Hawaiian earring on a disk and between each hoop push the surface straight up to make a hill of height 1.
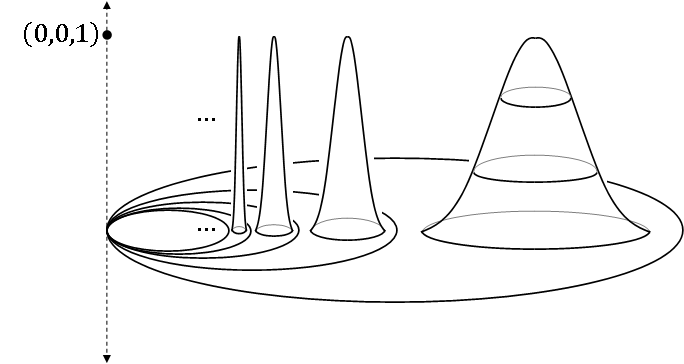
The fundamental group is uncountable but the only covering of $HA$ is itself so it is the unique object in its category of coverings. This weird covering behavior happens because you can deform any loop as close as you want to the basepoint (over finitely many hills) but cannot actually contract it all the way to the basepoint because the homotopy would have to take the loop over infinitely many of hills (contradicting compactness).
Topologies on the fundamental group detect this behavior. For instance the quotient topology from the loop space (the quasitopological fundamental group, $\pi_{1}^{qtop}(X)$) satisfies: If $p:Y\to X$ is a covering map, then $p_{\ast}:\pi_{1}^{qtop}(X)\to \pi_{1}^{qtop}(Y)$ is an open embedding of quasitopological groups. So if $\pi_{1}^{qtop}(X)$ is an indiscrete group there are no proper open subgroups so the only covering of $X$ is itself.
This works for all spaces including non-locally path connected ones. Really, I'd say it is easier to produce non-locally path connected examples. Here is a compact space $X$ with $\pi_{1}^{qtop}(X)\cong \mathbb{Z}_n$ indiscrete.
Let $T\subset \mathbb{R}^2$ be the closed topologists sine curve, $a\in T$ be the endpoint in the open path component and $b$ be a point in the other path component - say the origin. Now let
$$Y=\frac{T\times S^1}{T\times \{1\}\cup \{a\}\times S^1}$$
In other words, if $a$ is the basepoint of $T$, $Y$ is the reduced suspension $\Sigma T$.
You have $\pi_1(Y)\cong \mathbb{Z}$ where the loop $L:S^1\to Y$, $t\mapsto (b,t)$ represents a generator, but every neighborhood of $L$ contains a trivial loop so the quotient topology on $\pi_1(Y)$ is the indiscrete topology. If you attach a 2-cell to $Y$ using $L^n$ as an attaching map, you get a space $X$ where $\pi_{1}^{qtop}(X)\cong \mathbb{Z}_n$ is indiscrete. Both $Y$ and $X$ spaces have no nontrivial coverings.
Constructing locally path connected examples with countable indiscrete fundamental groups is much harder. In fact, I think it is an open question whether or not there exists a Peano continuum with finite, indiscrete fundamental group. For such spaces it is more efficient to talking about coverings via the "shape topology" and Spanier groups (the last section of this paper shows the shape topology consists precisely of the data of the category of coverings). Applied to your question: locally path connected $X$ has a categorical universal covering iff there is a minimal open subgroup in $\pi_1(X)$ with the shape topology. If the minimal open subgroup is the trivial subgroup you get back a classical universal covering and a discrete group.

