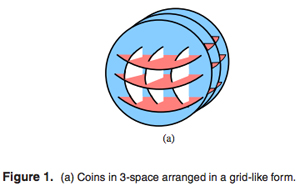
Consider $n$ affine copies of a compact cylinder, say $S^{1}\times [-3,3]$ with top and botom, sitting inside $\mathbb{R}^{3}$.
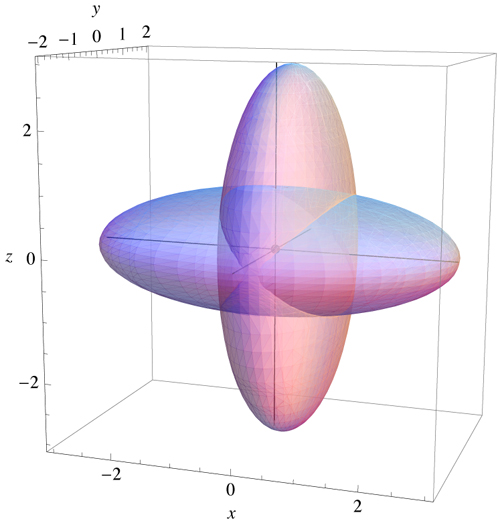
For each $n$ we may ask ourselves how to arrange the $n$ cylinders so that they divide 3-space into the maximum number of regions possible. For example, one cylinder divides 3-space in two regions. Two cylinders, if we intersect them so as to make a cross, divide space in 6 regions, but maybe more is possible.
If $n\ge 3$ things start to get complicated. For example, if $n=3$ we can obtain 14 regions if we start with two cylinders making a cross and then intersect the last cylinder diagonally, but I am not sure that this is the maximum. Perhaps more is possible?
I would like to know if there is a general formula giving us the maximum number of regions into which 3-space can be divided by cylinders.
Fundamental concepts like homology or the Euler characteristic may be of help if applied appropriately. Thus, if there is a general theory studying these kind of question I would appreciate any references on the matter.