As one may know, a dynamical system can be defined with a monoid or a group action on a set, usually a manifold or similar kind of space with extra structure, which is called the phase space or state space of the dynamical system. The monoid or group doing the acting is what I call the time space of the dynamical system, and is usually the naturals, integers, or reals. Often, one may require the evolution map to be continuous, differentiable, etc.
But has anyone studied a generalization in which we allow the time space to be something more general & exotic, a multidimensional space like $\mathbb{R}^n$, $\mathbb{C}$ (viewed as "2-dimensional" by considering it to be like $\mathbb{R}^2$), etc.? I'm especially curious about the case where the time space is $\mathbb{C}$ and the phase space is $\mathbb{C}^n$ or another complex manifold and the map is required to be holomorphic in both its arguments, as that holomorphism provides a natural linkage between the two dimensions that lets us think of the complex time as a single 2-dimensional time as opposed to two real times (any dynamical system with a timespace of $\mathbb{R}^n$ can be decomposed into a bunch of mutually-commutative evolution maps with timespaces of $\mathbb{R}$). My questions are:
Is it true that the only dynamical system with phase-space $\mathbb{C}$ and time-space $\mathbb{C}$ where the evolution map is required to be holomorphic in both its arguments (the time and point to evolve) is the linear one given by $$\phi^t(z) = e^{ut} z + K \frac{1 - e^{ut}}{1 - e^u}, K, u \in \mathbb{C}$$ ? I suspect so, because an injective entire function is linear (in the sense of a “linear equation” not necessarily a “linear map”), and $\phi^{-t}$ must be the inverse of $\phi^t$. Thus, $\phi^t$ must have the form $a(t) z + b(t)$ with $a: \mathbb{C} \rightarrow \mathbb{C}$, $b: \mathbb{C} \rightarrow \mathbb{C}$. Am I right?
Are there any interesting (e.g. with complicated, even chaotic behavior) dynamical systems of this kind on $\mathbb{C}^n$? On more sophisticated complex manifolds? (for the phase space, that is) If so, can you provide an example? Or does the holomorphism requirement essentially rule this out? EDIT: I provide one below.
There is something else here, an interesting observation I made. Consider the above complex-time, holomorphic dynamical system. We can investigate the two prime behaviors represented by the real and imaginary times. We'll just set u = 1, K = 0 for here.
In “real time”, the dynamics looks like an “explosion” in the plane: all points “blast” away from z = 0 at exponentially increasing velocity.
In “imaginary time”, the result is cyclic motion, swirling around $z = 0$ with constant angular velocity that depends only on the distance of the point from $z = 0$.
But if we trace the contours of these two evolutions, formed from different points on the plane, and then superimpose them, we have contours intersecting in what looks like contours from a contour graph of the image of the complex plane under the function $\exp(z)$! Conversely, we could say it looks like a countour plot of $\log(z)$ with a cut along a ray from $0$. So, somehow “naturally” related to the dynamical system $\phi^t(z) = \exp(t) z$ is the function $\exp(z)$ (or, perhaps, $\log(z)$).
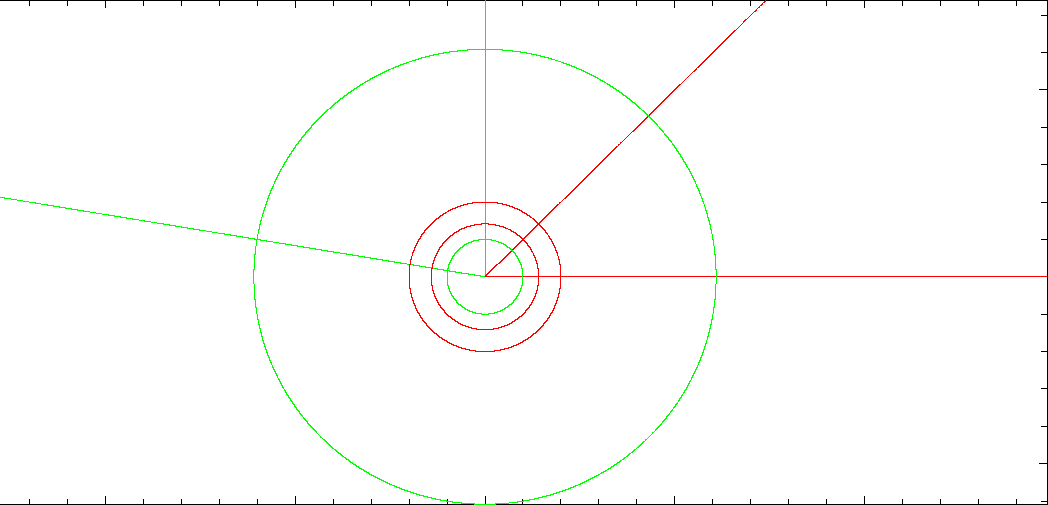
Note that my plotting facilities are unfortunately pretty limited, so I can't give really nice graphs with lots of contours, just a few selected ones taken from evolutions of various points in both real and imag times.
But we have another case. To see this, we must turn our attention away from a phase space given by the complex plane to one given by the Riemann sphere, $\hat{\mathbb{C}}$. In this case, we still have the dynamical system as above, but we have an additional class of dynamical systems given by the “Moebius transformations”, which include the above linear-function dynamical systems as a special case. One example is $$\phi^t(z) = \frac{(1 + e^{i\pi t}) z + (1 – e^{i\pi t})}{(1 – e^{i\pi t})z + (1 + e^{i\pi t})}$$. It is easy to check that this is indeed a Moebius transformation of the Riemann sphere. This map is holomorphic everywhere on the Riemann sphere. Note that for integer step t, the unit-step map is the reciprocal map.
Now we consider the contour lines of the real and imaginary evolution, as before. They look like this:
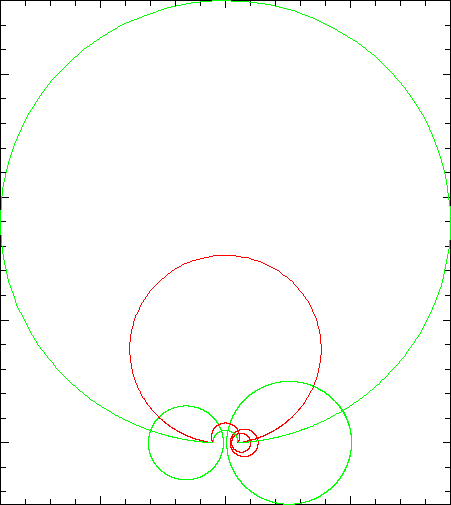
(Physics buffs may notice that the real evolution (concentric circles) reminds one of the lines of a magnetic field of a magnetic dipole (like a bar magnet), while the imaginary evolution (arcs joining at points) looks like the lines of an electric field of an electric dipole.)
Again, notice how the lines meet at right angles. It looks again like the image of the plane (or the Riemann sphere, perhaps?) under some function which may be holomorphic, though I'm not sure what that function is in this case. Is this the case? Is there such a function, with a special relation to this CTHDS in the same way as $\exp$ (or $\log$) is to the other?
But in any case, it appears that for a complex-time holomorphic dynamical system, or CTHDS, there exists an associated natural function. What is the significance of this function/map? How does it relate to the CTHDS? If you give me a CTHDS that I don't have a closed form for, can I find its natural map?
