Is this true of the frame bundle $\operatorname{Fr}(M)$?

Question

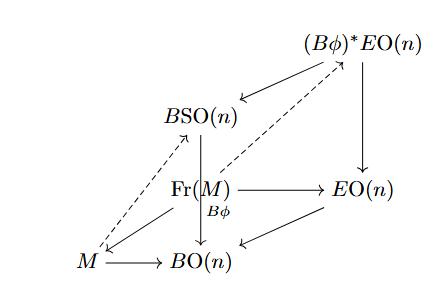
On an orientable (Riemannian) $n$-manifold $M$, with orthonormal frame bundle $\operatorname{Fr}(M)$, we have that the tangent bundle classifying map $\tau_M : M \to B{\operatorname O(n)}$ lifts to $B{\operatorname{SO}(n)}$ via the map $B\phi : B{\operatorname{SO}(n)} \to B{\operatorname O(n)}$ induced by the canonical group homomorphism $\phi : \operatorname{SO}(n) \to \operatorname O(n)$. This is expressed in the existence of the dotted arrow to the left in the diagram

My question is whether it makes sense to say that the dotted arrow from $\operatorname{Fr}(M)$ to $(B\phi)^*E{\operatorname O(n)}$ also exists such that the diagram still commutes. If so, what is it?

This question may be framed in more generality by replacing $\operatorname{SO}(n)$ with arbitrary $G$ and asking about $G$-structures on $M$. (I note that Dan Freed in his textbook presents a similar diagram in (1.38) for the definition of "flabby $G$-structures" except for using tangent bundles rather than associated frame bundles. However, I do not understand how exactly these flabby structures relate to the classical $G$-structures on $M$.)

Answer 1

There is a tiny confusion of language here. If you are talking about the orthonormal frame bundle you have a Riemannian manifold not just a manifold. The frame bundle has structure group $Gl_n(\mathbb{R})$.

In any case, if you concretely think of $BO(n)$ as limit of $n$-planes in $\mathbb{R}^N$ as $N \rightarrow \infty$ and $EO(n)$ as the limit of n-tuples of orthonormal vectors in $\mathbb{R}^N$ as $N \rightarrow \infty$. You can then think of $BSO(n)$ as n-planes together with an orientation realizing it as a double cover of $BO(n)$. Note that in this model $ESO(n)$ is exactly the same thing as $EO(n)$. The projection map to $BSO(n)$ in this model take an n-frame to it's span together with the orientation the ordered basis gives. The projection to $BO(n)$ forgets the orientation. Your pullback of by $B(\phi)$ of $EO(n)$ is the frame bundle of the tautological bundle. Since this bundle is oriented the frame bundle has two components $EO(n)_\pm$. Both are copies of $EO(n)$. $EO(n)_+$ is the one where the orientation of the frame agrees the orientation of plane it spans, $EO(n)_-$ is where it doesn't. $EO(n)_+$ is also $ESO(n)$. As such the lift you seek is already there and comes from this identification. Note that since $SO(n)$ is a (closed) subgroup of $O(n)$ if you have a contractible space on which $O(n)$ acts freely so does $SO(n)$ which gives a different take on why $EO(n)$ and $ESO(n)$ can be taken to the the same space.

Used above was the fact that in terms of frame bundles an orientation is simply a choice of component of the frame bundle, only the frames compatible with the orientation (assuming a connected base). The manifold is not orientable iff the frame bundle is connected. If you use a less geometric model the story might become fuzzier.

Answer 2

A lifting of the map $Fr(M)\to EO(n)$ along the map $(B\phi^\ast)EO(n)\to EO(n)$ always exists (up to homotopy, which I think is what you mean), simply because the space $EO(n)$ is contractible and the space $(B\phi^\ast)EO(n)$ is not empty.

(added:)

I suppose that by a lifting of $f:X\to B$ along $p:Y\to B$ you mean a map $\tilde f:X\to Y$ such that $p\circ \tilde f$ is homotopic to $f$. When $B$ is contractible, then any two maps from any space into $B$ are homotopic. So in that case a lifting simply means any map from $X$ to $Y$.

Or maybe you meant "lifting" in the strict sense that $p\circ \tilde f$ is equal to $f$. That is not reasonable to expect unless the map $B\phi$ is a fibration, which it is not if you literally mean that a functor $B$ is applied to a map of Lie groups $\phi$. On the other hand, maybe you mean for $B\phi$ to be mapping $BSO(n)$ to $BO(n)$ as a two-sheeted covering space, and therefore a fibration. In that case you are seeking to lift along a covering projection, and you can do so because a covering space of a (locally nice) contractible space is always a trivial covering space.