Boundedness of the preimage of sphere via homogeneous polynomials

Question

I am stuck with the following question. Any help or reference would be greatly appreciated.

Assume $F:\mathbb R^n\to \mathbb R^m$ to be a homogeneous polynomial of degree $d$, and assume $F$ to be surjective.

For every $\nu\in \mathbb S^{m-1}$ (the unit sphere in $\mathbb R^m$) let $x_\nu\in\mathbb R^n$ be such that $F(x_\nu)=\nu$

Is it true then that the set $\{|x_\nu|\mid \nu\in\mathbb S^{m-1}\}$ is uniformly bounded in $\mathbb R$? Alternatively is it true that we can always find a suitable solution $x_\nu$ to guarantee that the set above is bounded (maybe the whole preimage of the unit sphere is too big).

Edit: I think I have better specified the question. For $\nu\in \mathbb S^{m-1}$ the set $\{x\in \mathbb R^n\mid F(x)=\nu \}$ is not empty since the map $F$ is surjective, and there is an element of minimal norm. Thus we can choose $x_{\nu}$ such that $|x_{\nu}|=\min\{|x|\mid x \in F^{-1}(\nu)\}$.

Is it then true that there exists a constant $C>0$ such that $|x_{\nu}|<C$ uniformly as $\nu\in \mathbb S^{m-1}$?

Am I missing something obvious since I cannot write down a convincing proof for this fact?

Answer 1

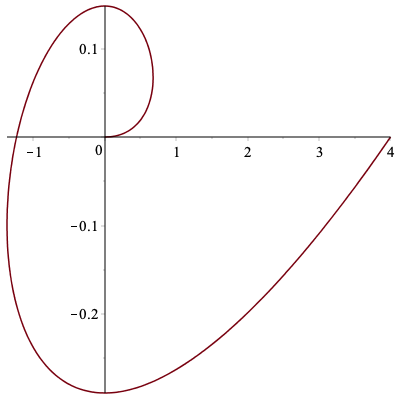
Here is a counterexample with $n=m=2$ and $d=8$.

Consider the polynomial $$p(t):=9t^2\big(t-\frac59\big)(t-1)$$and its derivative $$p'(t):=36t\big(t-\frac13\big)\big(t-\frac56\big).$$ Then, the $8$-th degree homogeneous polynomial map $F:\mathbb R^2\to\mathbb R^2$ $$F(x,y):=(x^2+y^2)^4\left[\matrix{p'\big(\frac{x^2}{x^2+y^2}\big)\cr \cr p\big(\frac{x^2}{x^2+y^2}\big)}\right]=\left[\matrix{ 2x^2(x^2+y^2)(2x^2-y^2)(x^2-5y^2)\cr\cr -x^4y^2(4x^2-5y^2)}\right]$$ is surjective and not open at the origin, or equivalently, $$\sup_{\|\nu\|=1}\min\big\{\|z\|:z\in f^{-1}(\nu) \big\}=+\infty.$$

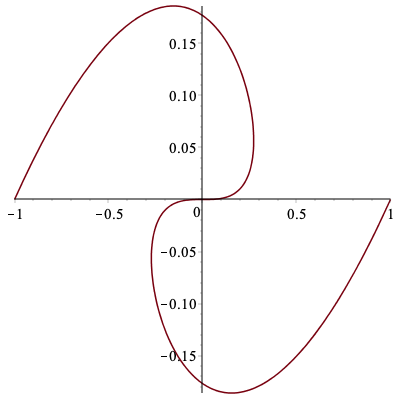
To prove the assertion, note that $F(\partial B(0,1))$ is a curve parametrized on the closed unit interval by $[0,1]\ni t\mapsto \gamma(t):= (p'(t),p(t))\in\Gamma.$ By homogeneity, $F(\mathbb R^2)=[0,+\infty)\cdot\Gamma$ and $F(B(0,r))=r^8[0,1]\cdot\Gamma$ for every $r>0$. We need therefore to show that $[0,+\infty)\cdot\Gamma=\mathbb R^2$, and that $[0,1]\cdot\Gamma$ is not a nbd of the origin. This brings us to the key point:

The curve $\Gamma$ has a polar representation of the form $p'(t)+ip(t)=\rho(t)e^{i\theta(t)}$ with $\rho:[0,1]\to[0,\infty)$, $\rho(0)=0$, $\rho(t)>0$ for all $0<t\le1$, and $\theta:[0,1]\to[0,2\pi]$ an increasing homeo.

In other words, $\Gamma$ emanates from the origin tangentially to the $x$-axis, makes a complete rotation around the origin, and ends exactly on the $x$-axis again. If you trust a Maple plot, the figure below may suffice, otherwise a formal proof follows.

Since the only multiple root of $p$ is $0$, $0$ is also the only zero of $\rho(t)=\sqrt{p(t)^2+p'^2(t)}$, which is therefore strictly positive for all $t>0$. From the sign of $p$ and $p'$, we have that $\gamma(t)$ is resp. in the $I,II,III,IV$ quadrant for $t$ between consecutive zeros of $pp'$, namely for $t$ in $I_1:=]0,\frac13[$, $I_2:=]\frac13,\frac59[$, $I_3:=]\frac59,\frac56[$, resp. $I_4:=]\frac56,1[$. It is therefore sufficient to observe that $\tan\theta(t)=\frac{p(t)}{p'(t)}$ is $o(1)$ for $t\to0$, which is clear, and that it is strictly increasing on each of the above mentioned intervals. To see it, note that e.g. that on each of these intervals $\log|p(t)|= 2\log t+ \log\big|t-\frac59\big|+\log|t-1|+\log9$ is a strictly concave function, hence $\big(\log|p(t)|\big)’=\frac{p’(t)}{p(t)}$ is strictly decreasing with constant sign, so $\frac{p(t)}{p’(t)}$ is strictly increasing.

rmk. The choice of the root $\frac59$, and the factor $9$, is not relevant but only due to aesthetic sake to get a simple factorization of $p'$, and a nice picture. Any other pair $p(t)=t^2(t-b)(t-1)$, and $q(t):=t(t-a)(t-c)$, with interlaced zeros $0<a<b<c<1$, would work as well.

Answer 2

It turns out that an example (of a surjective homogeneous polynomial map not open at the origin) in odd degree is even simpler.

Consider the $5$-th degree homogeneous polynomial map $G:\mathbb R^2\to\mathbb R^2$ $$G(x,y):= \left[\matrix{xy^4-x^5\cr \cr x^3y^2}\right].$$

• It is surjective. Since it is homogeneous of odd degree, is sufficient to show that the ratio of its coordinates, $\frac {xy^4-x^5}{ x^3y^2}$ of $G(x,y)$, covers all real-extended values. Indeed on the points $(1,t)$ and $(t,1)$ for all $t\in\mathbb R$, the ratio is $t^2-t^{-2}$ resp. $ t^{-2}-t^2$.

• It fails to satisfy the mentioned property (so it is not open at the origin). Let's take $\nu\in\partial B(0,1)\cap\mathbb R_+^2$, that is $\nu=(\cos\theta,\sin\theta)$ with $0<\theta<\frac\pi2$, and any $(x,y)\in G^{-1}(x,y)$. This means $$\cases {x(y^4-x^4)=\cos\theta>0\\ x^3y^2=\sin\theta>0}.$$ From this we see that $x>0$ and $xy^4>\cos\theta$, so $$\sin^2\theta=x^6y^4=x^5(xy^4)>x^5\cos\theta$$ and $x^5<\frac{\sin^2\theta}{\cos\theta}.$ Then $y^2=x^{-3}\sin\theta > \big(\frac{\sin^2\theta}{\cos\theta}\big)^{-\frac35}\sin\theta=\sin^{-\frac65}\theta\cos^{\frac35}\theta$ and $|y|> \tan^{\frac1{10}}\theta\cdot\cos\theta,$ so the minimum norm of elements of $G^{-1}(\nu)$ is not uniformly bounded for $\nu\in\partial B(0,1)$. $$*$$ For comparison with the map $F$ of the other answer, here is a picture of the $G$-image of the unit circle, which one can parametrise by $[-1,1]\ni t \to (t-2t^3 , t^3-t^5 )$.