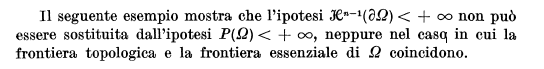Given a set of finite perimeter $\Omega$ s.t. $\partial ^* \Omega =\partial \Omega$, it's not true that $P(\Omega)= \mathcal{H}^{n-1} (\Omega)$

Question

In the article "Funzioni BV e tracce" by Anzellotti and Giaquinta (MR555952, Zbl 0432.46031), at page 6 you can read (assume $\Omega \subset \mathbb{R}^n$ open): "The following example shows that the hypothesis $\mathcal{H}^{n-1} (\partial \Omega) < + \infty$ cannot be replaced by the hypothesis $P(\Omega)< + \infty$, even if the the topological frontier and the reduced boundary of $\Omega$ coincide."

Now, I know that in general topological boundary and reduced boundary have nothing to do with each other, but I thought that if you have a set of finite perimeter, by De Giorgi's structure theorem they were essentially the same, in the sense that you can work equivalently with the perimeter or the Hausdorff measure (with the reduced boundary). Namely if you have a set of finite perimeter $E \subset \mathbb{R}^n$, by the structure theorem:

$$ D 1_E = \nu_E \mathcal{H}^{n-1} |_{\partial^* \Omega} ,$$

but if $\partial E= \partial^* E$, then:

$$+ \infty > P(E) = |D1_E|(\mathbb{R}^n)= \mathcal{H}^{n-1} (\partial ^* E)= \mathcal{H}^{n-1} (\partial E).$$

What's the caveat here that I am not seeing?

Answer 1

I will use notation from the book:

L. C. Evans, R. F. Gariepy, Measure theory and fine properties of functions, Textbooks in Mathematics. Boca Raton, FL: CRC Press (ISBN 978-1-4822-4238-6/hbk), 309 p. (2015), MR3409135, Zbl 1310.28001.

All the results that I am using below are carefully proved in Chapter 5 of that book.

Let $E\subset\mathbb{R}^n$ be measurable. The measure theoretic boundary $\partial_* E$ consists of points $x$ such that $$ \limsup_{r\to 0} \frac{|B(x,r)\cap E|}{r^n}>0 \quad \textit{ and } \quad \limsup_{r\to 0} \frac{|B(x,r)\setminus E|}{r^n}>0. $$ For example, if $E=\Omega$ is open and $x\in\partial\Omega$ is a vertex of a cusp, then $x\in\partial\Omega\setminus \partial_*\Omega$. Thus in general $\partial\Omega$ is a larger set and one can construct a bounded open set with a nasty boundary full of narrow parts so that $$ \mathcal{H}^{n-1}(\partial_*\Omega)<\infty \quad \textit{ but } \quad \mathcal{H}^{n-1}(\partial\Omega)=\infty.\label{1}\tag{$*$} $$ While I haven't read the example of Anzellotti and Giaquinta carefully, I believe this is what they have.

If $E$ is bounded measurable and $\mathcal{H}^{n-1}(\partial_*E)<\infty$, then $E$ has finite perimeter and the reduced boundary $\partial^*E$ satisfies $$ \partial^*E\subset\partial_*E, \quad \mathcal{H}^{n-1}(\partial_*E\setminus\partial^*E)=0 \quad P(E)=\mathcal{H}^{n-1}(\partial^*E)=\mathcal{H}^{n-1}(\partial_*E). $$ Here $P(E)$ is the perimeter. Now let's look again at the example of bounded $E=\Omega$ satisfying \eqref{1}. Since $\mathcal{H}^{n-1}(\partial_*\Omega)<\infty$, the set $\Omega$ has finite perimeter, and we have $$ P(\Omega)=\mathcal{H}^{n-1}(\partial_*\Omega)<\infty \quad \textit{ but } \quad \mathcal{H}^{n-1}(\partial\Omega)=\infty. \label{2}\tag{$**$} $$ If the reduced boundary coincides with the topological boundary and the set has finite perimeter, then we clearly have $$ \partial\Omega=\partial^*\Omega\subset\partial_*\Omega\subset\partial\Omega$$ and hence all sets are equal. Thus $P(\Omega)=\mathcal{H}^{n-1}(\partial_*\Omega)=\mathcal{H}^{n-1}(\partial\Omega)$. I think that the last part of the comment they made:

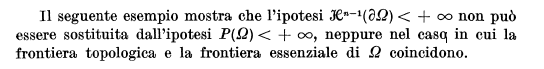
Not even in the case in which the topological boundary and the reduced boundary of $\Omega$ coincide.

is not correct, but the claim that it is possible to have \eqref{2} is true.