I'm studying compound poisson processes and in "Levy processes and infinitely divisible distributions" there is this theorem (4.3) :
To proof that it is a Levy process we have to show that:
But in this book the points (4) and (5) are not proven. How can I prove them?
$\newcommand{\N}{\mathbb N}\newcommand\ep\varepsilon$Apparently, the book "Levy processes and infinitely divisible distributions" you mentioned is the one by Sato.
According to the construction in Theorem 3.2 in this book, a Poisson process $(N_t)_{t\ge0}$ is defined by the condition \begin{equation} N_t=n\iff W_n\le t<W_{n+1}, \end{equation} where $t\in[0,\infty)$, $n\in\N_0:=\{0,1\dots\}$, $W_n:=\sum_{1\le k\le n}T_k$ (with $W_0=0$), and the $T_k$'s are iid random variables (r.v.'s) each with the exponential distribution with some mean $c\in(0,\infty)$.
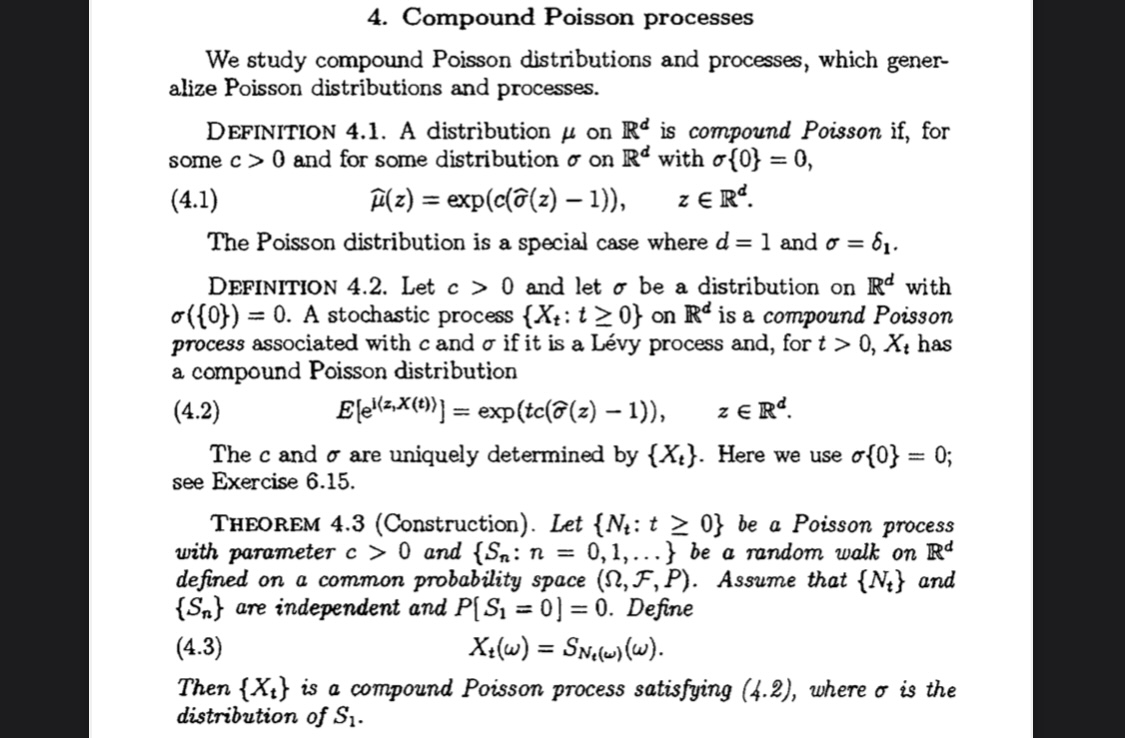
As seen from the image in your post, according to formula (4.3) in Sato's book, \begin{equation} X_t=S_{N_t}. \end{equation} So, \begin{equation} X_t=S_n\text{ if }W_n\le t<W_{n+1}, \end{equation} Therefore and because $W_n\to\infty$ almost surely (a.s.) as $n\to\infty$, it immediately follows that a.s. $(X_t)$ is right-continuous in $t\ge0$ and has finite left limits for all $t>0$, so that property (5) in Definition (1.6) in the book holds.
Moreover, for any real $s$ and $t$ such that $0\le s<t$ and any real $\ep>0$, \begin{equation} P(|X_t-X_s|>\ep)\le P(X_t\ne X_s)\le P(\exists n\in\N_0\ s<W_n\le t) \\ \le \sum_{n=0}^\infty P(s<W_n\le t) =\sum_{n=1}^\infty P(s<W_n\le t). \end{equation} Note that $W_n$ has the gamma distribution with parameters $n$ and $c$. So, \begin{equation} P(|X_t-X_s|>\ep)\le \sum_{n=1}^\infty\int_s^t du\,\frac{u^{n-1}}{(n-1)! c^{n-1}}\,e^{-u/c} \\ =\int_s^t du\,\sum_{n=1}^\infty\frac{u^{n-1}}{(n-1)! c^{n-1}}\,e^{-u/c} = \int_s^t du=t-s. \end{equation} Now the stochastic continuity of $(X_t)$ (which is property (4) in Definition (1.6) in the book) immediately follows. $\quad\Box$
