There are probably many ways to think about this. Here is one. I fear it might not be terribly specific to your situation.
You have a $\Theta_2$-set, i.e. a presheaf on Joyal's category $\Theta_2$. There is a nerve functor $\nu_2 : 2Cat \to \widehat{\Theta_2}= Fun(\Theta_2^{op},Set)$ (coming from the fact that $\Theta_2$ is a full subcategory of $2Cat$), but since you're missing horizontal composites, your $\Theta_2$-set is not in the image of $\nu_2$.
However, the image of $\nu_2$ is a reflective subcategory of $\widehat{\Theta}$, and the localization is easy to describe: it consists of those objects $X$ which are right orthogonal to the generalized spine inclusions, which surprisingly I'm having difficulty locating a reference for at the moment. This is a "baby" version of the Theta-space or Theta-set presentations of $(\infty,2)$-categories, and a generalization of the fact that the essential image of the usual nerve functor from 1-categories to simplicial sets is characterized by the unique-inner-horn-filler property (see 3.8 here) [1].
One way to say it is that that we localize $\widehat{\Theta_2}$ at the smallest set of maps $S$ with the following two properties:
Note that each object of $\Theta$ comes with a natural bipointing by its "first" and "last" objects $\bot$ and $\top$. If $\theta,\zeta \in \Theta$, then
$$\nu_2(\theta) \vee \nu_2(\zeta) \to \nu_2(\theta \vee \zeta)$$
is in $S$. Here the "wedge sum" $A \vee B$ is the pushout $A \cup_\ast B$ where on $A$ we use the "last" point $\top$ and on $B$ we use the "first" point $\bot$ -- the map above takes the wedge sum in two different categories, and the map is the resulting comparison map, which can be thought of as "filling in the horizontal composites".
If $A \to B$ is in $S \cap \Theta_1$, then
$$\Sigma(A) \to \Sigma(B)$$
is in $S$. Here, we observe that the usual suspension functor $\Sigma : 1Cat \to 2Cat$ (which carries $C$ to the 2-category $\Sigma C$ with two objects $\{0,1\}$ with $Hom(0,0) = Hom(1,1) = \ast, Hom(1,0) = \emptyset, Hom(0,1) = C$) carries $\Theta_1$ (which is a full subcategory of $\Theta_2$ and is isomorphic to $\Delta$) to $\Theta_2$. We left Kan extend to obtain a functor which we also call $\Sigma: \widehat{\Theta_1} \to \widehat{\Theta_2}$, and that's what we're applying above. The above map $A \to B$ can be thought of as "filling in the vertical composites".
In order to reflect your $\Theta$-set $X$ into the image of $\nu$, you do what one can always do with such a localization -- wherever you see a map $A \to X$ with $A \to B \in S$, with no extension to $B \to X$, you take a pushout in $\widehat{\Theta_2}$. Whenever you see a map $A \to X$ with two distinct extensions $B \to X$, collapse them together. Do this repeatedly until you can't anymore. This can be seen as a case of the small object argument.
The fact that you already have all vertical composites means that at least initially you only have to worry about maps in $S$ of the form (1) above. But the property of having all vertical composites might be lost as soon as you start doing the iterative gluing described above. So pretty soon you will have to consider maps of the form (2) as well.
[1] Well, the "spine-like" busieness here doesn't directly generalize inner horns -- for that see here. The directly analogous statement for 1-categories is as follows. We have $\Theta_1 = \Delta$. There is a nerve functor $\nu_1 = \Delta[-] : 1Cat \to \widehat{\Theta_1} = sSet$, which is fully faithful, and whose essential image is characterized as the right orthogonal complement of the following set of morphisms:
$$ \{\Delta[m] \vee \Delta[n] \to \Delta[m+n-1] \mid [m],[n] \in \Delta\}$$
Here $\Delta[m] \vee \Delta[n]$ is obtained from $\Delta[m]$ and $\Delta[n]$ by gluing the last vertex of $\Delta[m]$ to the first vertex of $\Delta[n]$. The map $\Delta[m] \vee \Delta[n] \to \Delta[m+n-1]$ is the one which on vertices sends $i \in [m] \mapsto i$ and $j \in [n] \mapsto m + j$. Note that as categories, $[m+n-1]$ is the pushout of $[m]$ and $[n]$ over a point in this way. The above map of simplicial sets is "filling in the missing composites".
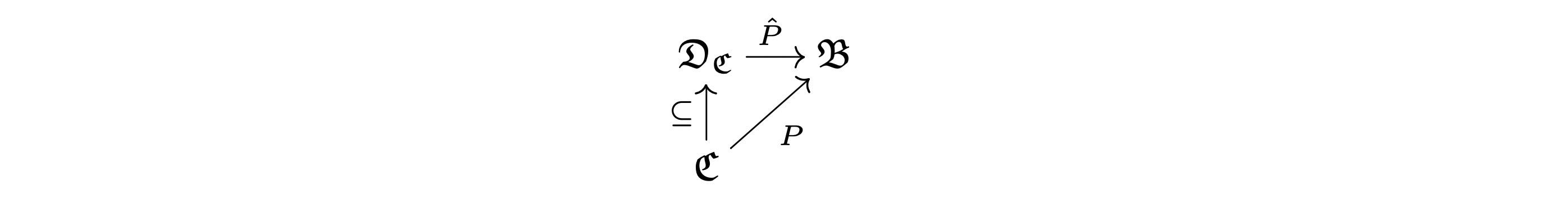 commutes, so 'almost pseudofunctors' out of $\mathfrak{C}$ uniquely determine pseudofunctors out of $\mathfrak{D}_\mathfrak{C}$.
commutes, so 'almost pseudofunctors' out of $\mathfrak{C}$ uniquely determine pseudofunctors out of $\mathfrak{D}_\mathfrak{C}$.