It is standard in category theory to study things 'from the top down' -- to study structured sets we use categories, to study structured categories we use bicategories, to study structured bicategories we use Gray-categories, and to study all of them at once we can use $\infty$-categories.
Unless I'm mistaken, some of Grothendieck's initial work on fibered categories was implicitly using bicategorical ideas (indexed categories) without the bicategorical vernacular, and the development of $2$-category theory allowed us to more succinctly express the ideas in play.
This has obviously been fruitful, but it seems counterintuitive to study simpler things using more complicated things. I can write down the definition of a set in a few seconds (primitive), the definition of a category in about a minute, the definition of a bicategory takes maybe a few minutes if rushed, and a tricategory with all of its coherence pasting diagrams explicitly written out would take 27 pages (if I remember correctly, I'm moving and the GPS book with the definition is still in the old house). Ideally, I would like to be able to study the more complicated things using conceptually simpler ones.
Has there been any work done attempting to seriously study higher categorical notions using lower categorical ones?
For example, we can consider the $1$-category of pseudonatural transformations and modifications (in full generality or between two fixed pseudofunctors) and study it using the machinery of $1$-category theory, perhaps gaining insight into pseudofunctor $2$-categories. We could also study the category of bicategories and pseudofunctors. These examples both require prior knowledge of the definitions involved in the higher stages of the categorical hierarchy though, which is not ideal.
There are also some negative results in this direction; for example, we can't form a category of tricategories and trihomomorphisms since composition of trihomomorphisms isn't associative on the nose; the best we can hope for is a bicategory (see page $4$ of the linked Garner/Gurski paper). This obstacle seems to imply that an approach based on this method would be limited to using levels just below the one we want to study.
Another type of example would be attempting to study the ingredients of higher categories at lower levels. For example, the definition of a bicategory uses categories, functors and natural transformations. While I'm not aware of a way to compile all three of these into one structure without using bicategories, we can collect the top two levels into a category ${\bf Fun}$ of functors and natural transformations -- functor categories between fixed categories $\mathcal{C}$ and $\mathcal{D}$ can be obtained as the full subcategory of ${\bf Fun}$ on functors whose domain is $\mathcal{C}$ and whose codomain is $\mathcal{D}$.
While the composition of functors and Godement product of natural transformations together don't form any kind of named structure I'm aware of on ${\bf Fun}$, if we restrict out attention to endofunctor categories $\mathcal{C}^\mathcal{C}$ for a fixed category $\mathcal{C}$ then composition and Godement products constitute a tensor product, turning $\mathcal{C}^\mathcal{C}$ into a strict monoidal category; whatever structure composition constitutes on ${\bf Fun}$, it's one one that restricts to a strict tensor product on certain 'well-behaved' full subcategories.
This can be seen as a structure 'endowed' on the endofunctor category by virtue of the fact that $\mathfrak{Cat}$ is a strict $2$-category, but we could also in theory make the observations about $\mathcal{C}^\mathcal{C}$ and ${\bf Fun}$ first and study them, gaining insight into horizontal composition of $2$-cells at the $2$-categorical level using $1$-categorical machinery. This is just a rough example of what I'm looking for, but it's the best I've come up with after a week or so thinking about it. Any assistance is appreciated.
Linked paper: arXiv:0711.1761v2 [math.CT]
EDIT: For another example in the second vein, we have the following lemma about sets and functions that essentially yields the intuituion for the Yoneda lemma, and can be used to prove it.
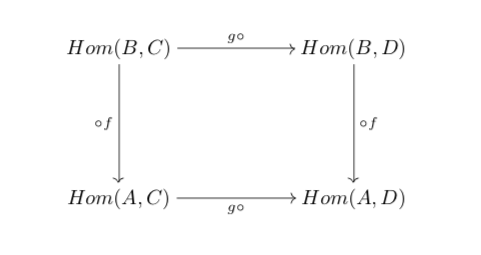
Lemma For sets $X,Y$, let $Hom(X,Y)$ denote the set of all functions from $X$ to $Y$. For any two functions $f:A\to B$ and $g:C\to D$, the following diagram commutes
where $g\circ$ and $\circ f$ denote postcomposition with $g$ and precomposition with $f$, respectively.
We can thusly study a central piece of $1$-category theory using sets and functions ($0$-category theory).