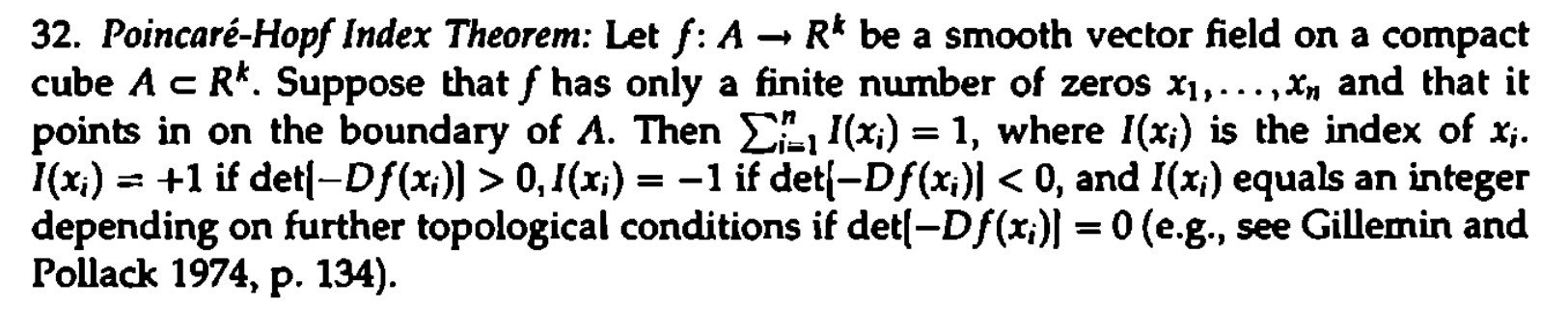9
$\begingroup$

As we know, the square $S=[0,1]\times[0,1]$ is not a manifold with boundary. Instead, it's a manifold with corners. For a tangent vector field on a compact manifold with boundary, we have the Poincaré–Hopf index theorem.

Then here is my question: is there a similar index theorem for manifolds with corners (e.g., the square, the cube) which relates the indices of a tangent vector field to the Euler characteristics of the manifold? If affirmative, what is the statement of this theorem?

Btw, I find an economics textbook (Vives, X., 1999. Oligopoly pricing: old ideas and new tools. MIT Press) which states that the Poincaré–Hopf theorem can apply to a compact cube (a manifold with corners). The statement (on page 362) is as below:

p. 362 of Vives, Oligopology pricing

This "version" is frequently used in economics papers. Is it correct?

Improve this question
$\endgroup$
8
  • $\begingroup$ If what you seek exists, you should consider an object that encodes the $C^1$-difference between a closed disc and a closed square: at the topological level both objects coincide. Probably the number of corner / the collection of their angles is relevant. $\endgroup$
    – Loïc Teyssier
    Commented Feb 6, 2022 at 12:07
  • 1
    $\begingroup$ The obvious thing that discriminates both situations from the point of view of vector fields is whether or not a singularity sits in a corner. Then, you'll have to define what is the index of these "quarter"-singularities (which is OK if the boundary of the manifold is assumed invariant by the vector field). $\endgroup$
    – Loïc Teyssier
    Commented Feb 6, 2022 at 12:09
  • $\begingroup$ @LoïcTeyssier Thanks for your comments. I have a question: Is the simplex (e.g., $S = \{ ({x_1},{x_2},{x_3}):\sum\limits_{i = 1}^3 {{x_i}} = 1,{\rm{ }}{x_i} \ge 0\}$) a manifold with corners? It has three angles. $\endgroup$
    – Ya He
    Commented Feb 6, 2022 at 12:26
  • $\begingroup$ @LoïcTeyssier I think quarter-singularity might be a misleading term here. Corners need not be right angle, so any number of them could join up to form a full singularity. But I guess the definition of degree as signed relative area of $S^{n-1}$ covered by the map would work safely for any angle. $\endgroup$
    – mlk
    Commented Feb 6, 2022 at 13:31
  • 2
    $\begingroup$ @YaHe: the simplex is a manifold with corners. In particular, the simplex you have written down is an equilateral triangle. Note that angles at corners less than 180 can be altered by change of variables, to any other angle less than 180. $\endgroup$
    – Ben McKay
    Commented Feb 6, 2022 at 14:42

1 Answer 1

Reset to default
11
$\begingroup$

With regards to the updated question: Note that the quoted statement is that the vector field points inward at the boundary. In particular this means that there are no singularities at the corners (nor at the flat parts of the boundary). Thus you can simply replace the manifold by a slightly smaller one, for which the corners are "rounded off". This then is a manifold with boundary, to which the usual Poincaré-Hopf theorem applies.

That being said, as discussed in the comments, the situation gets a bit more interesting when there are singularities at the boundary. There, the theorem still holds, provided the notion of index is correctly defined for those singularities. I think for this, one can simply copy the usual proof of the Poincaré-Hopf theorem which cuts out small balls around the singularities and then simply define the index as whatever is needed to make this work.

Toying around with the Gauss-map, I would expect something like $$ \operatorname{ind}(x_0) = \lim_{\epsilon \to 0} \frac{1}{|S^{n-1}|} \int_{S\cap \partial B_\epsilon(x_0)} \left(\frac{u}{|u|}\right)^* \omega_{S^{n-1}}$$ but for corners there might be situations where this limit is not entirely well defined.

Improve this answer
$\endgroup$
9
  • $\begingroup$ Thanks for your answer. For manifolds with boundary, the PH theorem assumes that the inner product of the Gauss map and the vector field is always positive at the boundary. For manifold with corners, let's still keep the assumption that there is no singularities at the corners (nor at the flat parts of the boundary). But the Gauss map does not exist at the corners. In order to make PH theorem still hold, how should the vector field behave at the corners? Can you specify in detail the conditions at the corners? $\endgroup$
    – Ya He
    Commented Feb 6, 2022 at 15:06
  • 1
    $\begingroup$ @YaHe Ultimatively you need to be able to smooth the corners without violating this condition, so it needs to point "outwards" in some sense. But this you get automatically because it is already pointing outwards for all the flat pieces of the boundary near the corner. $\endgroup$
    – mlk
    Commented Feb 6, 2022 at 16:06
  • 1
    $\begingroup$ @mlk: technically having the v.f. pointing inwards along the boundary away from the corner points is compatible with the v.f. being precisely zero on the corner points. I feel that one has to interpret "pointing inward" to imply "non-vanshing, and continuous on the boundary, and positive inner product against Gauss map where it is well-defined." (Perhaps obvious to you and me, but less so to someone less familiar.) $\endgroup$
    – Willie Wong
    Commented Feb 6, 2022 at 17:13
  • 3
    $\begingroup$ @YaHe: You don't have to define inward pointing from the Gauss map. For example, the existence of a curve $\gamma: [0,1)\to A$ such that $\gamma(0)$ is the boundary point, $\gamma'(0)$ equals to vector field at that point, and $\gamma|_{(0,1)}$ sits in the interior of $A$ is another viable definition. $\endgroup$
    – Willie Wong
    Commented Feb 6, 2022 at 17:22
  • 1
    $\begingroup$ @YaHe Your specified condition does not rule out the possibility that the vector field vanishes at one (or multiple) of the corners. For an explicit example: Let your domain be $\Omega:= \{(x,y)\in \mathbb{R}^2: |y| \leq \sin(x), x\in [0,\pi]\}$ and $V = (x,y)$. It is not too hard to see that away from the two corners the vector field $V$ points outward through the boundary. $\endgroup$
    – Willie Wong
    Commented Feb 7, 2022 at 5:25

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .