0
$\begingroup$

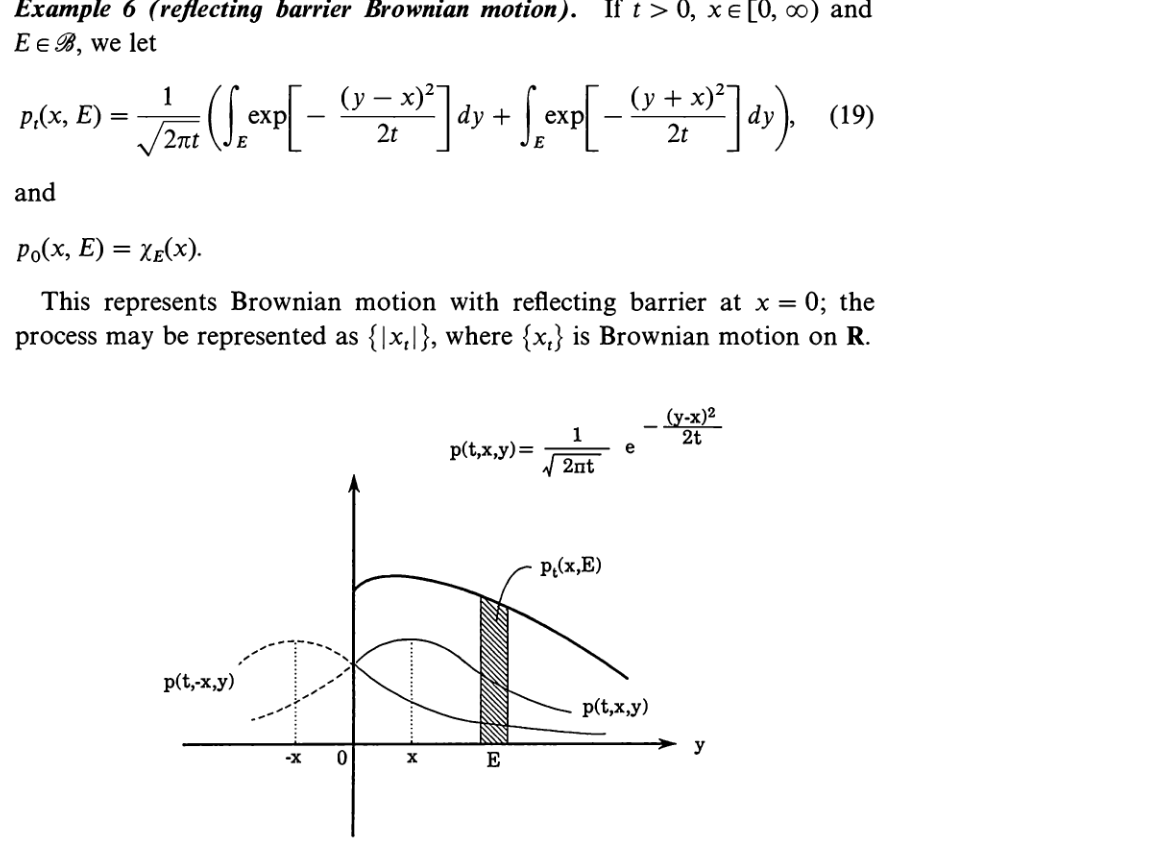
enter image description here

We can see from the above picture the transition density of reflecting Browninan motion is given by (19). As we know, the first part ($2p(t,x,y)$) is the transition density of a Brownian motion (from $x$ to set $y$) and the second part($2p(t,-x,y)$) also is the transition density of a Brownian motion (from $-x$ to set $y$).

  1. My first question is the figure. $p_t(x,E)$ should equal $p(t,x,y)$ + $p(t,-x,y)$, but why in the figure the area of the shadow denoting $p_t(x,E)$ looks larger than the sum of those denoting $p(t,x,y)$ and $p(t,-x,y)$?
  2. Why does the transition density (19) represent the rerflected Brownian motion at barrier 0? The connection among them is not so obvious. In particular, how to explain these two parts? why the sum of these two transition densities represent a modulus Brownian motion, where can I see it?
Improve this question
$\endgroup$

1 Answer 1

Reset to default
1
$\begingroup$

  1. The solid line in the figure that represents $p(t;-x,y)+p(t;x,y)$ looks qualitatively OK but quantitatively wrong.

  2. Let $y=|x_0|$ and $E\subset[0,\infty)\,.$ Then $p_t(y;E)=P(|x_t|\in E)=P(x_t\in E)+P(-x_t\in E)=\int_Ep(t;x,y)\,dx+\int_Ep(t;-x,y)\,dx\,.$

Improve this answer
$\endgroup$
2
  • $\begingroup$ In fact, your answer for the second question is not clear. Why you see (19), you can conncet with $|B_t|$? How two explain (19)? $\endgroup$
    – Ailiy Evan
    Commented Aug 16, 2021 at 13:57
  • $\begingroup$ Because $p(t;x,y)=\frac{1}{\sqrt{2\pi t}}\exp[-\frac{(y-x)^2}{2t}]$ the equation I wrote is (19). Secondly, the unreflected BM is $x_t$ and the reflected one is $|x_t|\,.$ $\endgroup$
    – Kurt G.
    Commented Aug 16, 2021 at 14:17

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .