8
$\begingroup$

Consider the following map \begin{align*} T \colon \mathbb{R}\times\mathbb{S}^1 \to & \mathbb{R}\times\mathbb{S}^1 \\ (x,\theta) \mapsto & \left(\frac{x}{4}+ \sin^2\left(\pi\left(\theta+\frac{x}{2}\right)\right)+0.01, \theta+\frac{3x}{4}+\sin^2\left(\pi\left(\theta+\frac{x}{2}\right)\right)+0.01\right) \end{align*} I have been doing some numerical simulations and it seems this system presents a chaotic attractor. Here there is an approximation of the attractor. The colored points are the fixed points of the map. 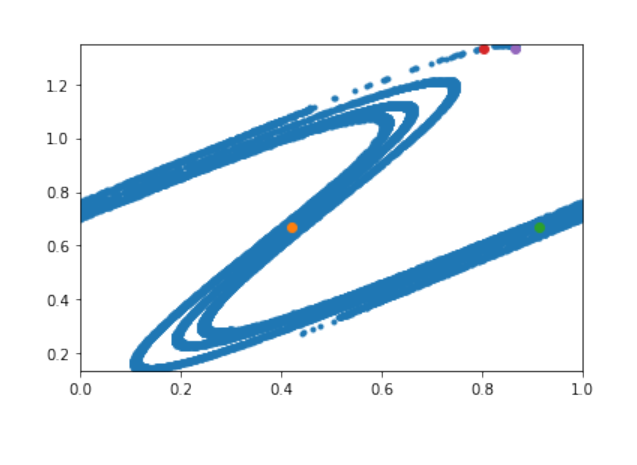enter image description here According by the following result that can be found in the Katok and Hasselblat book enter image description here the chaos in this system might be generated by a transverse intersection of stable and unstable manifold of the orange saddle point.

My Question: How do I check analytically that there is this intersection or how do I prove this map has chaotic behaviour?

Could anyone help me ?

Improve this question
$\endgroup$
1
  • 2
    $\begingroup$ I'm afraid I can't say anything useful about the particular system you ask about. I believe it to be the case that even for the much simpler system $f(x)=cx(1-x)$, which for $0\le c\le4$ is a map from $[0,1]$ to itself, there are a great many values of $c$ for which it is believed that the map is chaotic, but precious few for which there is a rigorous proof. $\endgroup$
    – Gerry Myerson
    Commented Jul 13, 2021 at 23:31

1 Answer 1

Reset to default
2
$\begingroup$

For specific systems at specific parameters, it can often be infeasible to give a pen-and-paper proof of chaos. However there is a rich literature of using computer-assisted-proofs based on interval arithmetic to demonstrate chaos, see for example

Mischaikow, K., & Mrozek, M. (1995). Chaos in the Lorenz equations: a computer-assisted proof. Bulletin of the American Mathematical Society, 32(1), 66-72.

The dynamical system you have looks similar to the Chirikov standard map. The following paper seems quite relevant to the problem you are studying. To quote from their abstract, they "show how interval analysis can be used to calculate rigorously valid enclosures of transversal homoclinic points in discrete dynamical systems."

Neumaier, A., & Rage, T. (1993). Rigorous chaos verification in discrete dynamical systems. Physica D: Nonlinear Phenomena, 67(4), 327-346.

In the intervening three decades there has been much progress and interest in developing computer-assisted-proofs of chaos in dynamical systems. For a more recent resource, I would recommend taking a look at the CAPD library:

Kapela, T., Mrozek, M., Wilczak, D., & Zgliczyński, P. (2021). CAPD:: DynSys: a flexible C++ toolbox for rigorous numerical analysis of dynamical systems. Communications in Nonlinear Science and Numerical Simulation, 101, 105578.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .