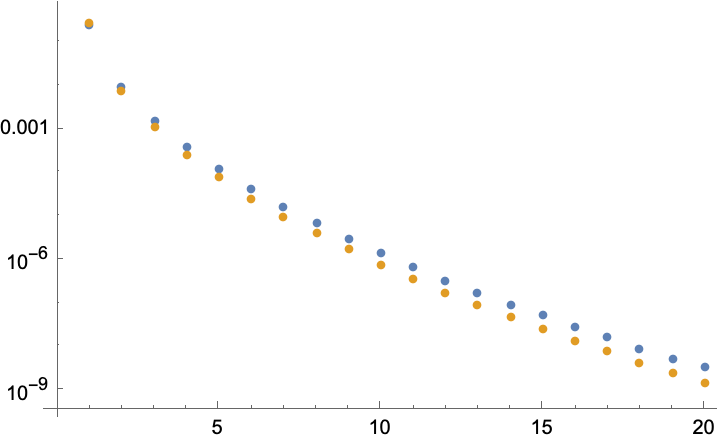
I would like to know the asymptotics of the following sequences of integrals: $$ I_n = \displaystyle { \int _0 ^{+ \infty} \dfrac{t^n}{(t + i)^{n + 1}} \cdot e^{-t} \ dt } $$
Using a deformation of path with Cauchy theorem, I've shown that $$ I_n = \displaystyle { \int _{i \mathbb{R}^+} \dfrac{t^n}{(t + i)^{n + 1}} \cdot e^{-t} \ dt = \int _0 ^{+ \infty} \dfrac{t^n}{(t + 1)^{n + 1}} \cdot e^{-it} \ dt } $$
I therefore have tried using Laplace method, but I have been unable to conclude anything...
Moreover, I didn't manage to use computer software to have some reliable numeric values, because of the oscilating function $t \mapsto e^{-it}$. So, I haven't got any conjecture...