Questions on analytic representations of the Kronecker delta function $\delta(x-1)$ and the Moebius function $\mu(n)$

Question

This question is related to analytic formulas for $a(n)$ where $f_a(x)$ and $F_a(s)$ defined in formulas (1) and (2) below are the summatory function and Dirichlet series associated with $a(n)$.

---

$$f_a(x)=\sum\limits_{n=1}^x a(n)\tag{1}$$

$$F_a(s)=s\int\limits_0^\infty f_a(x)\,x^{-s-1}\,dx=\sum\limits_{n=1}^\infty a(n)\ n^{-s}\tag{2}$$

---

In the remainder of this question $\tilde{a}(x)$ is used to refer to an analytic representation of the arithmetic function $a(n)$. The analytic representation $\tilde{a}(x)$ typically converges to $a(n)$ when $|x|=n\in\mathbb{Z}_{\ne 0}$, but $\tilde{a}(0)$ may or may not converge to $a(0)$ when $a(0)$ is defined and meaningful.

---

The following two analytic formulas for $\tilde{a}(x)$ are based on An Exact Formula for the Prime Counting Function, where the only difference between the two formulas is formula (4) starts the inner series at $j=1$ instead of $j=0$. Formulas (3) and (4) below are both indeterminate at $x=0$ (because $0^0$ is indeterminate) but they converge at $x=0$ in a limit sense. I'll note that formulas (3) and (4) are both extremely slow to converge and extremely sensitive to evaluation precision, and formula (4) generally diverges faster (at a smaller magnitude of $x$) than formula (3) when both formulas are evaluated at the same limit $I$.

---

$$\tilde{a}(x)=\underset{I\to\infty}{\text{lim}}\left(-2\sum\limits_{i=0}^I (-1)^i (2 \pi x)^{2 i} \sum\limits_{j=0}^i \frac{(-1)^j (2 \pi)^{-2 j} F_a(2 j)}{(2 i-2 j+1)!}\right)\tag{3}$$

$$\tilde{a}(x)=\underset{I\to\infty}{\text{lim}}\left(-2\sum\limits_{i=0}^I (-1)^i (2 \pi x)^{2 i} \sum\limits_{j=1}^i \frac{(-1)^j (2 \pi)^{-2 j} F_a(2 j)}{(2 i-2 j+1)!}\right)\tag{4}$$

---

I believe in some cases the analytic formula for $\tilde{a}(n)$ defined in formula (5) below may be related or equivalent to the analytic formulas defined in formulas (3) and (4) above. The analytic function $\tilde{a}(x)$ defined in formula (5) evaluates exactly to $a(n)$ when $x=n\in\mathbb{Z}\land0<|n|\le N$. When formulas (3) and (4) above converge for a particular definition of $a(n)$, there are at least two conditions related to the evaluation limit $N$ in formula (5) below that are necessary, but perhaps not sufficient, to achieve this equivalence which are discussed following formula (5) below.

---

$$\tilde{a}(x)=\underset{N\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N\frac{b(n)}{n}\sum\limits_{k=1}^n\cos\left(\frac{2 \pi k x}{n}\right)\right)\quad\text{where}\quad b(n)=\sum\limits_{d|n}a(d)\,\mu\left(\frac{n}{d}\right)\tag{5}$$

---

Condition (1): The first condition necessary for formula (5) to evaluate equivalent to formula (3) or (4) is the limit defined in formula (6) below must converge, and more specifically I believe it must converge to zero. If this limit diverges, formula (4) will still evaluate exactly to $a(n)$ when $x=n\in\mathbb{Z}\land0<|n|\le N$, but will diverge at non-integer values of $x$.

---

$$\underset{N\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N\frac{b(n)}{n}\right)=0\tag{6}$$

---

Condition (2): The second condition necessary for formula (5) to evaluate equivalent to formula (3) or (4) is it must be possible to evaluate $\tilde{a}(0)$ defined in formula (7) below to a particular value for arbitrarily large magnitudes of the evaluation limit $N$. This implies $\tilde{a}(0)$ defined in formula (7) below can be evaluated to this specific value for an infinite number of values of $N$.

---

$$\tilde{a}(0)=\sum\limits_{n=1}^N b(n)\tag{7}$$

---

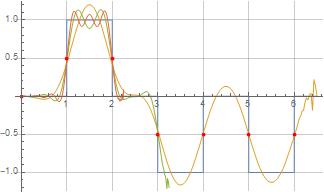
In all of the figures below, the red discrete portion of the plots represents the evaluation of the arithmetic function $a(n)$ at integer values. Formulas (3) and (4) are generally illustrated in blue (except Figure (1) which illustrates formula (4) in green) and formula (5) is always illustrated in orange.

---

In the case where $a(n)=1$, $f_a(x)=\lfloor x\rfloor$, $F_a(s)=\zeta(s)$, and $b(n)=\delta_{n-1}$ (Kronecker delta). For the case $a(n)=1$, Condition (2) specified above is met with respect to the potential equivalency of formulas (3) and (5), but the limit specified in Condition (1) above converges to $1$ instead of $0$.

---

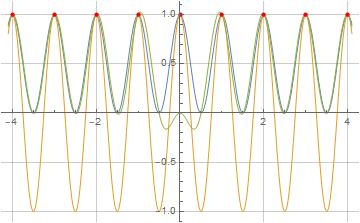
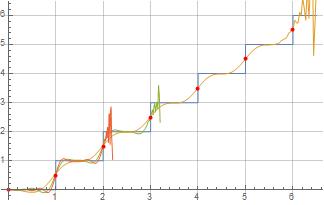
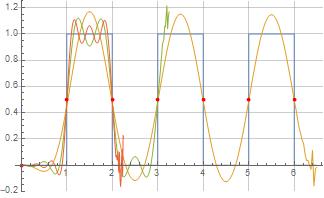
Figure (1) below illustrates for the case $a(n)=1$, formula (5) for $\tilde{a}(x)$ (orange) is more closely related to formula (3) for $\tilde{a}(x)$ (blue) than it is to formula (4) for $\tilde{a}(x)$ (green). In the case where $a(n)=1$, formula (3) for $\tilde{a}(x)$ corresponds to the power series for $\frac{1}{2}(1+\cos(2 \pi x))$, and formula (5) for $\tilde{a}(x)$ corresponds to $\cos(2 \pi x)$.

---

Figure (1): Illustration of formulas (3), (4), and (5) for $\tilde{a}(x)$ (blue, green, and orange) for $a(n)=1$

---

In the simplest case where $a(n)=\delta_{n-1}$ (Kronecker delta function), $f_a(x)=\theta(x-1)$, $F_a(s)=1$, and $b(n)=\mu(n)$. Note conditions (1) and (2) specified above are both met since $\underset{N\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N\frac{\mu(n)}{n}\right)=0$ and $\tilde{a}(0)=\sum\limits_{n=1}^N\mu(n)$ is the Mertens function $M(N)$ which evaluates to every integer an infinite number of times.

---

In the case of $a(n)=\delta_{n-1}$, formulas (8) and (9) below are equivalent to formulas (3) and (4) above where $_1\tilde{F}_2()$ is the Hypergeometric PFQ Regularized function.

---

$$\tilde{\delta}_{x-1}=\underset{I\to\infty}{\text{lim}}\left(\sum\limits_{i=0}^I (-1)^{i+1} \pi^{2 i+\frac{5}{2}} \, _1\tilde{F}_2\left(1;i+2,i+\frac{5}{2};-\pi ^2\right) x^{2 i}\right)\tag{8}$$

$$\tilde{\delta}_{x-1}=\underset{I\to\infty}{\text{lim}}\left(\sum\limits_{i=0}^I (-1)^i \pi^{2 i+\frac{1}{2}} \, _1\tilde{F}_2\left(1;i+1,i+\frac{3}{2};-\pi ^2\right) x^{2 i}\right)\tag{9}$$

---

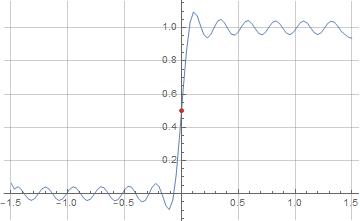
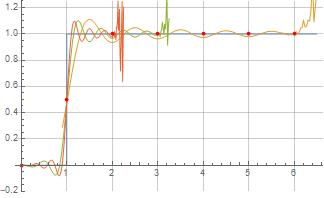
Figure (2) below illustrates formulas (3) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\delta_{n-1}$. Formula (3) is evaluated at $I=100$, and formula (5) is evaluated at $N=103$ which was selected to match the evaluation of formula (3) at $x=0$ which converges (in a limit sense) to $-2$. Note formula (5) evaluates so closely to formula (3) that the evaluation of formula (5) pretty much hides the underlying evaluation of formula (3).

---

Figure (2): Illustration of formulas (3) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\delta_{n-1}$

---

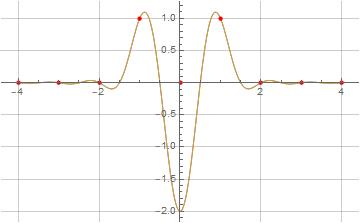
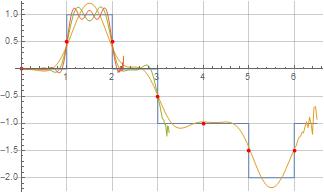
Figure (3) below illustrates formulas (4) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\delta_{n-1}$. Formula (4) is evaluated at $I=100$, and formula (5) is evaluated at $N=101$ which was selected to achieve the correct evaluation at $x=0$. Note formula (4) also converges (in a limit sense) to the correct value at $x=0$. Note formula (5) doesn't seem to evaluate as closely to formula (4) in Figure (3) below as it did to formula (3) in Figure (2) above. I've noticed formula (4) typically diverges faster (at a smaller magnitude of $x$) than formula (3) when both are evaluated at the same limit $I$ which may be part of the reason, but it may also be that formula (5) is not as closely related to formula (4) as it is to formula (3) which was clearly the case illustrated in Figure (1) above for $a(n)=1$.

---

Figure (3): Illustration of formulas (4) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\delta_{n-1}$

---

Question (1): Assuming $a(n)=\delta_{n-1}$, can it be shown that formula (5) above is exactly equivalent to formula (3) and/or formula (4) above as $I\to\infty$ and $N\to\infty$ with the additional constraint $N$ is selected such that formula (7) for $\tilde{a}(0)$ matches the evaluation of formula (3) and/or formula (4) for $\tilde{a}(x)$ at $x=0$?

---

In the case where $a(n)=\mu(n)$ (Moebius function), $f_a(x)=M(x)$ which is the Mertens function, $F_a(s)=\frac{1}{\zeta(s)}$, and $b(n)=\sum\limits_{d|n}\mu(d)\,\mu\left(\frac{n}{d}\right)$. Note condition (1) specified above is met since $\underset{N\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N \frac{b(n)}{n}\right)=0$ (see this answer to my related question on Math StackExchange). The second necessary condition for equivalence of formula (5) to formulas (3) or (4) in the case of $a(n)=\mu(n)$ is it must be possible to evaluate formula (7) above for $\tilde{a}(0)$ to $4$ or $0$ for arbitrarily large magnitudes of the limit $N$. I believe this condition is met but this is still an open issue (see my related question on Math StackExchange).

---

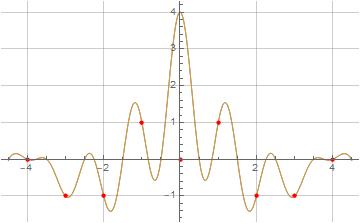
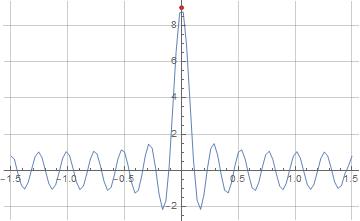
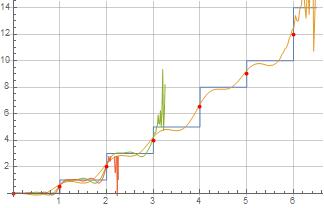
Figure (4) below illustrates formulas (3) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\mu(n)$. Formula (3) is evaluated at $I=100$, and formula (5) is evaluated at $N=141$ which was selected to match the evaluation of formula (3) at $x=0$ which converges (in a limit sense) to $4$. Note formula (5) evaluates so closely to formula (3) that the evaluation of formula (5) pretty much hides the underlying evaluation of formula (3).

---

Figure (4): Illustration of formulas (3) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\mu(n)$

---

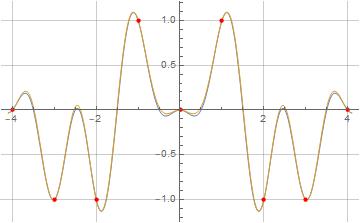
Figure (5) below illustrates formulas (4) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\mu(n)$. Formula (4) is evaluated at $I=100$, and formula (5) is evaluated at $N=140$ which was selected to achieve the correct evaluation at $x=0$. Note formula (4) also converges (in a limit sense) to the correct value at $x=0$. Note formula (5) doesn't seem to evaluate as closely to formula (4) in Figure (5) below as it did to formula (3) in Figure (4) above.

---

Figure (5): Illustration of formulas (4) and (5) for $\tilde{a}(x)$ (blue and orange) where $a(n)=\mu(n)$

---

Question (2): Assuming $a(n)=\mu(n)$, can it be shown that formula (5) above is exactly equivalent to formula (3) and/or formula (4) above as $I\to\infty$ and $N\to\infty$ with the additional constraint $N$ is selected such that formula (7) for $\tilde{a}(0)$ matches the evaluation of formula (3) and/or formula (4) for $\tilde{a}(x)$ at $x=0$?

---

In the case where $a(n)=(-1)^{n-1}$, $f_a(x)=\frac{1}{2}\left(1-\text{SquareWave}\left(\frac{x}{2}\right)\right)$, $F_a(s)=\eta(s)$ (Dirichlet eta function), and $b(n)=\{1,-2,0,0,0,...\}$ ($b(1)=1$, $b(2)=-2$, and $b(n)=0$ for $n>2$). For the case $a(n)=(-1)^{n-1}$, Conditions (1) and (2) specified above are both met with respect to the equivalency of formulas (3) and (5) for $\tilde{a}(x)$.

---

Figure (6) below illustrates for the case $a(n)=(-1)^{n-1}$, formula (5) for $\tilde{a}(x)$ (orange) is exactly equivalent to formula (3) for $\tilde{a}(x)$ (blue) but much less closely related to formula (4) for $\tilde{a}(x)$ (green). In the case where $a(n)=(-1)^{n-1}$, formula (3) for $\tilde{a}(x)$ corresponds to the power series for $-cos(\pi x)$, and formula (5) for $\tilde{a}(x)$ also corresponds to $-\cos(\pi x)$.

---

Figure (6): Illustration of formulas (3), (4), and (5) for $\tilde{a}(x)$ (blue, green, and orange) for $a(n)=(-1)^{n-1}$

---

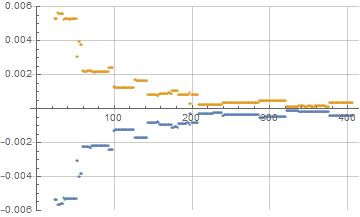
The discrete plot in Figure (7) below illustrates the relationship between the evaluation of the offset defined in formula (6) (orange) and the evaluation of formula (5) for $\tilde{a}(1.5)$ (blue) for the case $a(n)=\delta_{n-1}$ where $b(n)=\mu(n)$ and formula (7) for $\tilde{a}(0)$ corresponds to the Mertens function $M(N)$. Both formulas are evaluated at the zeros of the Mertens function in the range $1\le N\le 10000$ corresponding to Condition (2) necessary for the equivalence of formulas (4) and (5) for $a(n)=\delta_{n-1}$. There are $406$ zeros of the Merten's function in the range $1\le N\le 10000$ with the first occurring at $N=2$ and the last occuring at $N=9256$. The horizontal axis in Figure (6) below represents of the index of the Merten's function zero. Note the oscillations in the evaluation of $\tilde{a}(1.5)$ (blue) are opposite in sign but nearly equal in magnitude to the oscillations in the evaluation of the formula (6) offset (orange). Since the offset in formula (6) converges to zero as $N\to\infty$, it would seem the oscillations in the evaluation of formula (5) for $\tilde{a}(1.5)$ also converge to zero as $N\to\infty$ when $\tilde{a}(0)=M(N)=0$.

---

Figure (7): Illustration of formula (5) for $\tilde{a}(1.5)$ and the offset in formula (6) as a function 0f $N$ where $a(n)=\delta_{n-1}$ and the horizontal axis represents the index of $\tilde{a}(0)=M(N)=0$

---

The value $x=1.5$ was selected for Figure (7) above for a couple of reasons. First, formula (5) for $\tilde{a}(x)$ evaluates exactly correct when $x=n\in\mathbb{Z}\land0<|n|\le N$, so it seemed to me $\tilde{a}(x)$ would vary more halfway between integer values than it does when evaluated closer to integer values. Second, formula (5) for $\tilde{a}(1.5)$ and the offset corresponding to formula (6) both evaluate close to zero as $N$ increases which allows the plot range to be magnified when both functions are combined on the same plot thereby providing visibility into the finer details. The magnified plot range illustrated in Figure (7) above truncated some of the earlier evaluation points corresponding to smaller values of $N$ where formula (5) for $\tilde{a}(x)$ and the offset defined in formula (6) evaluated to larger magnitudes before they started to exhibit better convergence.

Answer 1

The function $f_a(x)=\sum\limits_{n\le x}a(n)$ defined in formula (1) in the question above can also be evaluated as defined in formula (a) below which leads to the analytic representations of $\tilde{f}_a(x)=\underset{\epsilon\to 0}{\text{lim}}\frac{f_a(x-\epsilon)+f_a(x+\epsilon)}{2}$ and it's first order derivative $\tilde{f}_a'(x)$ defined in formulas (b) and (c) below where the evaluation frequency $f$ is assumed to be a positive integer.

---

$$f(x)=\sum\limits_{n=1}^x a(n)=\sum\limits_{n=1}^x b(n)\ \left\lfloor \frac{x}{n}\right\rfloor\quad\text{where}\quad b(n)=\sum\limits_{d|n}a(d)\,\mu\left(\frac{n}{d}\right)\tag{a}$$

$$\tilde{f}_a(x)=\underset{N,f\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N b(n)\left(\frac{x}{n}-\left(\frac{1}{2}-\frac{1}{\pi}\sum\limits_{k=1}^{f\,n}\frac{\sin\left(\frac{2 \pi k x}{n}\right)}{k}\right)\right)\right),\quad x>0\tag{b}$$

$$\tilde{f}_a'(x)=\underset{N,f\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N\frac{b(n)}{n}\left(1+2\sum\limits_{k=1}^{f\,n}\cos\left(\frac{2 \pi k x}{n}\right)\right)\right),\quad x>0\tag{c}$$

---

Formulas (b) and (c) above correspond to analytic representations of $f(x)=\underset{N\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N a(n)\,\theta(x-n)\right)$ and $f'(x)=\underset{N\to\infty}{\text{lim}}\left(\sum\limits_{n=1}^N a(n)\,\delta(x-n)\right)$ where $\theta(x)$ is the Heaviside step function and $\delta(x)$ is the Dirac delta function.

---

When conditions (1) and (2) defined in the question above are met formulas (b) and (c) above can be simplified to formulas (d) and (e) below. The function $\tilde{f}_a'(x)$ defined in formula (e) below evaluates exactly to $2\,f\, a(n)$ when $x=n\in\mathbb{Z}\land0<|n|\le N$ which leads to the analytic formula for $\tilde{a}(x)$ defined in formula (5) in the question above. Formula (5) in the question above is simply formula (e) below multiplied by the normalization factor $\frac{1}{2\,f}$ and then evaluated at $f=1$.

---

$$\tilde{f}_a(x)=\underset{N,f\to\infty}{\text{lim}}\left(\frac{1}{\pi}\sum\limits_{n=1}^N b(n)\sum\limits_{k=1}^{f\,n}\frac{\sin\left(\frac{2 \pi k x}{n}\right)}{k}\right),\quad x>0\tag{d}$$

$$\tilde{f}_a'(x)=\underset{N,f\to\infty}{\text{lim}}\left(2\sum\limits_{n=1}^N\frac{b(n)}{n}\sum\limits_{k=1}^{f\,n}\cos\left(\frac{2 \pi k x}{n}\right)\right),\quad x>0\tag{e}$$

---

I've discovered the relationship between formula (4) in the question above and formula (e) above is deeper than I originally anticipated. I believe the following two analytic formulas for $\tilde{f}_a(x)$ and $\tilde{f}_a'(x)$ are valid when conditions (1) and (2) in the question above are met where once again the evaluation frequency $f$ is assumed to be a positive integer.

---

$$\tilde{f}_a(x)=\underset{\substack{K,f\to\infty \\ K\gg f\,x}}{\text{lim}}\left(-4 f \sum\limits_{k=1}^K \frac{(-1)^k\ x^{2 k+1}}{2 k+1} \sum\limits_{j=1}^k \frac{(-1)^j\ (2 \pi f)^{2(k-j)}\ F_a(2 j)}{(2 k-2 j+1)!}\right)\tag{f}$$

$$\tilde{f}_a'(x)=\underset{\substack{K,f\to\infty \\ K\gg f\,x}}{\text{lim}}\left(-4 f\sum\limits_{k=1}^K (-1)^k\ x^{2 k}\sum\limits_{j=1}^k \frac{(-1)^j\ (2 \pi f)^{2(k-j)}\ F_a(2 j)}{(2 k-2 j+1)!}\right)\tag{g}$$

---

When conditions (1) and (2) defined in the question above are met, I believe formulas (f) and (g) above are equivalent to formulas (d) and (e) above. When condition (1) is met but condition (2) is not met, I originally anticipated formulas (f) and (g) would still be valid even though formulas (d) and (e) above are no longer valid. When condition (1) is not met, I originally suspected additional terms might need to be added to formulas (f) and (g) to make them valid. But to my surprise formulas (f) and (g) above seem to evaluate correctly for every definition of $a(n)$ I've tested, but I'll note these evaluations were over very small ranges of $x$ using very small evaluation limits.

---

I've tested formulas (f) and (g) above for the function $a(n)=\delta_{n-1}$ where conditions (1) and (2) are met, and also for the function $a(n)=\mu(n)$ where condition (1) is met and I suspect condition (2) is also met. Formulas (f) and (g) seem to evaluate correctly for both of these cases. For $a(n)=\delta_{n-1}$, the functions $\tilde{f}(x)$ and $\tilde{f}'(x)$ correspond to analytic representations of $-1+\theta(x+1)+\theta(x-1)$ and $\delta(x+1)+\delta(x-1)$. For the case $a(n)=\mu(n)$, the functions $\tilde{f}(x)$ and $\tilde{f}'(x)$ correspond to analytic representations of the Mertens function $M(x)$ and it's first-order derivative.

---

I've tested formulas (f) and (g) above for the function $a(n)=1$ associated with the Dirichlet series for $\zeta(s)$. In this case, formula (f) above for $\tilde{f}(x)$ seems to evaluate correctly, and formula (g) above for $\tilde{f}'(x)$ seems to evaluate to a Dirac comb with a tooth missing at $x=0$. This seems to suggest the following analytic formulas for $\theta(x)$ and $\delta(x)$ where the functions $\tilde{f}_a(x)$ and $\tilde{f}_a'(x)$ referenced in formulas (h) and (i) below are defined in formulas (f) and (g) above and are assumed to be evaluated with $F_a(s)=\zeta(s)$ and with the same evaluation frequency $f$ used to evaluate formulas (h) and (i) below.

---

$$\theta(x)=\underset{f\to\infty}{\text{lim}}\left(\frac{1}{2}+\frac{1}{\pi}\sum\limits_{k=1}^f \frac{\sin(2 \pi k x)}{k}-\left(\tilde{f}_a(x)-x\right)\right)\tag{h}$$

$$\delta(x)=\underset{f\to\infty}{\text{lim}}\left(2\sum\limits_{k=1}^f \cos(2 \pi k x)-\left(\tilde{f}_a'(x)-1\right)\right)\tag{i}$$

---

Figures (1) and (2) below illustrate formulas (h) and (i) for $\theta(x)$ and $\delta(x)$ above both seem to evaluate pretty much as expected where both formulas were evaluated with $f=4$ and $K=200$.

---

Figure (1): Illustration of Formula (h) for $\theta(x)$ evaluated at $f=4$ and $K=200$

---

Figure (2): Illustration of Formula (i) for $\delta(x)$ evaluated at $f=4$ and $K=200$

---

I've also tested formulas (f) and (g) above for the function $a(n)=(-1)^{n-1}$ associated with the Dirichlet series for $\eta(s)$. In this case, formula (f) above for $\tilde{f}(x)$ seems to evaluate correctly, and formula (g) above for $\tilde{f}'(x)$ seems to evaluate to the sum of two Dirac combs where one of them has a tooth missing at $x=0$.

---

I've also tested formulas (f) and (g) above for the functions $a(n)=\lambda(n)$, $a(n)=\sigma_0(n)$, $a(n)=\Lambda(n)$, and $a(n)=\frac{\Lambda(n)}{\log n}$ associated with the Dirichlet series for $\frac{\zeta(2s)}{\zeta(s)}$, $\zeta(s)^2$, $\frac{\zeta'(s)}{\zeta(s)}$, and $\log\zeta(s)$, and to my surprise both formulas seem to evaluate correctly for these cases as well.

---

Assuming the function $G_a(s)$ defined in formula (j) below converges, it can perhaps be used to derive formulas for functions like $F_a(s)=\zeta(s)$ and $F_a(s)=\frac{\zeta'(x)}{\zeta(s)}$ associated with $a(n)=1$ and $a(n)=\Lambda(n)$ from relationships like those illustrated in formulas (k) and (l) below.

---

$$G_a(s)=s\int\limits_1^\infty\tilde{f}(x)\,x^{-s-1}\,dx=\underset{\substack{K,f\to\infty \\ K\gg f\,x}}{\text{lim}}\left(-4 f \sum\limits_{k=1}^K \frac{(-1)^k}{(2 k+1)}\frac{s}{(s-2 k-1)}\sum\limits_{j=1}^k \frac{(-1)^j\ (2 \pi f)^{2(k-j)}\ F_a(2 j)}{(2 k-2 j+1)!}\right)\tag{j}$$

---

$$\zeta(s)=\frac{s}{s-1}+s\int_1^\infty (\lfloor x\rfloor-x)\ x^{-s-1}\ dx\,,\quad\Re(s)>0\tag{k}$$

$$\frac{\zeta'(s)}{\zeta(s)}=\frac{s}{1-s}-s\int_1^\infty (\psi(x)-x)\ x^{-s-1}\ dx\,,\quad\Re(s)>\frac{1}{2}\quad\text{(assuming RH)}\tag{l}$$

---

The following figures illustrate formula (f) for $\tilde{f}_a(x)$ above for a variety of arithmetic functions in an attempt to lend some credence to the validity of formulas (f) and (g) above. In each figure below, the reference function is illustrated in blue and formula (f) for $\tilde{f}_a(x)$ is illustrated in orange, green, and red corresponding to the evaluation frequencies $f=1$, $f=2$, and $f=3$ respectively. The red discrete evaluation points at integer values of $x$ in the figures below represent the evaluation of $f_{a_o}(x)=\frac{1}{2}(f_a(x-\epsilon)+f_a(x-\epsilon))$. The evaluation limit $K=100$ was used in all figures below.

---

All figures below share a few common characteristics. First, note that in all cases the evaluation at $f=1$ (shown in orange) seems to converge to $f_{a_o}(x)$ at integer values of $x$. Second, note that in all cases increasing the evaluation frequency decreases the range of convergence with respect to $x$, but improves the fidelity of the approximation to $f_{a_o}(x)$ before the evaluation starts to diverge. The evaluation of each frequency was terminated slightly after it started to diverge in the figures below to prevent it from corrupting the rest of the plot range.

---

Figure (1): Illustration of $f_a(x)=\lfloor x\rfloor$ where $F_a(s)=\zeta(s)$

---

Figure (2): Illustration of $f_a(x)=\sum\limits_{n\le x}(-1)^{n-1}$ where $F_a(s)=\eta(s)$

---

Figure (3): Illustration of $f_a(x)=\theta(x-1)$ where $F_a(s)=1$

---

Figure (4): Illustration of $f_a(x)=M(x)$ (Mertens function) where $F_a(s)=\frac{1}{\zeta(s)}$

---

Figure (5): Illustration of $f_a(x)=D(x)$ (divisor summatory function) where $F_a(s)=\zeta(s)^2$

---

Figure (6): Illustration of $f_a(x)=L(x)$ (summatory Liouville function) where $F_a(s)=\frac{\zeta(2s)}{\zeta(s)}$

---

Figure (7): Illustration of $f_a(x)=\psi(x)$ (second Chebyshev function) where $F_a(s)=\frac{-\zeta'(s)}{\zeta(s)}$

---

Figure (8): Illustration of $f_a(x)=\Pi(x)$ (Riemann prime counting function) where $F_a(s)=\log\zeta(s)$

---

For the cases $a(n)=1$, $a(n)=(-1)^{n-1}$ and $a(n)=\delta_{n-1}$ where $F_a(s)=\zeta(s)$, $F_a(s)=\eta(s)$, and $F_a(s)=1$, I've determined formulas (f) and (g) above are simply the power series for the functions defined in formulas (m) to (r) below. This proves the validity of formulas (f) and (g) above for these particular cases, but I believe formulas (f) and (g) are more generally applicable to any definition of $a(n)$ for which the Dirichlet series $F_a(s)=\sum\limits_n\frac{a(n)}{n^s}$ converges for $\Re(s)\ge 2$. All formulas below are for $x\ge 0$, but $\tilde{f}_a'(x)$ and $\tilde{f}_a(x)$ are actually even and odd functions respectively.

---

$\quad a(n)=1 \text{ where } F_a(s)=\zeta(s)$:

$$\tilde{f}_a'(x)=\sum\limits_n\delta(x-n)=\underset{f\to\infty}{\text{lim}}\left(-\frac{\sin (2 f \pi x)}{\pi x}+\sum\limits_{n=1}^f (\cos(2 n \pi x)+\cos(2 (n-1) \pi x))\right)\tag{m}$$

$$\tilde{f}_a(x)=\sum\limits_n\theta(x-n)=\underset{f\to\infty}{\text{lim}}\left(-\frac{\text{Si}(2 f \pi x)}{\pi}+\sum\limits_{n=1}^f \left(\frac{\sin(2 n \pi x)}{2 n \pi}+x\ \text{sinc}(2 (n-1) \pi x)\right)\right)\tag{n}$$

---

$\quad a(n)=(-1)^{n-1} \text{ where } F_a(s)=\eta(s)$:

$$\tilde{f}_a'(x)=\sum\limits_n (-1)^{n-1}\delta(x-n)=\underset{f\to\infty}{\text{lim}}\left(\frac{\sin(2 f \pi x)}{\pi x}-2 \sum\limits_{n=1}^f \cos((2 n-1) \pi x)\right)\tag{o}$$

$$\tilde{f}_a(x)=\sum\limits_n (-1)^{n-1}\theta(x-n)=\underset{f\to\infty}{\text{lim}}\left(\frac{\text{Si}(2 f \pi x)}{\pi }-\frac{2}{\pi}\sum\limits _{n=1}^f \frac{\sin ((2 n-1) \pi x)}{2 n-1}\right)\tag{p}$$

---

$\quad a(n)=\delta_{n-1} \text{ where } F_a(s)=1$:

$$\tilde{f}_a'(x)=\delta(x-1)=\underset{f\to\infty}{\text{lim}}\left(\frac{\sin(2 f \pi (x+1))}{\pi (x+1)}+\frac{\sin(2 f \pi (x-1))}{\pi (x-1)}\right)\tag{q}$$

$$\tilde{f}_a(x)=\theta(x-1)=\underset{f\to\infty}{\text{lim}}\left(\frac{\text{Si}(2 f \pi (x+1))+\text{Si}(2 f \pi (x-1))}{\pi }\right)\tag{r}$$

---

Formulas (m) to (p) above lead to the following formulas for the Riemann zeta function $\zeta(s)$ and Dirichlet eta function $\eta(s)$. Formula (s) and (t) for $\zeta(s)$ and $\eta(s)$ can also be used to derive formulas for $\zeta(s)$ and $\eta(s)$ which converge for $\Re(s)>-1$.

---

$$\zeta(s)=\underset{f\to\infty}{\text{lim}}\left(2^s \pi^{s-1} \sin\left(\frac{\pi s}{2}\right) \Gamma(1-s) \left(-\frac{f^s}{s}+\frac{1}{2} \left(1+\sum\limits_{n=2}^f \left(n^{s-1}+(n-1)^{s-1}\right)\right)\right)\right),\ \Re(s)<2\tag{s}$$

$$\eta(s)=\underset{f\to\infty}{\text{lim}}\left(2 \pi^{s-1} \sin\left(\frac{\pi s}{2}\right)\ \Gamma(1-s) \left(\frac{2^{s-1} f^s}{s}-\sum\limits_{n=1}^f (2 n-1)^{s-1}\right)\right),\ \Re(s)<2\tag{t}$$

---

Formulas (n), (p), (r), and (t) above are illustrated in this answer I recently posted to my own question on MathOverflow.