5
$\begingroup$

A convex polygon all of whose vertices have integer coordinates is a convex lattice polygon.

  1. Do there exist mutually non-congruent convex lattice polygons which have the same area and same perimeter?

  2. If answer to 1 is yes, are there convex lattice polygons which can be cut into some integer number of convex lattice polygons which are not all congruent and all have same area and same perimeter?

Note: The questions have natural analogs in higher dimensions.

Improve this question
$\endgroup$
4
  • 3
    $\begingroup$ There are even pairs (and indeed triples, quadruples, etc.) of lattice triangles satisfying this condition, and with the perimeter as well as the area integral. For example, se16.info/hgb/triangleareaperimeter.htm shows the 24-37-37 and 29-29-40 triangles, both isosceles with area 420 and perimeter 98, that can be made with vertices at $(\pm 20, 0), (0, 21)$ and $(\pm 12, 0), (0, 35)$, and a third triangle attaining the same area and perimeter with sides 25-34-39 that can be realized as a lattice triangle with vertices $(0,0), (16,30), (36,15)$. $\endgroup$
    – Noam D. Elkies
    Commented Mar 14, 2021 at 6:20
  • 1
    $\begingroup$ A parallelogram and a kite. $\endgroup$
    – Wlod AA
    Commented Mar 14, 2021 at 6:45
  • $\begingroup$ Thanks for pointing out this quite surprising result! Guess one can find any number of such non-congruent lattice triangles all of equal area and equal perimeter - if one considers big enough values for area and perimeter! One further query would be if such triangles and convex polygons can also be found on lattices where the unit cell is not a unit square but a parallelogram with rational length sides and the slope (measured by the angle between the basic axes) is also rational. $\endgroup$
    – Nandakumar R
    Commented Mar 14, 2021 at 10:55
  • $\begingroup$ Perhaps the smallest pair of triangles would be the $17-25-28$ and $20-21-29$, both with perimeter $70$ and area $210$. The second is a right triangle, the first is the juxtaposition of the $8-15-17$ and $15-20-25$ right triangles. $\endgroup$
    – Gerry Myerson
    Commented Mar 17, 2021 at 7:45

3 Answers 3

Reset to default
5
$\begingroup$

This answers question 2 as well. But I think both questions are way more suitable for math.stackexchange.

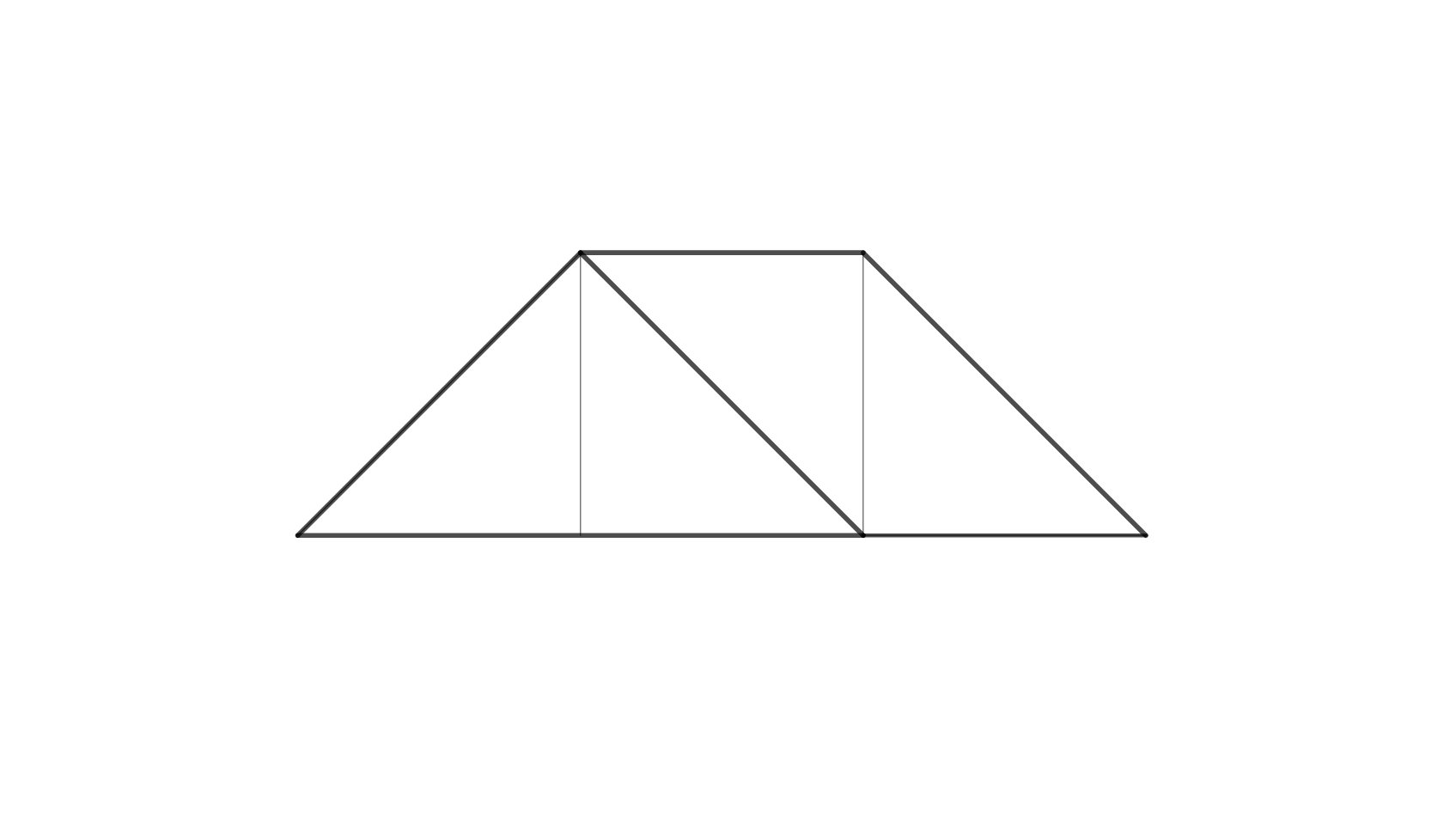
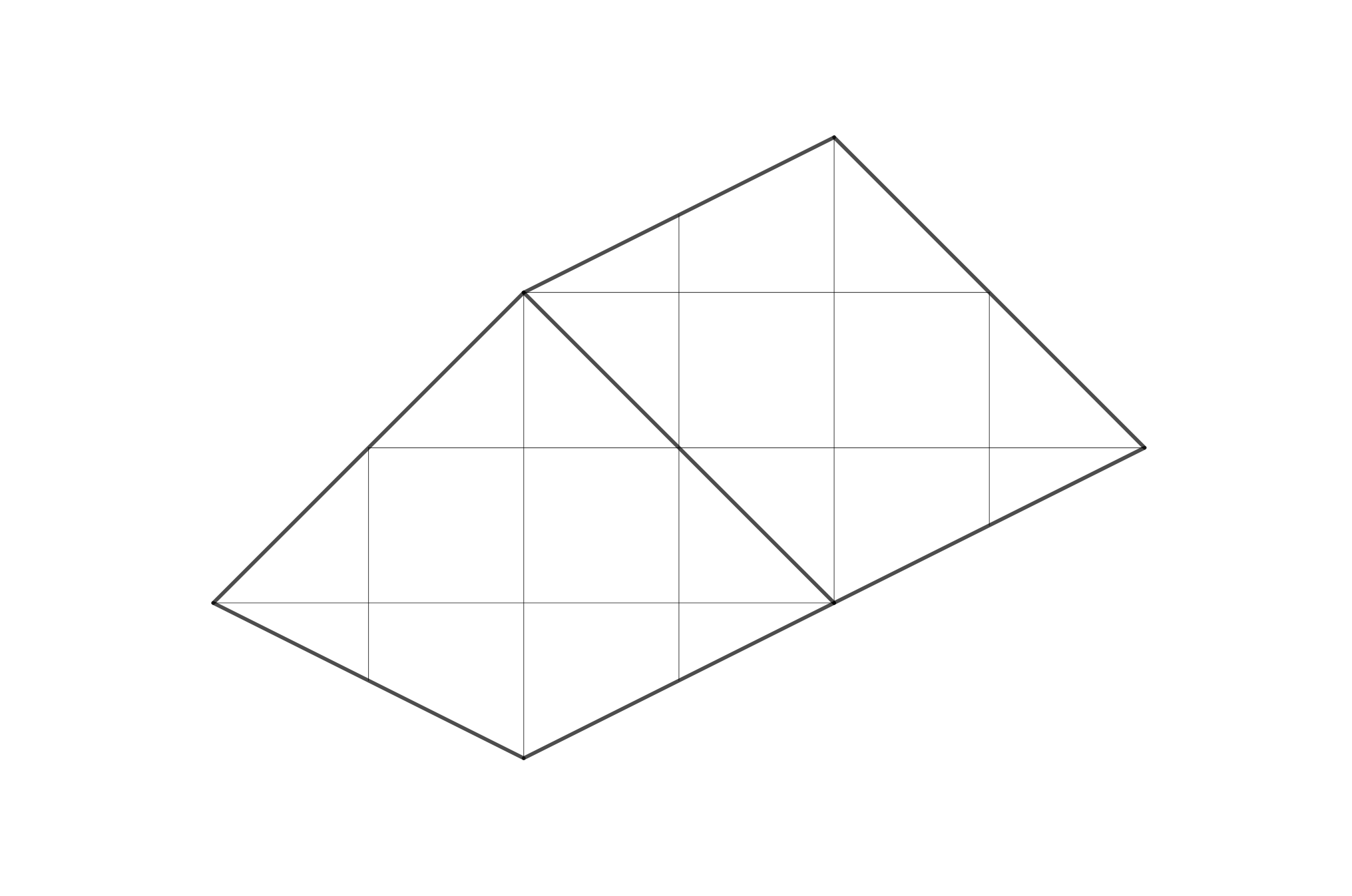
enter image description here

I add another example because, unlike the first, it has the property that multiplied by $I^{n-2}$ it gives further examples in $\mathbb{R}^n$ as well:

enter image description here

Improve this answer
$\endgroup$
3
  • $\begingroup$ That was very neat. Thanks! Just wondering if there is a case where convex lattice pieces can all be mutually non-congruent ... $\endgroup$
    – Nandakumar R
    Commented Mar 14, 2021 at 5:59
  • $\begingroup$ Probably yes. Make a suitable convex piece that is roughly the shape of a circular sector in the positive quadrant of the coordinate system, with enough corners, and symmetric about the $y=x$ line. Mirror it to the three other quadrants and you have four congruent pieces. Then add small bumps to the "sectors", but in different places, making them all incongruent. If you have enough corners and large enough pieces, you should be able to do this while maintaining convexity and the other conditions. $\endgroup$
    – Jukka Kohonen
    Commented Mar 14, 2021 at 7:06
  • $\begingroup$ Please note that the comments above were relevant prior to later edits to my answer. $\endgroup$
    – Yaakov Baruch
    Commented Mar 14, 2021 at 7:51
13
$\begingroup$

    Octagons

Pick's theorem says these two convex lattice polygons have area $$i+\frac{b}{2}-1 = 4 + 10/2 -1 = 8 \;,$$ and they both have perimeter $8 + 2 \sqrt{2}$. You can see I've "bumped out" two corners of an underlying octagon.

(I am interpreting the OP's phrase "the same area and perimeter" as "the same area and the same perimeter" as opposed to "the same area and the same perimeter, with the area numerically equal to the perimeter.")

Improve this answer
$\endgroup$
4
  • 3
    $\begingroup$ The central octagon can be shrunk to a square for an even simpler example. $\endgroup$
    – Yaakov Baruch
    Commented Mar 14, 2021 at 4:32
  • $\begingroup$ Really nice answer... Thanks! And, I understand Pick's theorem does not hold in 3D. Maybe in higher dimensions, the question has more ramifications. And yes, "same area and same perimeter" it ought to be. Edited! $\endgroup$
    – Nandakumar R
    Commented Mar 14, 2021 at 5:59
  • 2
    $\begingroup$ @NandakumarR First, for this nice example, and many others as well, Pick's theorem is not needed, for equality of areas and perimeters is seen directly. Second, in higher dimensions analogous examples are easily created by taking the Cartesian product with the unit interval, repeatedly. $\endgroup$
    – Wlodek Kuperberg
    Commented Mar 16, 2021 at 17:19
  • $\begingroup$ Thanks Wlodek Kuperberg for clarifying the role of Pick's theorem. Reg 3D, while it is indeed easy, as you point out, to make examples by taking a cartesian product, it might be of interest to find polyhedrons which are properly 3D (ie cannot be constructed simply by 'lifting' a 2D example) with the desired property. $\endgroup$
    – Nandakumar R
    Commented Mar 20, 2021 at 16:59
2
$\begingroup$

My earlier comment "parallelogram and kite" was signaling an infinite family of examples of groups of $m$ convex lattice polygons where all of them from the same group have the same diameter, perimeter, and area (where finite $m$ can be arbitrarily large). There are even two such infinite families.

The FIRST two power families:

Let $\ d_1>d_2>\ldots>d_n>0,\,\ $ and $\,\ y_0 > 0\,\ $ and $\,\ y_k=y_{k-1}+d_k\ $ for $\ k=1\ldots n.$

Let $\ 0\le a_0<a_1<\ldots a_n\,\ $ and $\,\ x_0:=X\ \ge \ a_0+ 2\cdot(a_0+\ldots +a_{n-1})+ a_n.$

We start with $4$ vertices $\ (0\ 0)\ $ and $\ (X\ 0)\,\ $ and $\ (\xi_0\ y_0):=(0\ y_0)\ $ and $\ (\eta_0\ y_0):=(x_0\ y_0).$

Next, we have $\ (\xi_k\ y_k)\,$ and $\ (\eta_k\ y_k),\ $ where either $$ \xi_k:=\xi_{k-1}+a_k\qquad\text{and}\qquad \eta_k:=\eta_{k-1}-a_{k-1} $$ or $$ \xi_k:=\xi_{k-1}+a_{k-1}\qquad\text{and}\qquad \eta_k:=\eta_{k-1}-a_k $$ for every $\ k=1\ldots n.$

This way, for fixed $d_k$ and $a_k$ and $X$, we get a family of $2^n$ polygonal when considering all above vertices, and we get an additional $2^n$ polygons when we omit $\ (0\ 0)\ $ and $\ (X\ 0).$ Within each of these two groups the perimeter and the area is respectively the same.

Furthermore, plenty of them can have the same diameter as well. In particular, when you put some additional constraints then all of them within the same group will have the same diameter equal to $\ \sqrt{h_0^{\,2}+X^2}\ $ in the case of $2^{n+1}$-group or $\ X\ $ in the case of the $2^n$-group respectively.

The SECOND two power families:

You take two members (the two can be the same) of one of these families, you take a mirror reflection of one of these two members w.r. to the x-axis in the case of the $2^{n+1}$-group, and w.r. to the line parallel line $y=y_0$. The union set of the vertices of these two polygons forms the vertex set of a respective convex lattice polygon of the SECOND type, and we have a similar situation as in the FIRST case.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .