Show commutativity of a diagram involving multiplier $C^*$-algebras

Question

Let me recall the following fact:

If $A$ is a $C^*$-algebra and $\pi: A \to \mathcal{B}(\mathcal{H})$ is a faithful non-degenerate representation, then we can explicitely realise the multiplier algebra $M(A)$ as bounded operators on $H$. More precisely, $M(A) \cong M_\pi(A)$ where $$M_\pi(A) = \{T \in \mathcal{B}(\mathcal{H})\mid T\pi(A) \subseteq \pi(A), \ \pi(A) T \subseteq \pi(A)\}.$$

---

Let $A$ be a $C^*$-algebra and $\mathcal{H}$ be a Hilbert space. The canonical $*$-morphism $$\mathcal{B}_0(\mathcal{H}) \otimes A \to \mathcal{B}_0(\mathcal{H}) \otimes A \otimes A: x \otimes a \mapsto x \otimes a \otimes 1$$ is non-degenerate, so extends uniquely to a strict $*$-morphism between the multiplier algebras. Let $\pi_u$ be a faithful and non-degenerate representation of $A$ on the Hilbert space $\mathcal{K}$. Let $\text{id}$ be the identity representation of $\mathcal{B}_0(\mathcal{H})$. I want to show that the following diagram commutes:

I can see that we have two maps $$\mathcal{M}(\mathcal{B}_0(\mathcal{H}\otimes A) \to \mathcal{M}(\mathcal{B}_0(\mathcal{H}\otimes A \otimes A)$$ such that $\mathcal{B}_0(\mathcal{H})\otimes A \ni x \otimes a \mapsto x \otimes a \otimes 1$. Somehow I want to invoke uniqueness of the extension that these maps must agree everywhere but for that I need to show that the composition along the two isomorphisms and the map $T \mapsto T \otimes 1$ (first down, then go right, then go up) is strictly continuous, and I can't see why this holds.

Question: What is the quickest way to see that this diagram commutes?

Answer 1

This is just a definition chase. Let me state a more general result: let $\newcommand{\mc}{\mathcal} C,D$ be $C^\ast$-algebras, let $\pi_C: C\rightarrow\mc B(H_C)$ be a faithful $*$-representation, and similarly $\pi_D$ on $H_D$. Let $\phi:C\rightarrow D$ be a non-degenerate $*$-homomorphism with extension $\tilde\phi:M(C)\rightarrow M(D)$. Similar remarks would apply to $\phi:C\rightarrow M(D)$.

Let $\alpha:\mc B(H_C)\rightarrow\mc B(H_D)$ be a $*$-homomorphism such that the following diagram commutes:$\require{AMScd}$

$$ \begin{CD} C @>\phi>> D \\ @V{\pi_C}VV @VV{\pi_D}V\\ \mc B(H_C) @>\alpha>> \mc B(H_D) \end{CD} $$

Then I claim also the following diagram commutes:

$$ \begin{CD} M(C) @>{\tilde\phi}>> M(D) \\ @V{\tilde\pi_C}VV @VV{\tilde\pi_D}V\\ \mc B(H_C) @>\alpha>> \mc B(H_D) \end{CD} $$

Here $\tilde\pi_C:M(C)\rightarrow\mc B(H_C)$ is the extension map, which sends $M(C)$ to $M_{\pi_C}(C) = \{ T\in\mc B(H_C) : T\pi_C(a), \pi_C(a)T\in\pi_C(C) \ (a\in C) \}$.

To show the claim, let $x\in M(C), c\in C$ so $\phi(xc) = \tilde\phi(x)\phi(c)$ and hence $$ \alpha(\tilde\pi_C(x)) \pi_D(\phi(c)) = \alpha(\tilde\pi_C(x)) \alpha(\pi_C(c)) = \alpha(\pi_C(xc)) = \pi_D(\phi(xc)) = \tilde\pi_D(\tilde\phi(x)) \pi_D(\phi(c)). $$ As $\phi$ and $\pi_D$ are non-degenerate both $\{ \phi(c)d : c\in C, d \in D \}$ is linear dense in $D$, and $\{ \pi_D(\phi(c)) \pi_D(d) \xi : c\in C, d\in D, \xi\in H_D \}$ is linearly dense in $H_D$. So we have that $$ \alpha(\tilde\pi_C(x)) \pi_D(\phi(c)) \pi_D(d) \xi = \tilde\pi_D(\tilde\phi(x)) \pi_D(\phi(c)) \pi_D(d) \xi $$ for all $c,d,\xi,x$ and hence $$ \alpha(\tilde\pi_C(x)) = \tilde\pi_D(\tilde\phi(x)) \qquad (x\in M(C)), $$ as required.

---

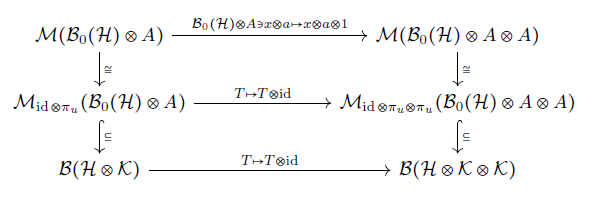
In your case, set $C=\mc B_0(H)\otimes A$ and $D=\mc B_0(H)\otimes A\otimes A$ with $\phi(c) = c\otimes 1$ and $\alpha(T)=T\otimes 1$, and $\pi_C, \pi_D$ the obvious maps to $H\otimes K$ and $H\otimes K\otimes K$, respectively. The middle row of your commutative diagram follows by restriction.

---

Notice that I did not assume that $\alpha$ was "continuous" in any sense, but that the first diagram commutes imposes conditions on $\alpha$. That $\pi_D$ and $\phi$ are non-degenerate shows that $\alpha$ is non-degenerate in the sense that $\{ \alpha(T)\xi : T\in\mc B(H_C), \xi\in H_D \}$ is linear dense in $H_D$. Indeed, also $\pi_C$ is non-degenerate, and this shows that also $\pi_C:C\rightarrow M(\mc B_0(H_C))$ is non-degenerate, so $\{ \pi_C(c) t : c\in C, t\in\mc B_0(H_C) \}$ is linearly dense in $\mc B_0(H_C)$. Thus, if $\alpha$ is at least bounded, \begin{align*}\newcommand{\lin}{\operatorname{lin}} &\overline{\lin}\{ \alpha(t)s : t\in \mc B_0(H_C), s\in \mc B_0(H_D) \} \\ &= \overline{\lin}\{ \alpha(\pi_C(c)t)s :c\in C,t\in \mc B_0(H_C), s\in \mc B_0(H_D) \} \\ &= \overline{\lin}\{ \pi_D(\phi(c)) \alpha(t) s:c\in C,t\in \mc B_0(H_C), s\in \mc B_0(H_D) \}. \end{align*} However, this does not seem to necessarily be all of $\mc B_0(H_D)$. Thus, if we consider $\mc B(H_C)$ as $M(\mc B_0(H_C))$, and restrict $\alpha$ to $\mc B_0(H_C)$ say giving $\alpha_0:\mc B_0(H_C) \rightarrow \mc B(H_D)$, I do not see why necessarily $\alpha_0$ is non-degenerate; equivalently, whether $\alpha$ is strictly continuous. It is in your case, of course.