A zonotope is a polytope whose 2-faces are centrally symmetric.
Question: If a polytope $P$ is centrally symmetric and combinatorially equivalent to a zonotope, is it itself a zonotope?
There are polytopes whose normal fans are central hyperplane arrangements which are not zonotopes. These are called "belt polytopes." I think they would give a counterexample to your question.
In retrospect, there are easy counterexamples.
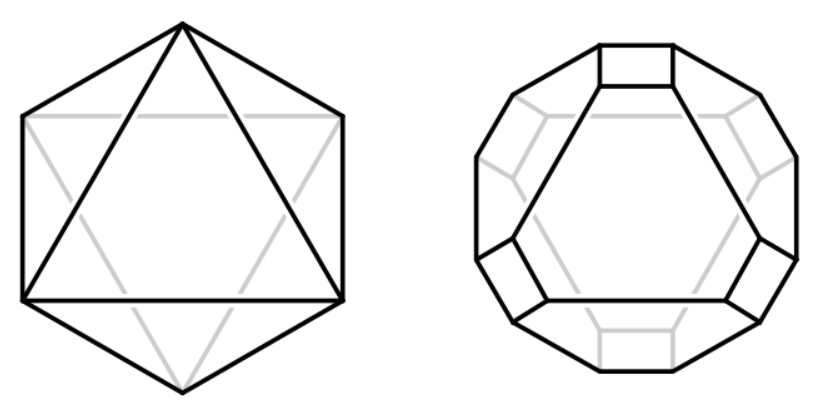
For example, the truncatd octahedron (or permutahedron) is a zonotope. However, below (on the right) is a centrally symmetric realization that is not a zonotope (it has non-centrally symmetric faces):