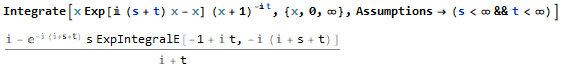Suppose we have two random variables $X$ and $Y$ both distributed as gamma random variables with parameters $\alpha_X,\beta_{X},\alpha_{Y},\beta_Y$, with characteristic functions given by:
$$\phi_{X}(t) = \left(1 - \frac{it}{\beta_X}\right)^{-\alpha_X} \;\;\text{ and }\;\;\phi_{Y}(t) = \left(1 - \frac{it}{\beta_Y}\right)^{-\alpha_Y}$$
Furthermore, suppose that $X$ and $Y$ are comonotone : the joint c.d.f is then given, via the upper frechet-hoffding bound, by :
$$F_{X,Y}(x,y) = \min(F_X(x),F_Y(y))$$
Note that this random vector has no density.
Can we obtain a formulation for the joint characteristic function ? Same question for more than $2$ comonotone gammas, say $X_i$ with parameters $\alpha_i,\beta_i$ for $i \in \{1,...,n\}$.