Proof that a quasicategory is equivalent to the homotopy coherent nerve of a simplicial category

Question

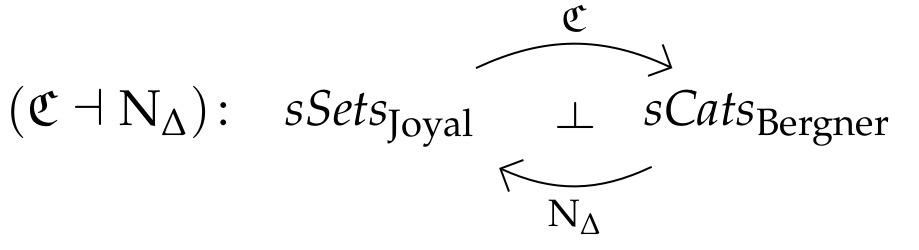
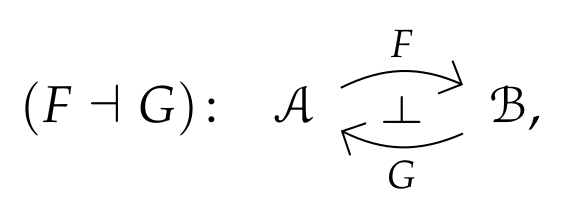On the abstract of a paper by Emily Riehl and Dominic Verity, it is stated that

Every quasicategory arises as a the homotopy coherent nerve of a simplicial category up to equivalence.

Where can one find a proof of this statement?

---

On motivation for the question, HTT 4.2.4.1 would then imply that any colimit in a quasicategory could be computed as a homotopy colimit of a diagram $F\colon\mathbf{J}\rightarrow\mathbf{C}$, where $\mathbf{J}$ and $\mathbf{C}$ are simplicial categories.

Answer 1

Sorry, we should have made this more clear. One proof appears as Theorem 7.2.2 in our previous paper in this series, The comprehension construction. As suggested by others, it follows from a suitably defined Yoneda embedding (which is hard to construct in the ∞-categorical setting).

Explicitly we show that a quasi-category B is equivalent to the homotopy coherent nerve of the full simplicial subcategory of the slice category qCat/B spanned by the right fibrations B/b -> B for each vertex b in B. Because the only objects we consider here are right fibrations the hom-spaces between two such in qCat/B are automatically Kan complexes.

The hard part of this is defining the map of quasi-categories from B to the homotopy coherent nerve, which by adjunction we construct as a simplicially enriched functor indexed by the "homotopy coherent realization" of B.

Answer 2

Let $\mathbf{C}$ be a quasicategory. Using a version of the Yoneda lemma for quasicategories, Joyal constructs, in Section 15.3 of his Notes on Quasi-Categories, a simplicial category $\overline{\mathbf{C}}$ such that $\mathrm{N}_\Delta(\overline{\mathbf{C}})$ is equivalent to $\mathbf{C}$.

Answer 3

The result is actually valid for any simplicial set.

Lemma. If

is a Quillen equivalence, then the composition $$A\xrightarrow{\eta_A}G(F(A))\xrightarrow{G\left(P_{F(A)}\right)}G(P(F(A)))$$ is a weak equivalence, where $P(A)$ denotes the fibrant replacement of an object $A\in\mathscr{A}$.

Proof. See Lemma 3.5.2 of these notes for a proof.

Now, HTT 2.2.5.1 states that $(\mathfrak{C},\mathrm{N}_\Delta)$ gives a Quillen equivalence:

The above lemma then implies that given a cofibrant object in $\mathit{sSets}_\mathrm{Joyal}$ (that is, any simplicial set) $S_\bullet$, we have a weak equivalence $$S_\bullet\rightarrow\mathrm{N}_\Delta\left(P(\mathfrak{C}[S_\bullet])\right).$$

In particular, if $\mathscr{C}$ is a quasicategory, then $Q(\mathfrak{C}[\mathscr{C}]) $ is a simplicial category whose homotopy coherent nerve is equivalent to $\mathscr{C}$.