Problem on distances in a polygon

Question

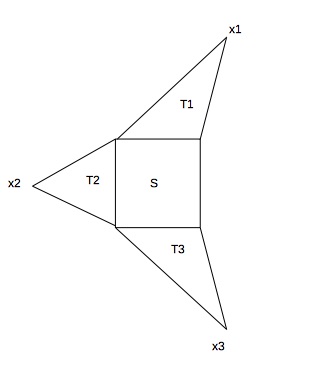
In $\mathbb{R}^2$ consider a square (call it $S$) and three triangles (one acute $T_2$ and two obtuse $T_1$ and $T_3$) such that each triangle shares one different side with the square and the triangles and the square are disposed exactly as in the following picture.

Define $P:=S\cup T_1\cup T_2\cup T_3$. Call $x_i$ the vertex of $T_i$ opposed to the side of $T_i$ shared with the square $S$. Choose any point $p$ inside the square $S$ such that all three segments $\overline{px_i}$ are entirely contained in $P$.

Now move all the vertexes of $P$ in a continuous way in such a manner that all the following lengths are not increased:

• the lengths of all the sides of the square and of the three triangles

• the lengths of the two diagonals of the square and the lengths of the segments from each $x_i$ to the vertexes of the square which are contained in $P$.

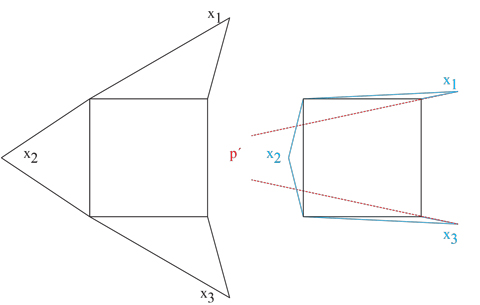
In the following picture I've drawn in blue all the segments whose lengths are not increased moving the vertexes of $P$:

Call $P':=S'\cup T_1'\cup T_2'\cup T_3'$ the polygon obtained in such a manner and $x_i'$ the vertexes of the new triangles.

For any $p'\in S'$ define $d(x_i',p')$ in the following way:

• If $\overline{x_i'p'}\subset P'$, then $d(p',x_i'):=|p'x_i'|$

• If $\overline{x_i'p'}\not\subset P'$, then $d(p',x_i'):=$ minimum of $|v'p'|+|v'x_i'|$ for $v'$ which varies between the vertexes of $S'\cap T_i$

I'm wondering if it's always possible to find $p'\in S'$ such that $d(p',x_i')\le |px_i|$ for $i=1,2,3$.

So my question is of course how to prove the existence of $p'$. I'm trying to consider all possible cases in which the vertexes of $P$ could move (given the bonds of the lengths), but it's quite complicated. Do you thing there's a better way to proceed?

Answer 1

Let me prove a bit more general statement.

Let $P=[v_1\dots v_n]$ and $P'=[v_1'\dots v_n']$ be two solid polygons such that if $[vw]$ is a side of $P$ or a diagonal which lies in $P$ completely then $|v-w|\ge |v'-w'|$. Then there is a short map $P\to P'$ which sends any vertex $v$ of $P$ to the corresponding vertex $v'$ of $P'$.

Note that if there is one diagonal $[vw]$ such that $|v-w|=|v'-w'|$ then we can cut $P$ in two polygons and reduce the question to a simpler case.

First note that we can assume that $|v-w|=|v'-w'|$ for any side of $[vw]$ of $P$. If this is note the case choose a vertex $x'$ so that the triangle $[x'v'w']$ lies in $P'$. Put joints in these vertexes and increase $|v'-w'|$ up to $|v-w|$ so that the rest of diagonals stay the same or increase. The new poygon $P''$ admits a short map to $P'$ so we can make each side to be the same as in $P$ one by one and reduce the question to this case.

Note that the convex angles get smaller in $P'$. Therefore one concave angle, say $a$, should go up, in particular it stays concave. Choose 4 vertexes: $a$, its neighbors $x$ and $y$ and a vertex $b$ visible from $a$. Put joints in these vertexes. We can move the polygon straighting the angle at $a$, keeping the distances $|a-x|$, $|a-y|$, $|b-x|$ and $|b-y|$ and nondecreasing the rest of diagonals. Repeating if necessary, we will get one more diagonal fixed.

P.S. Doing these operations, you can end in a generalized solid polygon --- a flat disc with polygonal boundary, when it is mapped to the plane you can get overlaps.

Answer 2

This was a counterexample under one interpretation of the question, where distance was Euclidean straight-line distance. Now that the OP has defined a new distance measure in cases where the line-of-sight segments are not inside $P'$, I would have to rethink this...