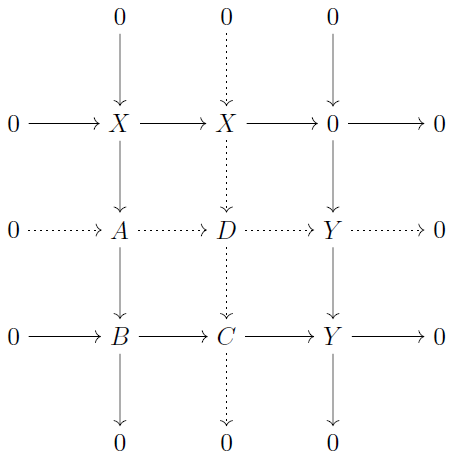
Let $R$ be a commutative ring. Suppose $R$-modules $X,A,B,C$ and $Y$ are given such that the outer two rows and the outer two columns in the following diagram are exact.
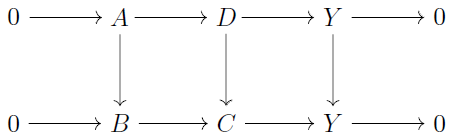
Does it follow that there exists an $R$-module $D$ such that the augmented diagram commutes and the dotted row and column are exact? Or are there counterexamples?
Such a $D$ would provide a simultaneous extension of $C$ by $X$ and of $Y$ by $A$.
This is easily seen to be true if either the left column splits (in which case we may take $D=C\oplus X$) or if the bottom row splits (in which case we may take $D=A\oplus Y$). So the answer is positive if $R$ is a field, for instance. I'm not sure how to prove the general case, neither have I been able to produce a counterexample.
Any idea or reference would be welcome.
Edit: In light of Jason Starr's comment providing a counterexample over $R=\mathbb Z[t]$, I would also be interested in sufficient conditions on $R$ such that the claim is true. For instance:
Does the claim hold if $R$ is a PID? Hereditary?