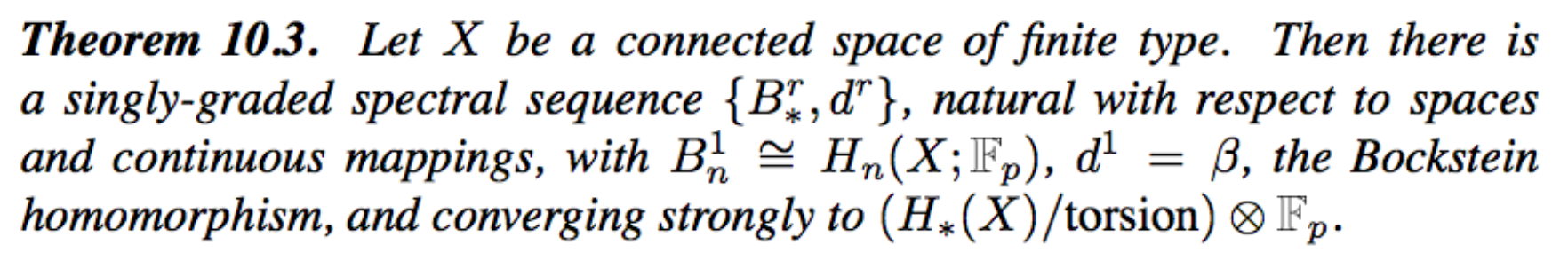I find the following theorem for Bockstein spectral sequence at http://pages.vassar.edu/mccleary/files/2011/04/MC10.fin_.pdf, page 459:
Question. for a fixed $k$, if $\beta$ does not hit $H_k(X;\mathbb{F}_p)$ hence $B^1_k$ survives at the second page, can we conclude that $B^1_k$ survives at the infinity page thus $B^1_k$ is a summand of $(H_*(X;\mathbb{Z})/Torsion)\otimes \mathbb{F}_p$?
No, you can't necessarily make this conclusion. Your spectral sequence is obtained from another doubly-graded Bockstein spectral sequence of the form $$ E^1_{r,s} = \begin{cases} H_{r+s}(X;\Bbb F_p) &\text{if }s+t \geq 0, s \geq 0\\ 0 &\text{otherwise} \end{cases} $$ with $d_1$ differential the Bockstein and $E^\infty$-page the associated graded of $H_*(X;\Bbb Z_p)$ by the multiples-of-$p$ filtration. (You can think of the $E^1$-page as $H_*(X;\Bbb F_p) \otimes \Bbb F_p[v]$, where $v$ is a polynomial generator in bidegree $(r,s) = (-1,1)$ representing multiplication-by-$p$.)
In this spectral sequence (with the assumption that $X$ has finite type), you get a $d^k$-differential if and only if there was a summand of the form $\Bbb Z/p^k$ in $H_*(X;\Bbb Z)$; this becomes a summand $\Bbb F_p[v]/v^k$ in the $E_\infty$ page. You basically get your spectral sequence by inverting $v$, and so the same holds there: there is a $d^k$-differential if and only if the original space had a $\Bbb Z/p^k$-summand in its homology.
