10
$\begingroup$

As the title suggests, I'm trying to apply the Adams Spectral sequence to get some insights of the bordism group $$ \Omega_4(\xi)= \pi_4(M\xi)$$ where $\xi \colon BSpin \times K(D_{2n},1) \to BSO$ is a stable vector bundle. I'm trying to use ASS because after an application of the James Spectral Sequence (kind of twisted AHSS) I was able to conclude that $$ \Omega_4(\xi)= \mathbb{Z} \ \text{ or } \mathbb{Z}\oplus \mathbb{Z}_2$$ Here you can find a little bit of context and some description of my previous attempts.

My idea is that a computation of $_{(2)}{\pi_4(M\xi)}$ should give me the right choice for $\Omega_4(\xi)$, therefore I try to run an ASS. My lack of expertise in this field lead me to ask a question about how to start the ASS, since I think it's easier to study the ASS with a clear problem in mind, otherwise I wouldn't understand the importance of a lot of technical lemmas done in many of the books covering it.

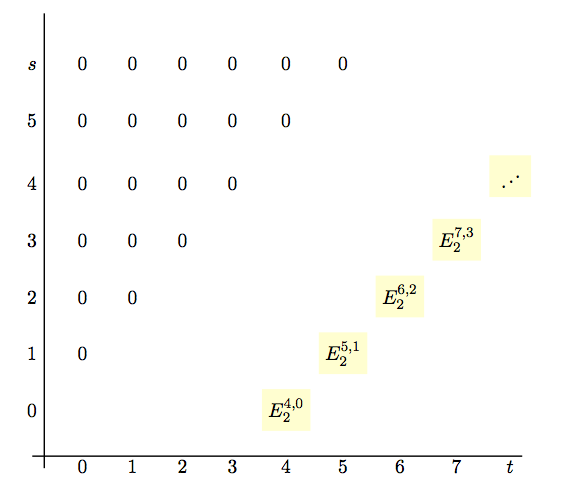
So the ASS I'm interested in should look like this $$E^{s,t}_2\cong Ext_{\mathcal{A}_2}^{s,t}(H^*(M\xi;\mathbb{Z}_2); \mathbb{Z}_2)$$ enter image description here

where the yellow diagonal is the one I'm interested in. A first glance to it lead me to this question:

(1) How can I conclude something if the diagonal contains infinitely many non-zero stable terms?

Even computing the $2$-page is troublesome. According to what I know (I've read the chapter about ASS in Fomenko-Fuchs Homotopical Topology), I should find a (minimal)-projective resolution of the the $\mathcal{A}_2$-module $H^*(M\xi;\mathbb{Z}_2)$ which via Thom iso I think can be seen as $H^*(BSpin ;\mathbb{Z}_2)\otimes H^*(D_{2n};\mathbb{Z}_2)$. Problem is that I'm supposed to find an infinitely long (minimal) resolution $B_{\bullet}\to H^*(M\xi)$, since for example $E^{s,t}_2=\hom_{\mathcal{A}_2}(B_s,\mathbb{Z}_2)$ so I really need to compute $B_s$ for all $s$ (and for every internal grade $t$).

(2) How can I (cleverly) compute at least the second page of this ASS and conclude something out of it?

The reason I'm asking these questions is that (as you can see in the linked question) I was suggested to use ASS, so I believe something could be said in this case and I'm really interested in learning how to use this powerful tool. The big amount of algebra (at least for a non-algebrist grad student like me) involved in the ASS is making difficult to me getting used to such a tool.

I'm aware that there are plenty of material about the ASS in the books and online, but I prefer learning them by getting my hands dirty with concrete examples I'm really interested in computing, otherwise I fear I will get lost in the ocean of literature about ASS

Improve this question
$\endgroup$
12
  • 2
    $\begingroup$ Note that the Thom iso between $H^*(M\xi;\mathbb Z_2)$ and $H^*(BSpin;\mathbb Z_2) \otimes H^*(D_{2n};\mathbb Z_2)$ is not an iso of $\mathcal A_2$-modules if you use the standard module structures, you need to twist the latter since the $\mathcal A_2$-action on the Thom class is non-trivial. $\endgroup$
    – user95545
    Commented Jan 18, 2017 at 15:10
  • $\begingroup$ Dear @user95545 yes I'm aware of that, but thanks for stressing it out. Interestingly enough, it shares the same twisting of the James SS when you want to compare it with the AHSS $\endgroup$
    – Riccardo
    Commented Jan 18, 2017 at 15:11
  • 2
    $\begingroup$ Another remark simplifying the computation: If I remember correctly, you said that there is a vector bundle $V$ over $D_{2n}$ such that $H^*(M\xi)\cong H^*(MSpin)\otimes H^*(TV)$. Then one possible computation uses that $MSpin\cong ko$ in low degrees, $H^*(ko)\cong \mathcal A//\mathcal A(1)$, so that one can use a change of rings isomorphism and needs to compute $Ext^{s,t}_{\mathcal A(1)} (H^*(TV);\mathbb Z_2)$. $\endgroup$
    – user95545
    Commented Jan 18, 2017 at 16:12
  • 1
    $\begingroup$ Those computations over $\mathcal{A}(1)$ are totally doable. Look at Chapter 2 of the Adams spectral sequence Primer on Bruner's website. $\endgroup$
    – Sean Tilson
    Commented Jan 30, 2017 at 9:24
  • 1
    $\begingroup$ Also, the grading convention in the diagram you drew is not what is used by anyone who works with the Adams spectral sequence. I only mention this to make you aware as this could lead to confusion. $\endgroup$
    – Sean Tilson
    Commented Jan 30, 2017 at 9:25

1 Answer 1

Reset to default
6
$\begingroup$

You should look at Hatcher's notes on spectral sequences, and if you're fluent enough in German, I can recommend §§5-6 of: Stolz, Hochzusammenhängende Mannigfaltigkeiten und ihre Ränder. With an English introduction. Lecture Notes in Mathematics, 1116. Springer-Verlag.

The upshot is that since you are interested only in low dimensions, you need to construct the resolution only in low dimensions.

Improve this answer
$\endgroup$
5
  • $\begingroup$ Thanks for your answer. I don't quite understand your sentence: "The upshot is that since you are interested only in low dimensions, you need to construct the resolution only in low dimensions.". I mean, the element in the yellow diagonal (which should be the one I'm interested in) require higher and higher steps of he resolution to be computed explicitly. Or am I missing something here? $\endgroup$
    – Riccardo
    Commented Jan 18, 2017 at 15:24
  • 1
    $\begingroup$ If you use a minimal resolution, then there will be no relations involving terms of the form "an element of degree 0 of $\mathcal A_2$ times a generator of the resolution", so the degree of the generators "goes up by at least 1". In degree 1, there is only $Sq^1$ in $\mathcal A_2$, and Stolz describes how to handle this, so that the degree of the generators "goes up by at least 2" which means that the computation will be finite. $\endgroup$
    – user95545
    Commented Jan 18, 2017 at 16:04
  • $\begingroup$ Thanks a lot, I will meditate on this last comment a little bit, since I need a little bit of time to really understand the notions involved in it! $\endgroup$
    – Riccardo
    Commented Jan 18, 2017 at 16:10
  • 1
    $\begingroup$ Let me just add what Stolz says in the introduction: "In §6 we develop a method suitable for the computation of Ext-groups including their multiplicative structure, which may be of independent interest: Given a module M over A which is bounded from below and locally finite, one can construct a 'minimal, almost free resolution up to dimension d' in a finite number of steps . From such a resolution the groups Ext^s,t(M) including the multiplicative structure can be read off in the range t-s<d. Observe that there might be infinite many pairs (s,t) with t-s<d and Ext^1,t(M) non-trivial." $\endgroup$
    – user95545
    Commented Jan 18, 2017 at 16:16
  • $\begingroup$ dear @user95545, even though I'm not so fluent in german, I read the chapters you mentioned in Stolz's and I've a few questions about that. 1) can we use an almost-free resolution for computing the $Ext$-term? 2) in 6.18 Stolz builds the resolution, since $M_s$ is $(2s-1)$-connected, the yellow diagonal $(t-s=4)$ is non-zero up to $t=8$ right? (and this is the reason why you claimed that my diagonal as finitely many non-zero terms right?) $\endgroup$
    – Riccardo
    Commented Jan 19, 2017 at 17:23

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .