0
$\begingroup$

Suppose that we have a group $G$ acting on the spaces $E$ and $B$. Suppose moreover that we have fibre bundles $\xi$ and $\eta$ in the following commutative diagram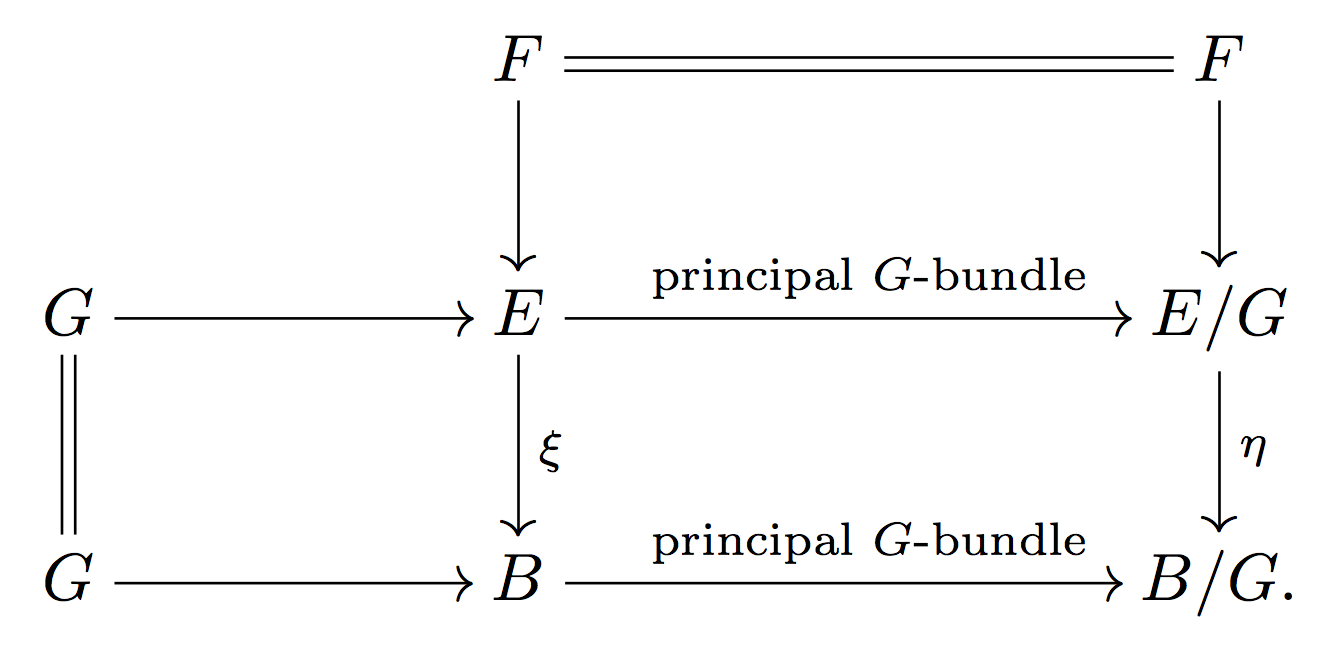enter image description here

If $\xi$ is a trivial bundle, i.e. the Cartesian product, whether could we conclude that $\eta$ is trivial as well?

Improve this question
$\endgroup$

1 Answer 1

Reset to default
6
$\begingroup$

No. let $F$ have a $G$-action, take $B=EG$ and $E=EG \times F$ with the diagonal action. Then $\eta$ is the Borel construction $EG \times_G F \to BG$ so need not be trivial.

Improve this answer
$\endgroup$

You must log in to answer this question.

Not the answer you're looking for? Browse other questions tagged .