Contrary to your claim, the overlapping number $N$ can exceed the kissing number $K$. Moreover, there exists some fixed value of $K$ for which $N$ can be arbitrarily large.
This is a sketch of a proof, which I haven't attempted to make rigorous because it would be incredibly tedious to do so.
We shall define an $N$-nematode to be a compact subset defined in polar coordinates by:
$$ \varepsilon \leq r \leq 1 $$
$$ f(r) \leq \theta \leq f(r) + \dfrac{2 \pi}{N}$$
where $f$ is a smooth function, and $\varepsilon > 0$ is small.
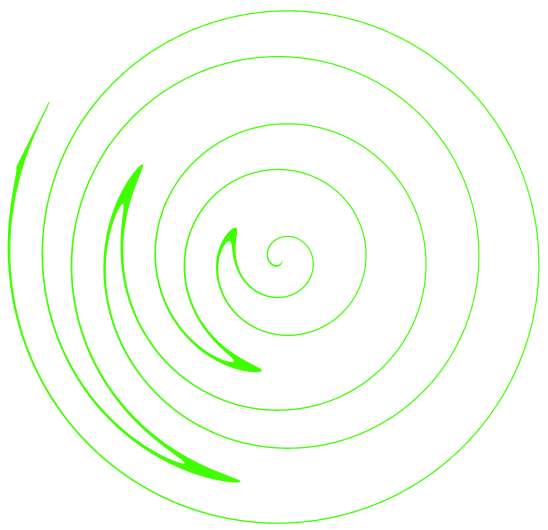
(It has a small ball of radius $\varepsilon$ removed from the centre, which you can't see in the diagram because $\varepsilon$ is microscopic.)
Just to check that you've understood my description, an $N$-nematode has area $\pi (1 - \varepsilon^2) / N$.
Clearly, $N$ $N$-nematodes can coexist in a 'family', where each nematode touches two others, and their non-overlapping union is the entire annulus. This time, I've made the $\varepsilon$-hole visible:
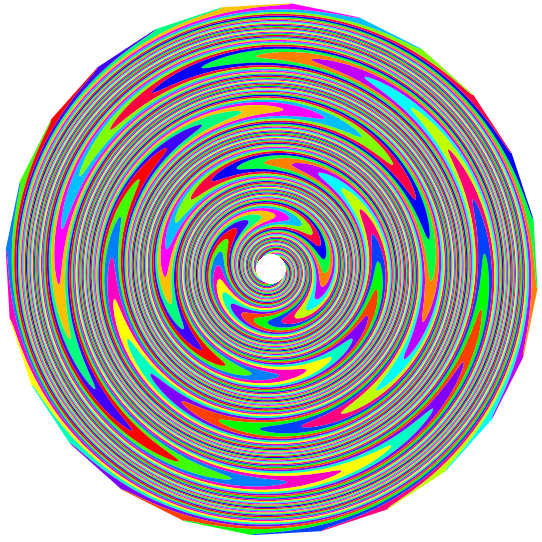
Now, provided we make the nematodes really pathological (for example, if the function $f$ violently oscillates sinusoidally between $\pm \Omega^7$ a total of $\Omega^{13}$ times, where $\Omega$ is the reciprocal of $\varepsilon$), then a nice property emerges. Specifically, the centres of any two non-overlapping nematodes must be either distant (greater than $2 - \varphi$) or incredibly close (within $\varphi$ of each other), where $\varphi \ll \dfrac{\varepsilon}{N}$. Basically, nematodes are either disjoint in the way that discs are, or are almost concentric.
So in any arrangement of non-overlapping nematodes, the nematodes cluster into sets of cardinalities $\leq N$. Now, for a given nematode $\nu$, we can easily bound the following quantities:
- The number of nematodes in the same cluster as $\nu$ which touch $\nu$ (bounded above by $2$);
- The number of different clusters, members of whom can be touched by $\nu$ (bounded above by the maximum number of points that fit into a radius-$(2 + 2 \varphi)$ disc such that no two are within a distance of $2 - \varphi$, which is certainly $7$);
- The number of nematodes within another cluster that can touch $\nu$ (certainly $3$ will suffice, by some sophisticated argument concerning the convex hulls of clusters).
This gives a universal bound (independent of $N$) on the kissing number $K$ as $2 + 7 \times 3 = 23$. And we can easily get an overlapping number of $N$ by arbitrarily and effortlessly dropping a nematode $\nu$ onto a family of $N$ nematodes, where the distance between the centre of $\nu$ and the centre of the family is $1$.
The result follows.