3
$\begingroup$

Assume we have a $3\times 3$ grid with rows and columns being short exact sequences of $C^*$-algebras.\xymatrix{&0\ar[d]&0\ar[d]&0\ar[d]&\0 \ar[r] & A_{00} \ar[r]^{\varphi_{00}}\ar[d]^{\psi_{00}} & A_{01} \ar[r]^{\varphi_{01}}\ar[d]^{\psi_{01}} & A_{02} \ar[r]\ar[d]^{\psi_{02}} & 0\0 \ar[r] & A_{10} \ar[r]^{\varphi_{10}}\ar[d]^{\psi_{10}} & A_{11} \ar[r]^{\varphi_{11}}\ar[d]^{\psi_{11}} & A_{12} \ar[r]\ar[d]^{\psi_{12}} & 0\0 \ar[r] & A_{20} \ar[r]^{\varphi_{20}}\ar[d] & A_{21} \ar[r]^{\varphi_{21}}\ar[d] & A_{22} \ar[r]\ar[d] & 0\&0&0&0&}

This gives a grid of 6-term exact sequences: 3 "horizontal" sequences and 3 "vertical" sequences, forming a torus-like diagram with 18 groups and 36 maps. Commutativity of 16 out of 18 squares follows from naturality of functors $K_0$, $K_1$ the index map $\delta_1$ and exponential map $\delta_0$. Two squares remain, and it seems, that they are "anti-commutative", but I didn't manage to prove this. These squares are the following. 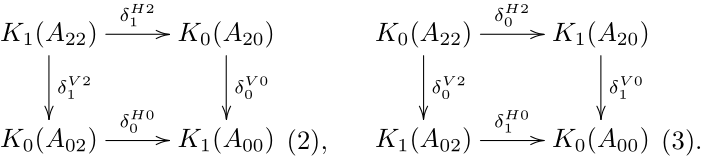2 squares from K-theory diagram

My conjecture is that both squares are anti-commutative, i.e.

  1. $\delta_0^{V0}\delta_1^{H2}(g) = -\delta_0^{H0}\delta_1^{V2}(g)$ for any $g\in K_1(A_{22})$,
  2. $\delta_1^{V0}\delta_0^{H2}(g) = -\delta_1^{H0}\delta_0^{V2}(g)$ for any $g\in K_0(A_{22})$.

One follows from the other by replacing $A_i$ with its suspension $SA_i$.

Question: Is it possible to prove the anti-commutativity of squares (2) and (3) in general?

P.S. The notation follows the book "An introduction to $K$-theory for $C^*$-algebras" by Rordam, Larsen and Laustsen. Upper index of $\delta_i$ describes the exact sequence from (1) it corresponds to. E.g. $H2$ refers to the bottom (#2) horizontal sequence on diagram (1).

Improve this question
$\endgroup$
1
  • $\begingroup$ It also folows from the fact that $K$ sends short exact sequences of $C^*$-algebras to exact triangles of spectra, and then from general properties of triangulated categories. $\endgroup$
    – Fernando Muro
    Commented Nov 26, 2014 at 9:55

1 Answer 1

Reset to default
1
$\begingroup$

This is true. It follows essentially from the naturality of the boundary map with respect to morphisms of extensions. We need to show that the composition of the two boundary maps $$ K_*(A_{00})\to K_{1-*}(A_{02}\oplus A_{02})\to K_*(A_{22}) $$ vanishes. But the first map factors as a composition of the boundary map $K_*(A_{00})\to K_{1-*}(A_{01}+A_{01})$ and the restriction map $K_{1-*}(A_{01}+A_{01})\to K_{1-*}(A_{02}\oplus A_{02})$ induced by the quotient map $$A_{01}+A_{01}\to\dfrac{A_{01}+A_{01}}{A_{00}}\cong A_{02}\oplus A_{02}.$$ On the other hand, the composition of the restriction map $K_{1-*}(A_{01}+A_{01})\to K_{1-*}(A_{02}\oplus A_{02})$ with the second boundary map $K_{1-*}(A_{02}\oplus A_{02})\to K_*(A_{22})$ must be zero because these are two consecutive maps in a six-term exact sequence.

Improve this answer
$\endgroup$

You must log in to answer this question.