Distance from constant width bodies

Question

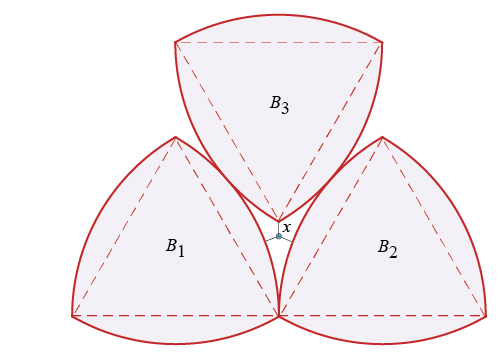
EDIT As @David has observed, my conjecture was clearly wrong for $\ n:=2.\ $ Let me still give it a chance for $\ n\ge 3$.

I'll call a family $\ F\ $ of bound closed convex subsets of $\ \mathbb R^n\ $ to be impressive $\ \Leftarrow:\Rightarrow\ $ each set $\ B\in F\ $ has constant width greater or equal $1$, and each two different members of $\ F\ $ have disjoint interiors. Family $\ F\ $ is called assuming $\ \Leftarrow:\Rightarrow\ \ F\ $ is impressive and the width of each $\ B\in F\ $ is exactly $1\ $ (so that all diameters are $1$ under the given circumstances).

The following conjecture seems obvious but I don't have a proof:

CONJECTURE   Let $\ n>2.\ $ There exists real $\ \delta_n > 0\ $ such that for every impressive $(n+1)$-element family $\ F\ $ in $\ \mathbb R^n,\ $ and every $\ x\in\mathbb R^n,\ $ the following inequality holds:

$$\max_{B\in F} d(x\ B)\ \ge\ \delta_n$$

where $\ d(x\ B) := \inf_{y\in B}\, ||x-y||\ $ (the Euclidean norm is meant).

The harder challenge seem to be the exact computation of the maximal possible $\ \delta_n.\ $ Now let's still call this maximal constant simply $\ \delta_n.\ $ Furthermore, I'd like to know also a similar constant $\gamma_n\ $ restricted to the assuming families, i.e. $\ \gamma_n\ $ is the maximal constant such that for every $(n+1)$-element assuming family $\ F,\ $ and for every $\ x\in\mathbb R^n,\ $the following inequality holds:

$$\max_{B\in F} d(x\ B)\ \ge\ \gamma_n$$

Obviously, $\ \, \delta_n\, \le\ \gamma_n$.

Needless to say, I apologize if this problem is well-known.

Answer 1

Not an answer; just an illustration. I had some difficulty understanding the question, so...

Here $n=2$, so the shapes are planar, $\mathbb{R}^2$. I used Reuleaux triangles for the $3=n{+}1$ unit-constant-width bodies $F=\{ B_1, B_2, B_3 \}$ forming an "impressive" and "assuming" family $F$. A particular point $x \in \mathbb{R}^2$ is shown, with segments achieving $d(x,B_i)$. In this case, all three of those min-distances to the bodies are equal, so that is also the max $\gamma_2$.

---

 

---

So I think the question is simply asking if there is a lowerbound on the radius of a ball that can nestle in the gap.? I.e., can we ensure that the gap is not arbitrarily small?

Apologies if I am misinterpreting...

Added: To address $d{=}3$ & Per A.'s question, here is an image (from here) of a constant-width Meissner tetrahedron:

---