Not an answer; just an illustration. I had some difficulty understanding the question, so...
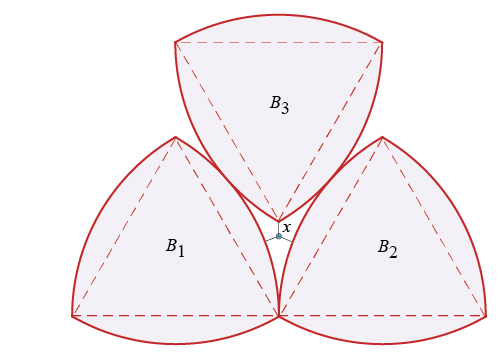
Here $n=2$, so the shapes are planar, $\mathbb{R}^2$. I used Reuleaux triangles for the $3=n{+}1$ unit-constant-width bodies $F=\{ B_1, B_2, B_3 \}$ forming an "impressive" and "assuming" family $F$. A particular point $x \in \mathbb{R}^2$ is shown, with segments achieving $d(x,B_i)$. In this case, all three of those min-distances to the bodies are equal, so that is also the max $\gamma_2$.
![Reuleaux][1]
So I think the question is simply asking if there is a lowerbound on the radius of a ball that can nestle in the gap.? I.e., can we ensure that the gap is not arbitrarily small?
So I think the question is simply asking if there is a lowerbound on the radius of a ball that can nestle in the gap.? I.e., can we ensure that the gap is not arbitrarily small?
Apologies if I am misinterpreting...
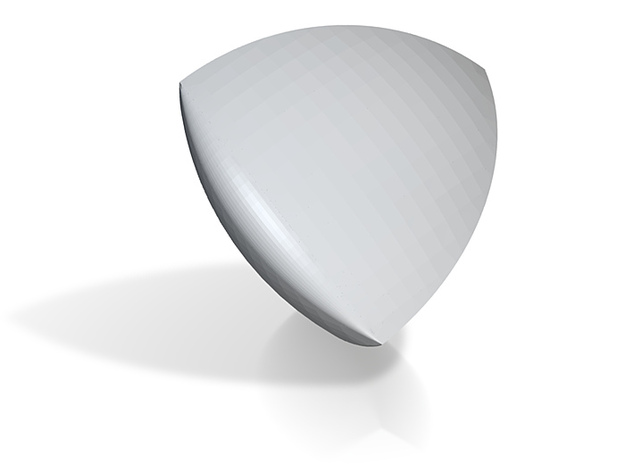
Added: To address $d{=}3$ & Per A.'s question, here is an image (from herehere) of a constant-width Meissner tetrahedronMeissner tetrahedron:
![CWidth3D][2]