Colourings of $\mathbb Q\times \mathbb Q$ in three colours

Question

Using two-adic valuation Monsky coloured $\mathbb Q\times \mathbb Q$ in red, blue, and green, so that on each line points of at most two colours are present.

Question. I would like to know if there is some kind of classification of three-colourings with this property, or some structure theorem about them. How rare are such colouring? (I would like to exclude "degenerate" colourings that use almost only two colours)

The colouring of Monsky is as follows: $(x,y)$ is coloured

blue if $|x|_2\ge |y|_2,\, |x|_2 \ge 1$,

green if $|x|_2< |y|_2,\, |y|_2 \ge1$,

red if $|x|_2< 1,\, |y|_2 < 1$.

Answer 1

Here are, at least, some remarks about your question that will not fit as a comment:

You request parenthetically that you would like to exclude "degenerate" colourings that use almost only two colours. Let us make this notion more precise.

In particular, I refer to the paper:

Hales, A., & Straus, E. (1982). Projective colorings. Pacific Journal of Mathematics, 99(1), 31-43.

First, we disallow colorings that use only two colors.

Second, we consider your notion of "almost only two colours" as being a trivial coloring, defined as being one of the following two types of colorings (up to the appropriate interchange of colors):

1. Color a single point, $p$, red. Color every line through $p$ (with $p$ excluded) either only green or only blue, randomly.

2. Color the points on a line, $\ell$, either green or blue, randomly; color all points not on $\ell$ red.

Denote by $\mathbf{P}_{2}(F)$ the projective plane over a commutative field $F$. Hales and Straus prove the following more general theorem:

Example: From the theorem above, we see that $\mathbf{P}_{2}(\mathbb{Q})$ has a nontrivial $3$-coloring, since the field $\mathbb{Q}$ admits the nontrivial non-Archimedean valuation $|\cdot|_2$.

The construction for the proof of Theorem 1 is essentially the same as that of Monsky (mentioned in a foot-note as: We recently became aware that this coloring was previously introduced in [Monsky] for the affine plane). Here is one direction of the iff statement from the paper cited above:

An important note is that Monsky's original paper was entitled On Dividing a Square into Triangles. This remark is relevant as marked in red below (p. 41 in Hales & Straus):

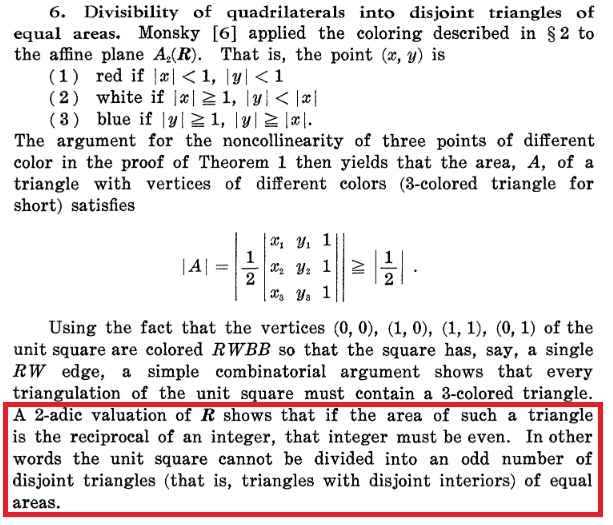
This is the reason that Monsky uses the $2$-adic valuation. As to your question (at least the projective version): There should be no reason why you could not, as in the proof of Theorem 1, begin by choosing any nontrivial non-Archimedean valuation on $\mathbb{Q}$. You may not be proving anything about squares divided into disjoint triangles, but you will still be able to attain a nontrivial $3$-coloring of $\mathbf{P}_{2}(\mathbb{Q})$. (See Hales & Straus' Theorem 7 for ramifications of using a $p$-adic valuation when $p > 2$.)

As a final remark, of which you are likely aware, one might wonder what nontrivial non-Archimedean valuations exist on $\mathbb{Q}$. The answer is essentially the $p$-adic valuations for primes $p$; this result is implied by Ostrowski's Theorem (cf. e.g., Koblitz's p-adic Numbers, p-adic Analysis, and Zeta-Functions).