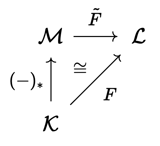Prof and the completion of Cat under right adjoints

Question

In Bénabou's Les distributeurs, in which the bicategory of profunctors is introduced, Bénabou remarks (page 17, quoted below) that $\mathbf{Prof}$ may be viewed as the construction of a bicategory from $\mathbf{Cat}$ by freely adjoining adjoints to functors. (Indeed, for every functor $F : A \to B$ between small categories, we have profunctors $F_* : A \nrightarrow B$ and $F^* : B \nrightarrow A$ given by $F_*(b, a) = B(b, Fa)$ and $F^*(a, b) = B(Fa, b)$, such that $F_* \dashv F^*$.) Is $\mathbf{Prof}$ the free bicategory with this property?

More precisely, does the inclusion $\mathbf{Cat} \to \mathbf{Prof}$ exhibit $\mathbf{Prof}$ as the free bicategory for which every 1-cell in $\mathbf{Cat}$ has a right adjoint in $\mathbf{Prof}$?

If not, how close is this statement to being true?

Le but visé en construisant Dist était d'ajouter des adjoints pour tous les foncteurs (les flèches de Cat). Ce but est atteint de la façon la plus économique possible : tout distributeur peut être représenté par un couple formé d'un foncteur et du distributeur adjoint d'un autre foncteur.

Answer 1

The idea that passing from $Cat$ to $Prof$ is a great way to give functors adjoints features prominently in Richard Wood's theory of proarrow equipments. Rosebrugh and Wood showed in Proarrows and cofibrations that there is a general 2-categorical construction which takes a 2-category like $V-Cat$ for an enriching category $V$ and outputs $V-Prof$. (Incidently, they also showed that the same construction applied to the 2-category of topoi and geometric morphisms yields the 2-category of topoi and left exact functors). There's another construction which does something similar for internal categories. But neither of these constructions claim to have precisely the universal property of adjoining adjoints.

In Adjoining adjoints, Dawson, Pare, and Pronk study the 2-categorical construction which freely adjoins adjoints to 1-cells. If I remember correctly, they find that this construction does not quite turn $Cat$ into $Prof$, nor $Set$ into $Span$. But I think they formulate a base-change condition to add to the universal property which comes closer. More recently Yanovski and Horev have studied a similar construction in $\infty$-categories.

I apologize for being too lazy to look through the papers I'm linking to and fish out the actual answer!

I should also mention that the same authors showed that freely adjoining adjoints is in general undecidable in undecidability of free adjoints. I'm not a computability person, so I don't know have a good handle on the "computability of the input" assumptions going into this. In general this construction does seem to be ill-behaved (beyond cases like the free adjunction on a 1-morphism). I thought I'd read about getting better behavior by imposing some additional compatibility on the adjunction, but I can't seem to find it now. It might be related to Free extensions of double categories by the same authors.

EDIT: Aha! In The span construction, the same authors give a universal property of spans in terms of companions and conjoints rather than pure adjointness. I suspect similar considerations might be relevant to $Cat$ and $Prof$.

Answer 2

I discovered a related characterisation in Betti's Formal theory of internal categories. For $\mathcal E$ a finitely complete category, Betti claims (in the theorem at the top of page 49) that $\mathbf{Prof}(\mathcal E)$ is the smallest bicategory containing $\mathbf{Cat}(\mathcal E)$ closed under right extensions. He attributes this result to Bénabou's Les distributeurs, though I can see no such result there. The proof is sketched for the action on 1-cells, but not 2-cells.

Answer 3

I shall sketch out a proof that $\mathbf{Prof}$ is almost obtained from $\mathbf{Cat}$ by adjoining right adjoints to every 1-cell, following Roald Koudenburg's suggestions in the comments. The remaining question is which isomorphisms of 1-cells hold in $\mathbf{Prof}$ that do not hold for purely formal reasons.

---

Edit: the following is not quite true, because there may be additional isomorphisms between 2-cells of $\mathcal M$ that do not hold for purely formal reasons, e.g. Beck–Chevalley conditions.

Proposition. Let $({-})_* \colon \mathcal K \to \mathcal M$ be a proarrow equipment in which every 1-cell $P \colon X \not\to Y$ in $\mathcal M$ factors (not necessarily uniquely) as a representable followed by a corepresentable, and as a corepresentable followed by a representable, i.e. $P \cong {p_1}_* ; {p_2}^*$ and $P \cong {p_3}^* ; {p_4}_*$ for $p_1, p_2, p_3, p_4$ in $\mathcal K$. Then $({-})_*$ exhibits $\mathcal M$ as the free adjunction of right adjoints to 1-cells in $\mathcal K$, in the sense that, for any pseudofunctor $F \colon \mathcal K \to \mathcal L$ for which each 1-cell in the image of $F$ has a right adjoint, there is an essentially unique pseudofunctor $\tilde F \colon \mathcal M \to \mathcal L$ making the following diagram commute up to pseudonatural isomorphism. (Note that $\tilde F$, being a pseudofunctor, necessarily preserves adjunctions.)

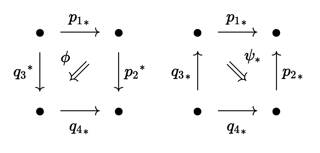
Proof. We shall show that any pseudofunctor $L \colon \mathcal M \to \mathcal L$ is determined by its action on representables, by which the result will follow. Since $({-})_*$ is the identity-on-objects, the action of $L$ on 0-cells is trivially determined by the action on representable 0-cells, since every 0-cell is representable. For any proarrow $P$, we have $L(P) \cong L({p_1}_* ; {p_2}^*) \cong L({p_1}_*) ; L({p_2}^*)$, hence determined by the action of $L$ on representables and corepresentables. But the action on corepresentables is determined by the action on representables, since we must have $L({p_2}^*) \cong L({p_2}_*)^*$ since ${p_2}_* \dashv {p_2}^*$ and pseudofunctors preserve adjunctions. Finally, the action of $L$ on any 2-cell $\phi \colon P \Rightarrow Q$ is determined by its action on $\phi' \colon {p_1}_* ; {p_2}^* \cong P \Rightarrow Q \cong {q_3}^* ; {q_4}_*$. By taking mates, $L(\phi')$ is uniquely determined by its action on a 2-cell ${q_3}^* ; {p_1}_* \Rightarrow {q_4}_* ; {p_2}_*$ which is necessarily representable as $({-})_*$ is locally fully faithful.

Therefore, $L$ is determined on 0-cells, 1-cells, and 2-cells by its action on representables, and hence by the pseudofunctor $L({-})_* \colon \mathcal K \to \mathcal M$. Given a pseudofunctor $F \colon \mathcal K \to \mathcal L$, there is thus an essentially unique pseudofunctor $\tilde F \colon \mathcal M \to \mathcal L$ making the diagram commute up to pseudonatural isomorphism.

---

The desired characterisation of $\mathbf{Prof}$ now follows.

---

Corollary. The proarrow equipment $({-})_* \colon \mathbf{Cat} \to \mathbf{Prof}$ exhibits $\mathbf{Prof}$ as the free adjunction of right adjoints to 1-cells in $\mathbf{Cat}$.

Proof. Every profunctor factors as a representable followed by a corepresentable using the collage construction, and as a corepresentable followed by a representable using the cocollage construction (Proposition 2.3.2 in Les distributeurs). The result then follows by applying the Proposition.