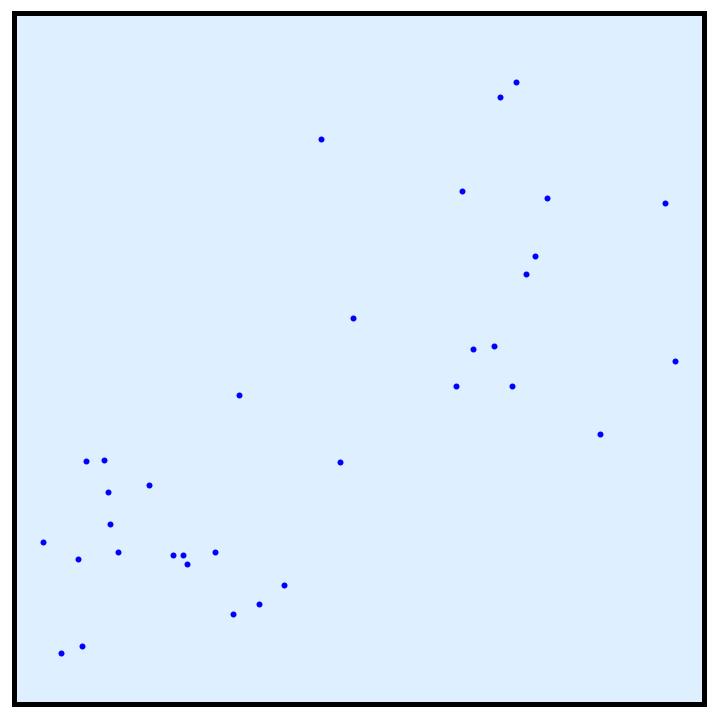
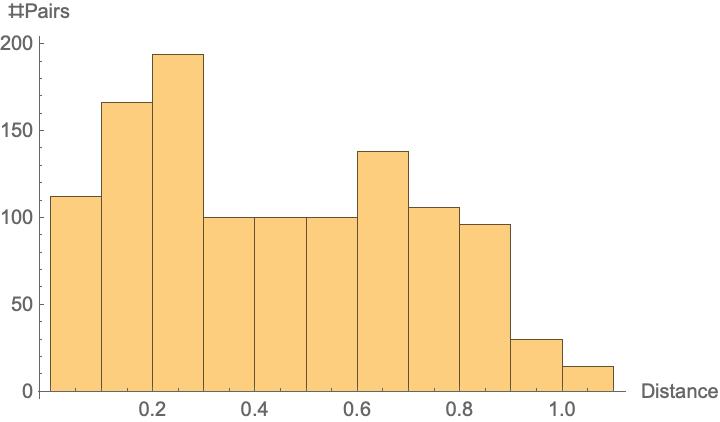
Here are three conjectures, in the hopes of showing that we can say something non-trivial.
[UPDATE: This is essentially all in a paper from Erdős, "On Sets of Distances of $n$ Points", from 1946. The conjecture on maxima is in his theorem 3, though he called that part well-known. The conjecture on minima is a later theorem of Harborth, cited here, but the bound in less precise forms is also in Erdős's theorem 3. The conjecture on modes is made after Erdős's theorem 2, in the speculation that $g(n)<n^{1+\epsilon}$, after a proof that $g(n)<n^{3/2}$. Finally Erdős's theorem 1 gives bounds on the number of distinct distances, between $(n-3/4)^{1/2}-1/2$ and $cn/(\log n)^{1/2}$.]
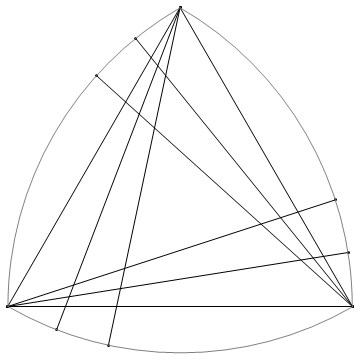
Maxima: Among $n$ points, the maximal distance can be achieved by at most $2n$ ordered pairs. This bound will be achieved when all the points are on a Reuleaux triangle, with the three 60-degree circle arcs centered on three of the points. [Thanks to Yoav Kallus for the idea for this construction.]
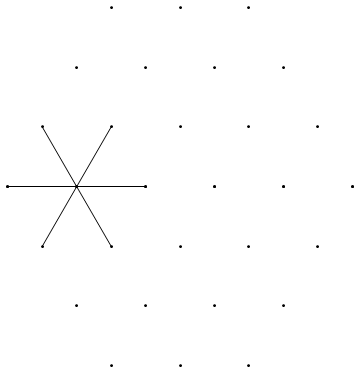
Minima: Among $n$ points, the minimal distance can be achieved by at most $6n-2\sqrt{12n-3}$ ordered pairs. This bound will be achieved when $n=3k^2-3k+1$ and the points are in a hexagonal lattice with $k$ points on each side of the hexagon.
Modes: For arbitrarily large $k$ and $n$, there are configurations of $n$ points in which the modal distance is achieved by at least $kn$ ordered pairs. For example, using square lattices:
- If $k=7$, the distance of $\sqrt{5}=\sqrt{2^2+1^2}$ can be achieved by at least $7n$, and almost $8n$ ordered pairs.
- If $k=15$, the distance of $\sqrt{65}=\sqrt{8^2+1^2}=\sqrt{7^2+4^2}$ can be achieved by at least $15n$, and almost $16n$ ordered pairs.
- If $k=23$, the distance of $\sqrt{325}=\sqrt{18^2+1^2}=\sqrt{17^2+6^2}=\sqrt{15^2+10^2}$ can be achieved by at least $23n$, and almost $24n$ ordered pairs.
But the claim would be false if we replaced $kn$ by $kn^{1+\epsilon}$.
Again, these are only conjectures; I'd be happy to see proofs or alternatively configurations where the minimal or maximal or modal distance is achieved more often.